Suppose that E1 and E2 are two events of a random experiment such that P(E1)=14,P(E2∣E2)=12andP(E1∣E2)=14. Observe the lists given below :
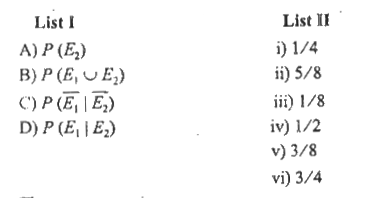
The correct matching of the list I from the list II is :Solution in Telugu
A-ii, B-iiii, C-vi, D-i
A-iv, B-v, C-vi, D-i
A-iv, B-ii , C-vi, D-i
A-I, B-ii, C-iii, D-iv
The correct Answer is:C
Step by step video, text & image solution for Suppose that E_1 and E_2 are two events of a random experiment such that P(E_(1))=1/4 , P(E_2 |E_2) =1/2 and P(E_1 |E_2)=1/4. Observe the lists given below : The correct matching of the list I from the list II is : by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - PROBABILITYEXERCISE - EXERCISE 2 (SPECIAL TYPE QUESTIONS) SET-45videos
PROBABILITY
- BOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - PROBABILITYEXERCISE - EXERCISE 2 (SPECIAL TYPE QUESTIONS) SET-28videos
PROBABILITY
- BOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - PERMUTATIONS & COMBINATIONSEXERCISE - EXERCISE 2 ( SPECIAL TYPE QUESTIONS ) SET -410videos
PERMUTATIONS & COMBINATIONS
- BOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - PRODUCTS OF VECTORSEXERCISE - Exercise 2 (Special Type Questions) SET -D14videos
PRODUCTS OF VECTORS
Similar Questions
A: There are 4 letters and 4 addressed envelopes. If the letters are put in the random in the envelopes , the probability that all the letters may be placed in wrongly addressed envelopes is 3/8.
R: If n letters are put at random in the n addressed envelopes , the probability that all the letters may be in wrong envelopes = 1-11!+12!−13!+.....+(−1)nn!07:42View Solutionthe probabilities of getting 3 heads when tossing 7 coins is
View SolutionA : the number of ways in which 5 boys and 3 girls can sit in a row so that two girls come together is 5!6P5
R : the number of ways of ways in which m ( first type of different ) things and n ( second type of different ) things (m+1≥n) can be arranged in a row so that no two things of second kind come together is m! (m+1)Pn01:41View SolutionA : Angle between the vectors I - 2j + k, 2i - j - k is π3.
R : If θ is the angle between a,b then cos θ=a.b|a||b|00:38View SolutionStatement -1 : Let E1,E2,E3 be three events such that P(E1)+P(E2)+P(E3)=1, then E1,E2,E3 are exhaustive events.
Statement-2 if the events E1,E2 and E3 be exhaustive events, then P(E1∪E2∪E3)=105:21View SolutionE1,E2 are events of a sample sapce such that P(E1) =1/4 , P(E2∣E1)=1/2,P(E1∣E2)=1/4. Then P(E1∣E2)+P(E1∣¯¯¯¯E2)=
05:51View SolutionSuppose E1 and E2 are two events of a sample space such that P(E1)=12,P(E2∣E1)=12,P(E1∣E2)=14 then P(E'2)= _____
03:23View SolutionFill in the Blanks :
If E1 and E2 are independent events, then P(E1 ∩ E2) =..01:32View SolutionStatement -1 : Let E1,E2,E3 be three events such that P(E1)+P(E2)+P(E3)=1, then E1,E2,E3 are exhaustive events.
Statement-2 if the events E1,E2 and E3 be exhaustive events, then P(E1∪E2∪E3)=101:27View SolutionSuppose that E1 and E2 are two events of a random experiment such that P(E1)=14,P(E2∣E1)=12andP(E1∣E2)=14. Observe the lists given below :
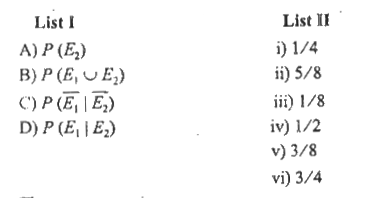
The correct matching of the list I from the list II is :01:32View SolutionIf E_1 and E_2 are two independent events associated with an experiment, then show that P(E_1cupE_2)=1-P(E_1^c)P(E_2^c)
02:15View SolutionIf E1 and E2 are independent events associated with an experiment such that P(E1)=P1 and P(E2)=P2. Find
P(E1andE2).01:15View SolutionIf E_1 and E_2 are independent events associated with an experiment such that P(E_1)=P_1 and P(E_2)=P_2 . Find P(E_1 but not E_2) .
01:15View SolutionIf E_1 and E_2 are independent events associated with an experiment such that P(E_1)=P_1 and P(E_2)=P_2 . Find P( neither E_1 nor E_2) .
01:52View SolutionIf E1 and E2 are independent events associated with an experiment such that P(E1)=P1 and P(E2)=P2. Find
P(E1orE2).02:07View Solution

