The volume charge density in a spherical ball of radius R varies with distence r from the centre as p(r)=p0[1−(rR)3], where,p0 is a constant. The radius at which the field would be maximum is Solution in Telugu
R21/3
R
R2
R1/32
The correct Answer is:A
Given, ρ(r)=ρ0[1−(rR)3]
So, effective charge at r is q ( r ) =∫ρ ( r ) .V
=ρ0[1−(rR)3]43πr3
For maximum value of field,
dq(r)dr=0
⇒43πρ0[3r2(1−r3R3)+r3(−3r2R3)]=0
⇒3r2−3r5R3−3r5R3=0
⇒r3=R32⇒r=R213
Topper's Solved these Questions
Similar Questions
A nucleus of deuterons or deuterium is a bound atomic system best described by
View SolutionThe pressure on a circular plate is measured by measuring the force on the plate and the radius of the plate. If the errors in measurement of the force and the radius are 5% and 3% respectively, the percentage of error in the measurement of pressure is
View SolutionAn infinite cylindrical wire of radius R and having current density varying with its radius r as, J=J0[1−(r/R)]. Then answer the following questions.
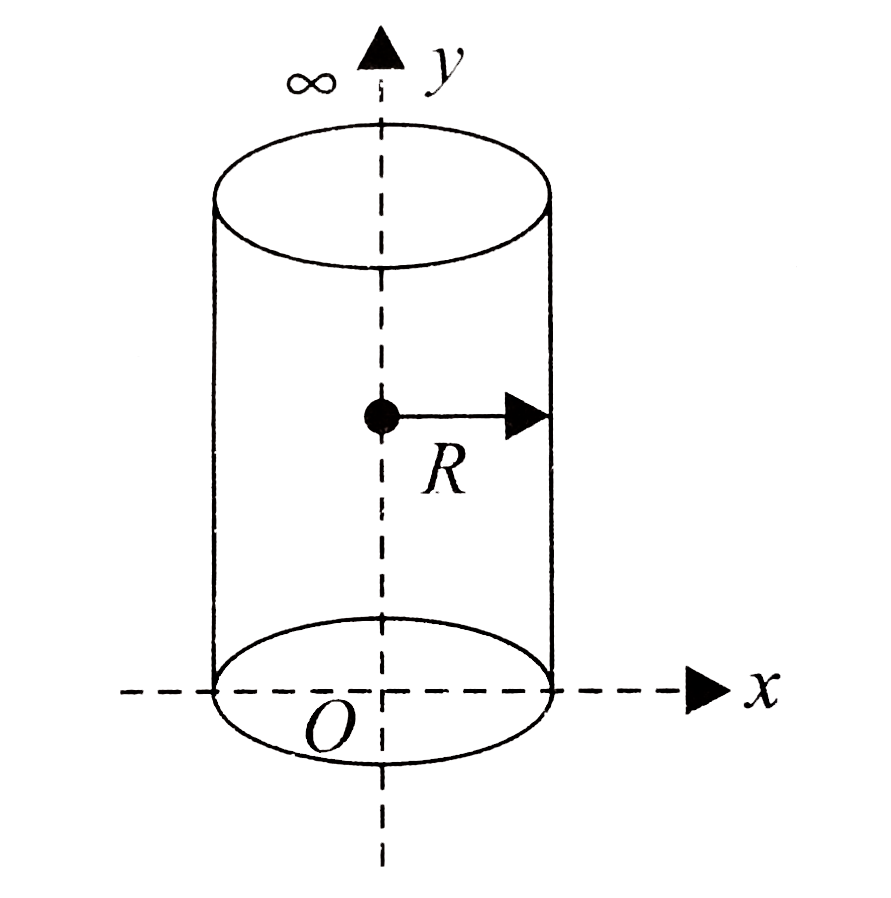
Position where magnetic field strength is maximum.
View SolutionThe earth is a homogeneous sphere of mass M and radius R. There is another spherical planet of mass M and radius R whose density changes with distance r from the centre as p=p_(0)r. (a) Find the ratio of acceleration due to gravity on the surface of the earth and that on the surface of the planet. (b) Find p_(0).
View SolutionLet p=(Qr^(3))/(piR^(5)) be the volume charge density at distance r from the centre for a a soild sphere of radius R and charge Q. The electric field at r= (R)/(2) from the centre will be
View Solutionएक गोलाकार चालक पर आवेश इस प्रकार वितरित है कि गोले के केंद्र से दूरी r पर आवेश के आयतन घनत्व p का मान निम्न है ,
p=p0(1−ra), जबकि 0<r<a तथा p=0 जबकि r>a, यहाँ P0, एक नियतांक है।
कुल वितरित आवेश की गणना कीजिए।
View SolutionConsider a spherical symmetric charge distribution with charge density varying as ρ={[ρ0(1−rR),r≤R][0,,r>R]}The electric field at r ,r≤R will be
View Solution[" Consider a spherical symmetric "],[" charge distribution with charge "],[" density varying as "],[[rho={[rho_(0)(1-(r)/(R)),r R]]
View SolutionThe volume charge density in a spherical ball of radius R varies with distance r from the centre s ρ(r)=ρ0[1−(rR)3] where ρ0 is a constant. The radius at which the field be maximum is
View SolutionA spherical region of space has a distribution of charge such that the volume charge density varies with the radial distance from the centre r as ρ=ρ0r3,0≤r≤R where ρ0 is a positive constant. The total charge Q on sphere is
View SolutionThe density of a solid sphere of radius R is P(r)=20r2R2 where, r is the distence from its centre. If the gravitational field due to this sphere at a distence 4 R from its centre is E and G is the gravitational constant, them the ratio of EGR is
View SolutionLet there be a spherical symmetric charge density varying as p(r)=p0rR upto r = R and ρ(r)=0 for r>R, where r is the distance from the origin. The electric field at on a distance r(r<R) from the origin is given by -
View SolutionThe mass density of a planet of radius R varie with the distance r form its centre as ρ(r)=p0(1−r2R2). Then the graviational field is maximum at :
View SolutionIn a region of space having a spherically symmetric distribution of charge, the electric flux enclosed by a concentric spherical Gaussian surface, varies with radius r as
ϕ=ϕ0r3R3 for r≤R
ϕ=ϕ0 for r > R
where R and ϕ0 are constants.
The volume charge density in the region is given as
View SolutionThe mass density of a planet of radius R varie with the distance r form its centre as ρ(r)=p0(1−r2R2). Then the graviational field is maximum at :
View Solution