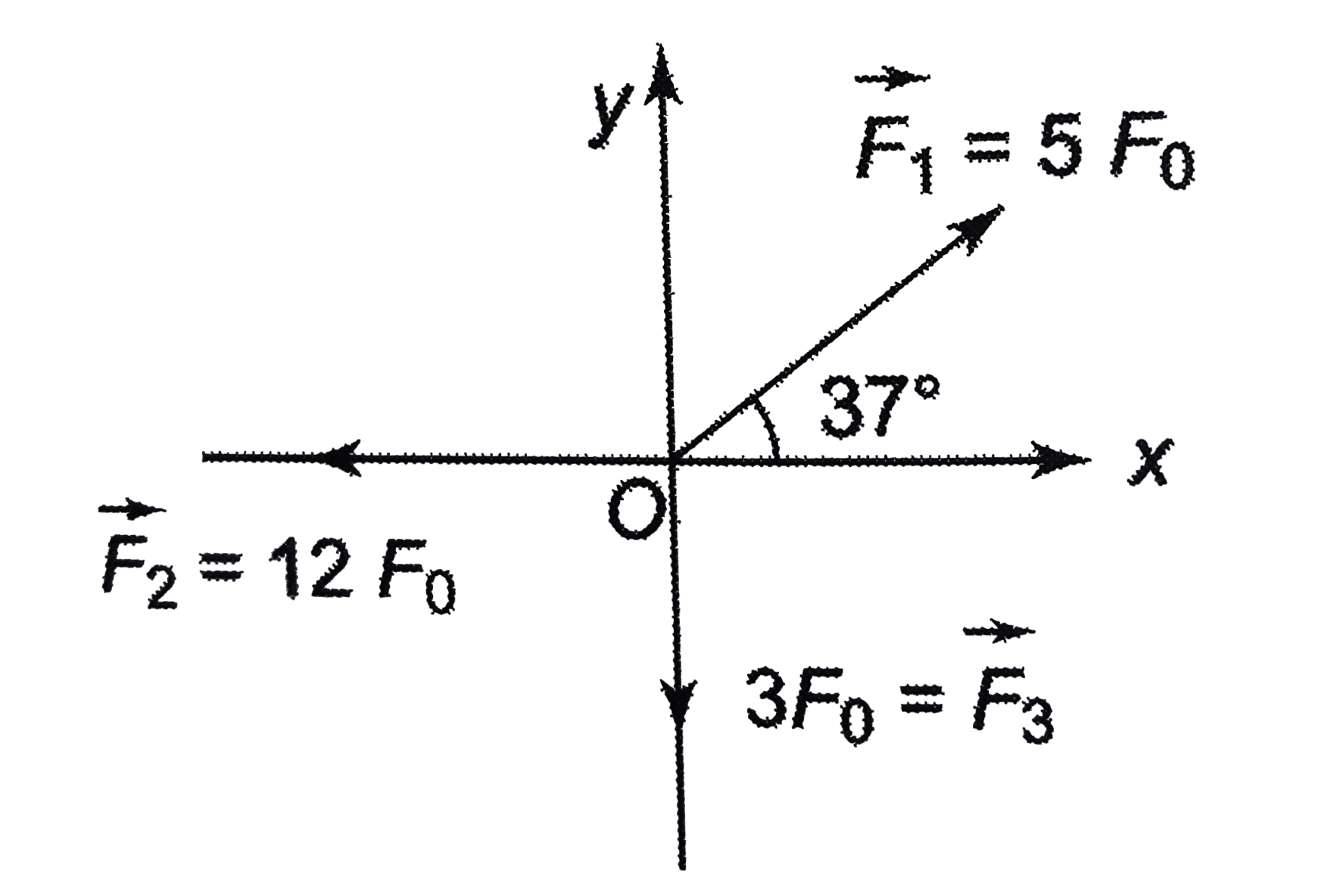
Find the resultant of the following forces.
[sin37∘=35,cos37∘=45,sin53∘=45,cos53∘=35]
![Find the resultant of the following forces. [sin 37^(@) = (3)/(5) ,cos 37^(@) = (4)/(5) , sin 53^(@) = (4)/(5) , cos 53^(@) = (3)/(5)]](https://d10lpgp6xz60nq.cloudfront.net/physics_images/CPS_V01_C03_S01_014_Q01.png)
A
The resultant of these is 10N at53∘with the positive X-axis.
B
The resultant of these is 10N at43∘with the positive X-axis.
C
The resultant of these is 100N at53∘with the positive X-axis.
D
The resultant of these is 120N at53∘with the positive X-axis.
Video Solution
Text Solution
The correct Answer is:A
Resolve the inclined vectors.
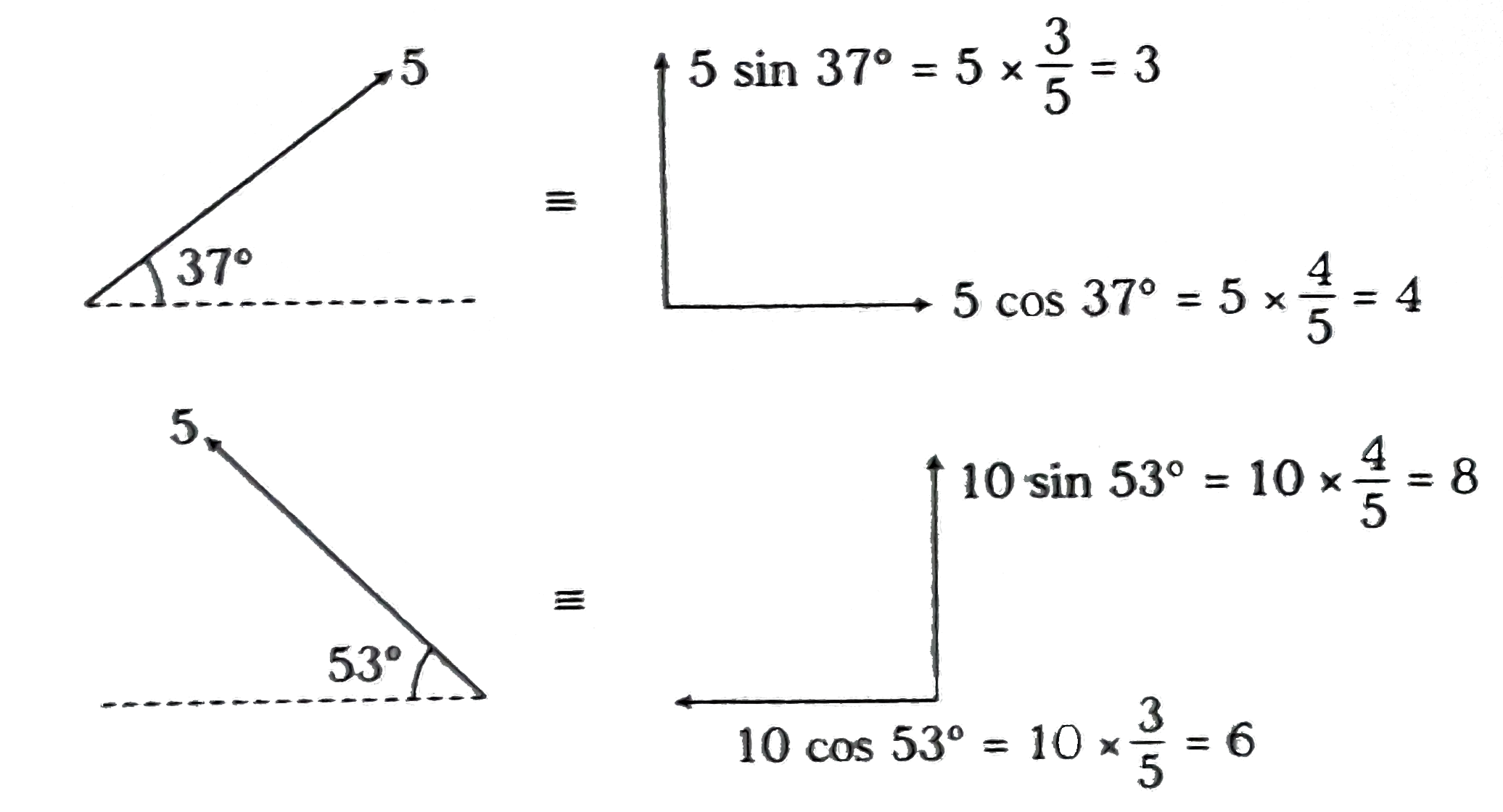
Now combine the components in x and directions, by taking the proper sing.
Fx=4−6+8=6
andFy=3+8−3=8
resultant force ', F=√(6)2+(8)2=10N
Direction of force,tantheta=FyFx=86=43⇒θ=53∘

The resultant of these is 10N at53∘with the positive X-axis.
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
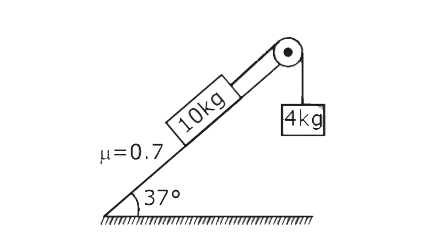
In the arrangement shown in the figure [sin37∘=3/5] .
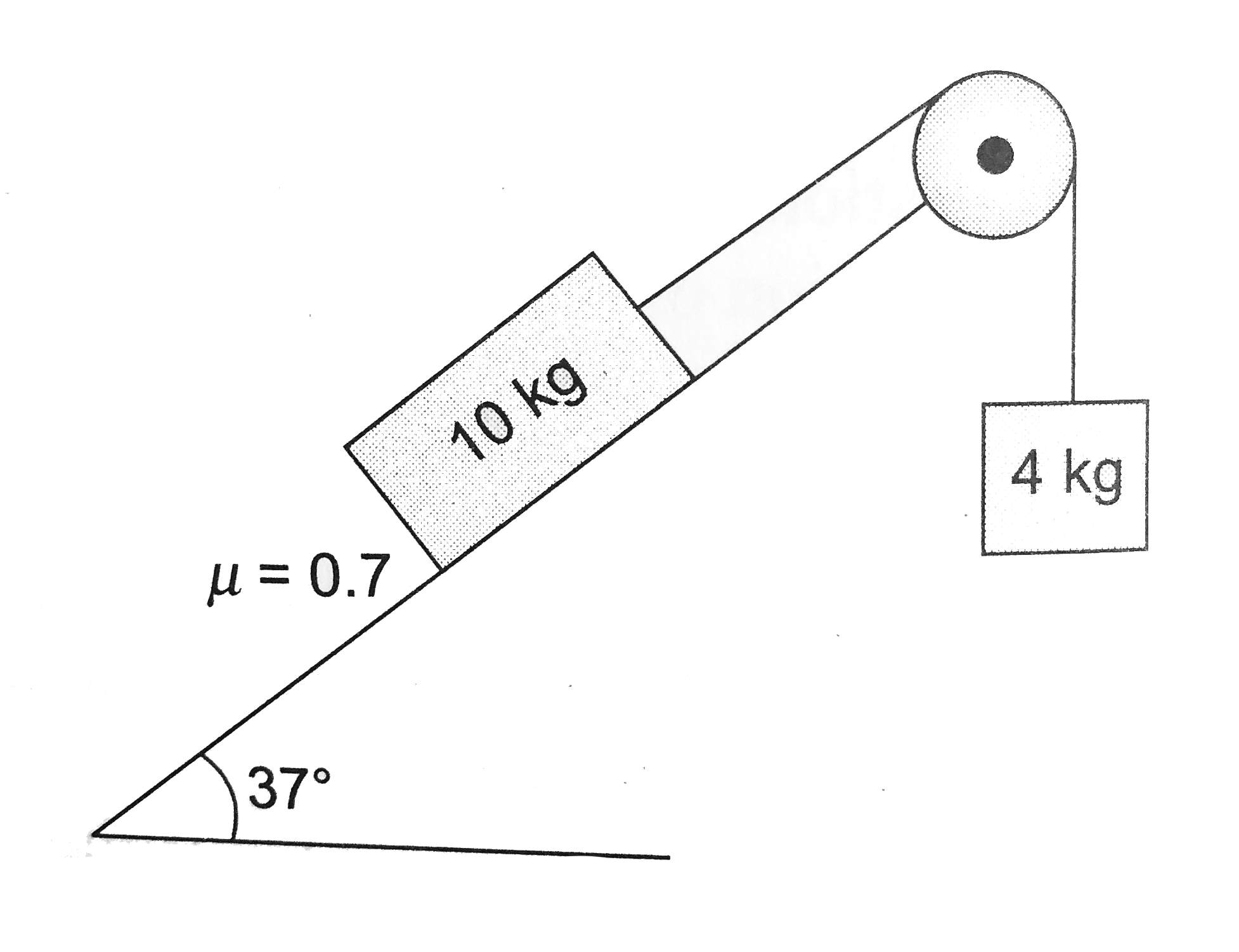 Adirection of force of friction is up the planeBthe mangnitude of force of friction is zeroCthe tension in the string is 20NDmagnitude of force of friction is 56N
Adirection of force of friction is up the planeBthe mangnitude of force of friction is zeroCthe tension in the string is 20NDmagnitude of force of friction is 56NIn the arrangement shown in figure [sin37∘=35]
 Adircetion o fforce of friction is up the planeBthe manitude of force o ffriction is zeroCthe tension in the string 40 NDmagnitude of force of friction is 56N
Adircetion o fforce of friction is up the planeBthe manitude of force o ffriction is zeroCthe tension in the string 40 NDmagnitude of force of friction is 56NFind the resultant amplitude of the following simple harmonic equations :
x1=5sinωt
x2=5sin(ωt+53∘)
x3=−10cosωtA5B10C15D20
Find the distance of the following points from the origin (4,-5,3)
View SolutionWithout using the trigonometric tables, evaluate the following :
117sin70∘cos20∘−47cos53∘cosec37∘tan15∘tan35∘tan55∘tan75∘
View SolutionEvaluate the following :
(i)sin58∘cos32∘
(ii) sec42∘cosec48∘
(iii)tan37∘cot53∘
View Solution∫(cosx)4(sinx)3((sinx)5+(cosx)5)35 dx
View SolutionEvaluate the following: sin(cos−13/5)
View Solution