A uniform chain of length l is placed on a smooth horizontal table, such that half of its length hangs over one edge. It is releasedfrom rest, the velocity with which it leaves the table is
The correct Answer is:A
To solve the problem of finding the velocity with which the chain leaves the table, we can follow these steps:
Step 1: Understand the System
We have a uniform chain of length L placed on a smooth horizontal table, with half of its length (L2) hanging off the edge. When the chain is released from rest, the hanging part will fall due to gravity.
Step 2: Identify the Mass and Center of Mass
The total mass of the chain can be denoted as M. Since half of the chain is hanging, the mass of the hanging part is M2. The center of mass of the hanging part, which is L4 from the edge of the table, will fall a distance of L4 when the chain is released.
Step 3: Calculate the Change in Potential Energy
The change in potential energy (ΔPE) when the chain falls can be calculated as:
ΔPE=mass×g×height
For the hanging part:
ΔPE=(M2)⋅g⋅(L4)=MgL8
Step 4: Relate Potential Energy to Kinetic Energy
When the chain falls, this potential energy converts into kinetic energy (KE). The kinetic energy of the chain when it leaves the table is given by:
KE=12Mv2
Since only half of the mass is falling, the kinetic energy at the point of leaving will be:
KE=12(M2)v2=Mv24
Step 5: Set Up the Energy Conservation Equation
Setting the change in potential energy equal to the kinetic energy gives us:
MgL8=Mv24
Step 6: Solve for Velocity
We can cancel M from both sides (assuming M≠0):
gL8=v24
Multiplying both sides by 4:
gL2=v2
Taking the square root gives us:
v=√gL2
Final Step: Conclusion
Thus, the velocity with which the chain leaves the table is:
v=√gL2
We have a uniform chain of length L placed on a smooth horizontal table, with half of its length (L2) hanging off the edge. When the chain is released from rest, the hanging part will fall due to gravity.
The total mass of the chain can be denoted as M. Since half of the chain is hanging, the mass of the hanging part is M2. The center of mass of the hanging part, which is L4 from the edge of the table, will fall a distance of L4 when the chain is released.
The change in potential energy (ΔPE) when the chain falls can be calculated as:
ΔPE=mass×g×height
For the hanging part:
ΔPE=(M2)⋅g⋅(L4)=MgL8
When the chain falls, this potential energy converts into kinetic energy (KE). The kinetic energy of the chain when it leaves the table is given by:
KE=12Mv2
Since only half of the mass is falling, the kinetic energy at the point of leaving will be:
KE=12(M2)v2=Mv24
Setting the change in potential energy equal to the kinetic energy gives us:
MgL8=Mv24
We can cancel M from both sides (assuming M≠0):
gL8=v24
Multiplying both sides by 4:
gL2=v2
Taking the square root gives us:
v=√gL2
Thus, the velocity with which the chain leaves the table is:
v=√gL2
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - WORK, ENERGY AND POWEREXERCISE - MATCH THE COLUMNS7videos
WORK, ENERGY AND POWER
- BOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based2videos
WORK, ENERGY & POWER
- BOOK - DC PANDEYCHAPTER - WORK, POWER AND ENERGYEXERCISE - E Integer Type Questions11videos
WORK, POWER AND ENERGY
Similar Questions
A chain of length L and mass M is held on a frictionless table with (1/n)th of its length hanging over the edge. When the chain is released, find the velocity of chain while leaving the table.
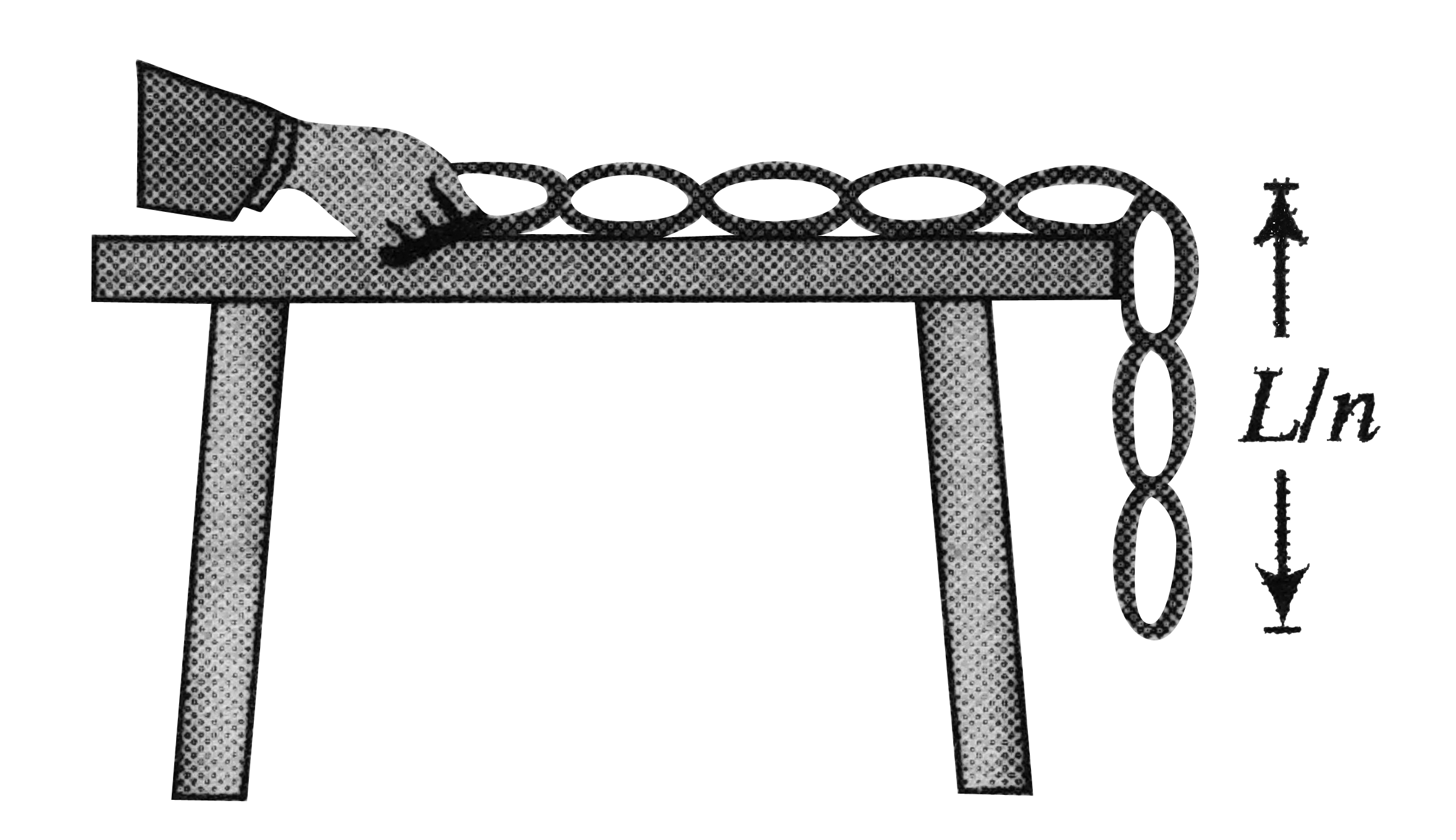 View Solution
View SolutionA uniform chain of mass m and length l is placed on a smooth table so that one−third length hanges freely as shown in the figure. Now the chain is released, with what velocity chain slips off the table?
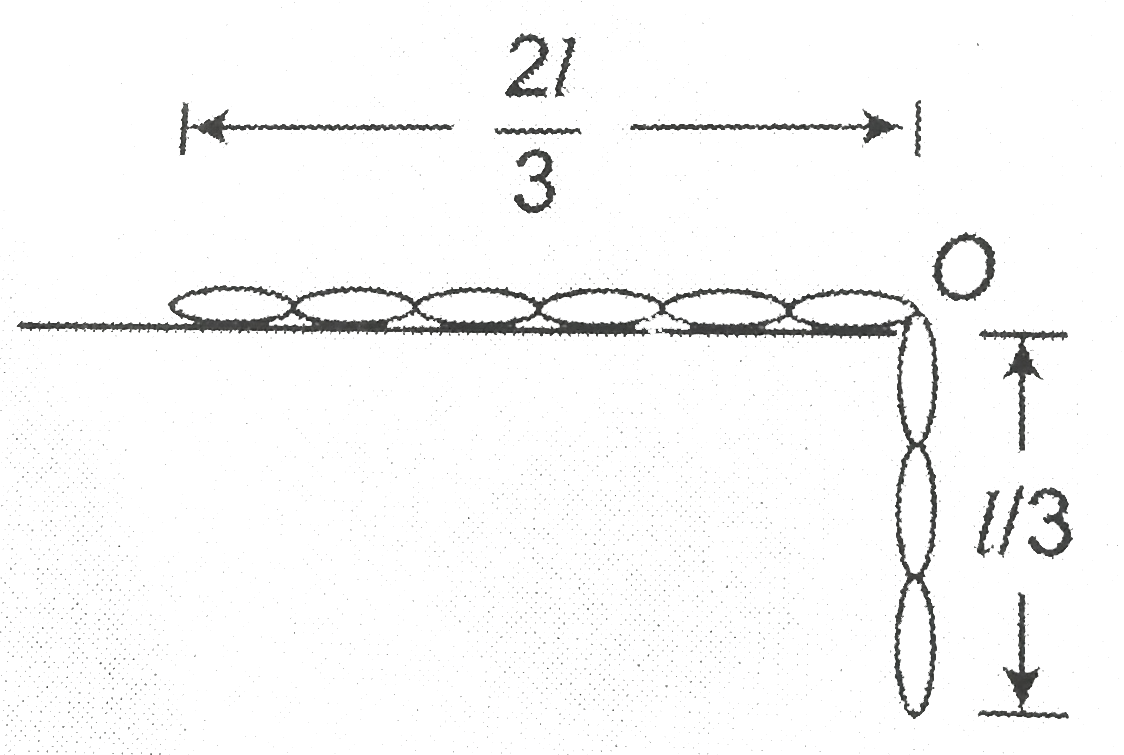 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A uniform chain of length l and mass m is placed on a smooth table with one-fourth of its length hanging over the edge. The work that has to be done to pull the whole chain back onto the table is :
A14mglB18mglC116mglD132mgl - Question 2 - Select One
A uniform chain has mass Mand length L. It is lying on a smooth horizontal table with half of its length hanging vertically downward. The work done in pulling the chain up the table is:
AMgL/2BMgL/4CMgL/8DMgL/16 - Question 3 - Select One or More
A uniform chain of length L lies on a smooth horizontal table with its length perpendicular to the edge of the table and a small portion of the chain is hanging over the edge. The chain starts sliding due to the weight of the hanging part
AThe acceleration of the chain is (gx/L), where x is the length of the hanging part of chain.BThe acceleration of the chain is (g/L)(L−x), where x is the length of hanging part of chain.CThe velocity of the chain is x√gL, where x is the length of hanging part of chain.DThe velocity of the chain is (L−x)√gL, where x is the length of hanging part of chain.
A flexible but inextensible chain of length l is placed on a smooth table with an initial length 1=nl hanging down the table. Calcultae the velocity with which the chain will leave the table if released from rest. Consider two cases : (i) the hanging part does not touch the ground which is at a large distance, (ii) the lower end of the hanging part initially just touches the ground. Also calculate the time in which the chain leaves the table in the first case
[Hint : Taking the table as the datum and m as the mass per unit length of the chain, apply the principle of conservation of energy].View SolutionA uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

What is the speed of the chain, when it just loses contact with the table?View SolutionA uniform chain of mass m and length l is kept on a horizontal table with half of its length hanging from the edge of the table.Work done in pulling the chain on to the table so that only 1/5 th of its length now hangs from the edge is K(mgl200) .Find the value of K.
View SolutionA uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

If the chain is limiting equlibrium, what is the coefficient of friction for the contact between table and chain?View SolutionA uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

If a gentale pull is given to hanging end, it will start moving down. What is the change in gravitational potential energy of the chain, as it jist leaves the table?View Solution

