A metallic rod of length l and cross-sectional area A is made of a material of Young's modulus Y. If the rod is elongated by an amount y, then the work done is proportional to
Y
1/Y
The correct Answer is:C
(c) Volume V=cross-sectional A length l or V=Al
Strain
Young's modulus ,
Work done,
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - ELASTICITYEXERCISE - Chapter Exercise73videos
ELASTICITY
- BOOK - DC PANDEYCHAPTER - ELASTICITYEXERCISE - Match the columns4videos
ELASTICITY
- BOOK - DC PANDEYCHAPTER - ELASTICITYEXERCISE - Check point 12.215videos
ELASTICITY
- BOOK - DC PANDEYCHAPTER - CURRENT ELECTRICITYEXERCISE - All Questions434videos
CURRENT ELECTRICITY
- BOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Integer17videos
ELECTROSTATICS
Similar Questions
A wire of length L and cross sectional area A is made of a material of Young's modulus Y. If the wire is streched by an amount x, the work done is………………
View SolutionA wire of length L and area of cross section A is made of a material of Young's modulus Y. If it is stretched by an amount x, the work done is given by
View Solution
Knowledge Check
- Question 1 - Select One
A wire of length L and cross sectional area A is made of a material of Young's modulus Y. If the wire is stretched by an amount x, the work done is :
AYALxBYALx2CYA2LxDYA2Lx2 - Question 2 - Select One
A wire of length L and cross sectional area A is made of a material of Young's modulus Y. If the wire is stretched by an amount x, the work done is :
AYALxBYALx2CYA2LxDYA2Lx2 - Question 3 - Select One
A wire of length L and area of cross section A is made of a material of Young's modulus Y. If it is stretched by an amount x, the work done is given by
A2YAx2LBYAx2LC12YAx2LD12YAxL
A uniform rod of length cross- sectional area A and Young's modulus Y is subjected to two forces F and 2F at its two ends, stretching the rod.The heat generated in the rod is found to be (10a+b)F2l12AY .Find a+b.{a,b : Positive integers}
View SolutionA wire of length L and cross-sectional area A is made of material of Young's modulus Y. The work done in stretching the wire by an amount x is
View SolutionA metal wire of length L, area of cross-section A and Young's modulus Y behaves as a spring of spring constant k.
View SolutionA metallic rod of length 'L' and cross-section 'A' has Young's modulus 'Y' and coefficent of linear expansion 'α'. If the rod is heated to a temperature. 'T' the energy stored per unit volume is:
View SolutionA uniform cylindrical rod of length L and cross-sectional area by forces as shown in figure.
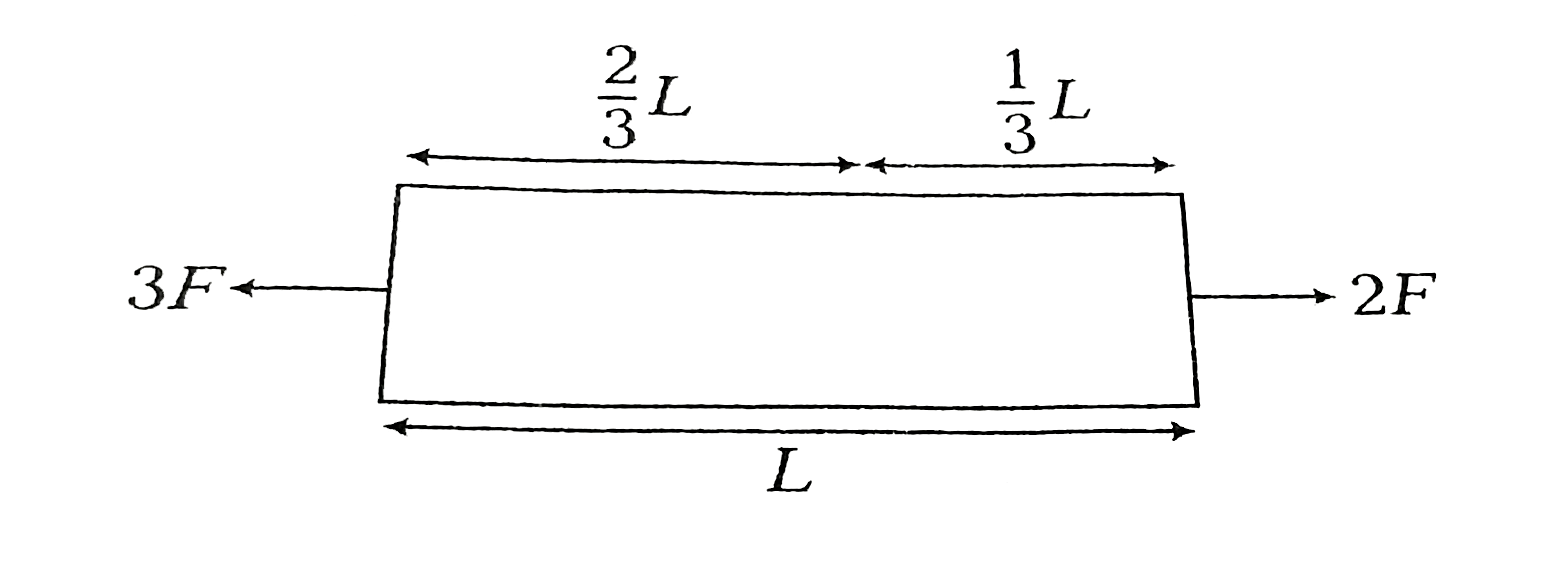
The elongation produced in the rod isView Solution
