A curved glass vessel full of water upto a height of 10 cm has a bottom of area 10cm2, top of area 30cm2 and volume 1 L.
(i) Find the force exerted by the water on the bottom.
(ii) Find the resultant force exerted by the sides of the glass on the water.
(iii) If the glass vessel is covered by a jar and the air inside the jar is completely pumped out, what will be the answers to parts (a) and (b)
(iv) If a glass vessel of different shape is used provided the height, the bottom area and the volume are unchanged, will the answers to parts (a) and (b) change.
(Take, g=10ms−2 , density of water =103kgm−2 and atmospheric pressure =1.01×105Nm−2)
(i) Force exerted by the water on the bottom
F1=(ρ0+ρgh)A1
Here, ρ0= atmospheric pressure =1.01×105Nm−2
ρ= density of water =103kgm−3
g=10ms−2,h=10cm=0.1m
and A1= area of base =10cm2=10−3m2

Substituting these values in Eq. (i), we get
F1=(1.01×105+103×10×0.1)×10−3
or F1=102N (downwards)
(ii) Force exerted by atmosphere on water
F2=(ρ0)A2
Here, A2= area of top =30cm2=3×10−3m2
∴F2=(1.01×105)(3×10−3)
=303 N (dwonwards)
Force exerted by bottom on the water
F3=−F1orF3=102N (upwards)
Weight of water, w=(volume)(density)(g)
=(10−3)(103)(10)
=10 N (downwards)
Let F the force exerted by side walls on the water (upwards). Then for equilibrium of water (FBD)
Net upward force=net downward force
or F+F3=F2+w
∴ F=F2+w−F3=303+10−102
or " " F=211 N (upwards)

(iii) If the air inside the jar is completely pumped out,
F1=(ρgh)A1 (asρ0=0)
=(103)(10)(0.1)(10−3)
=1 N (downwards)
In this case, F2=0
and " " F3=1N (upwards)
∴ F=F2+w−F3
=0+10−1
=9 N (upwards)
(iv) No, the answer will remain the same. Because the answers depend upon ρ0,ρ,g,h,Aa and A2
Topper's Solved these Questions
- FLUID MECHANICSBOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Example 13.7
1 Videos
- FLUID MECHANICSBOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Example 13.8
1 Videos
- FLUID MECHANICSBOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Example 13.5
1 Videos
- EXPERIMENTSBOOK - DC PANDEYCHAPTER - EXPERIMENTSEXERCISE - Subjective
15 Videos
- GENERAL PHYSICSBOOK - DC PANDEYCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
A glass full of water upto a height of 10 cm has a bottom of are 10cm2, top of area 30cm2 and volume 1 litre.
(a) Find the force exerted by the water on the bottom.
(b) Find the resultant force exerted by the sides of the glass on the water.
(c ) If the glass is convered by a jar and the air inside the jar is completely pumped out, what will be the answer to parts (a) and (b).
(d) If a glass of different shape is used, provided the height, the bottom area, the top area and the volume are unchanged, will the answer to parts (a) and (b) change.
Take g=10m/s3, density of water=103kg/m3 and atmospheric pressure =1.01×105N/m2.
View SolutionA glass full of water upto a height of 10 cm has a bottom of are 10cm2, top of area 30cm2 and volume 1 litre.
(a) Find the force exerted by the water on the bottom.
(b) Find the resultant force exerted by the sides of the glass on the water.
(c ) If the glass is convered by a jar and the air inside the jar is completely pumped out, what will be the answer to parts (a) and (b).
(d) If a glass of different shape is used, provided the height, the bottom area, the top area and the volume are unchanged, will the answer to parts (a) and (b) change.
Take g=10m/s3, density of water=103kg/m3 and atmospheric pressure =1.01×105N/m2.
View Solution
Knowledge Check
In the above question, the resultant upward force exerted by the sides of the glass on the water is
A100 NB102 NC303 ND211 NA glass of water upto a height of 10 cm has a bottom of area 10cm2 , top of area 30cm2 and volume 1 litre. The downward force exerted by water on the bottom is…(Taking g=10m/s2 ,density of water =103kg/m3 , atmosphereic pressure =1.01×105N/m2)
A100 NB102 NC110 ND120 NA water container has water upto a height of 20 cm, volume 2 litres. Top of container has area 60 cm2 and bottom has area of 20 cm2. The downward force exerted by water at the bottom will be.
A200 NB100 NC222 ND122 N
A glass full of water has a bottom of area 20cm2, top of area 20cm, height 20cm and volume half a litre.
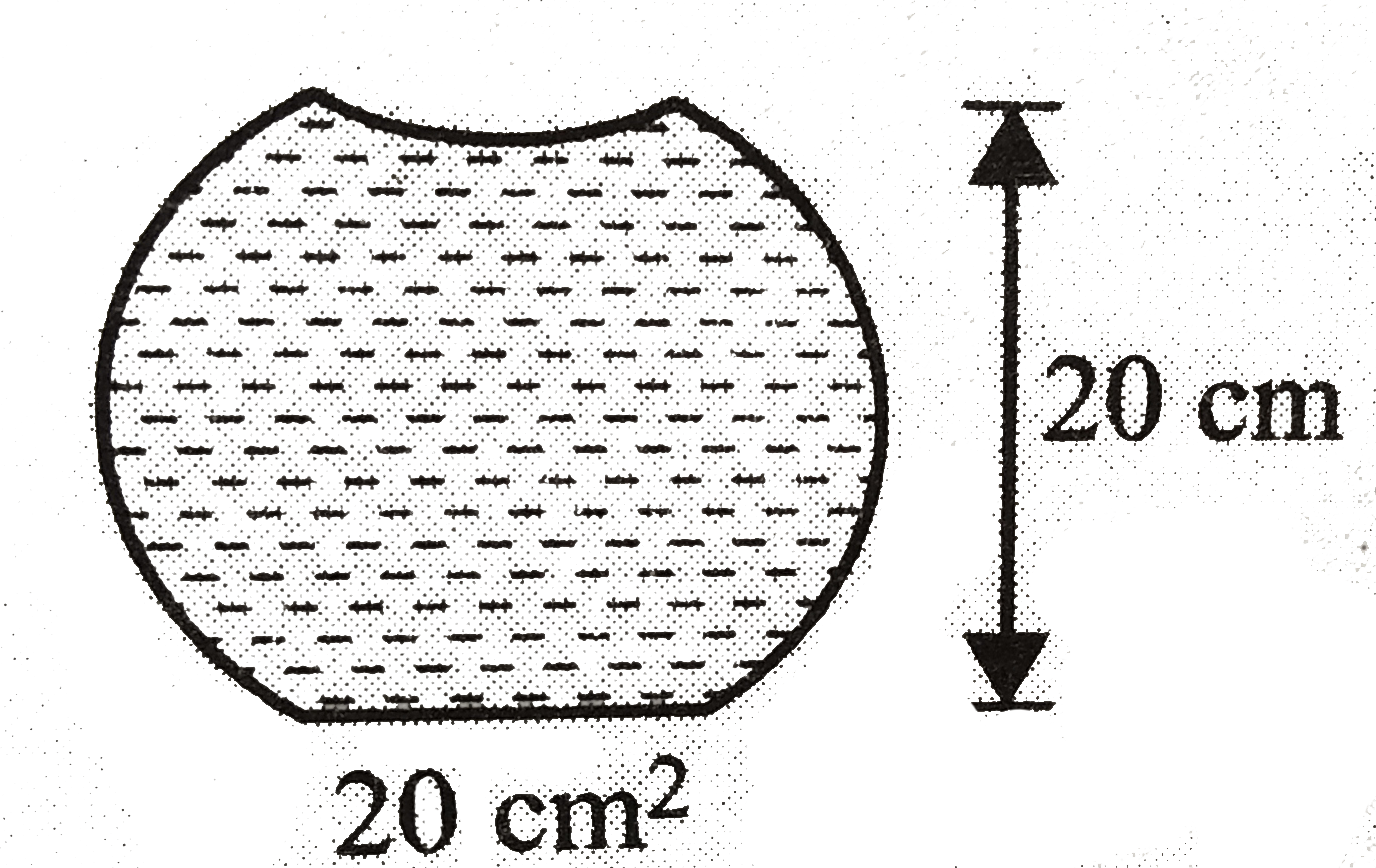
a. Find the force exerted by the water on the bottom.
b. Considering the equilibrium of the v, after. find the resultant force exerted by the side, of the glass on the water. Atmospheric pressure =1.0×105N/m2. Density of water =1000kg/m−3 and g=10m/s2
View SolutionA glass full of water has a bottom of area 20cm2, top of area 20cm, height 20cm and volume half a litre.
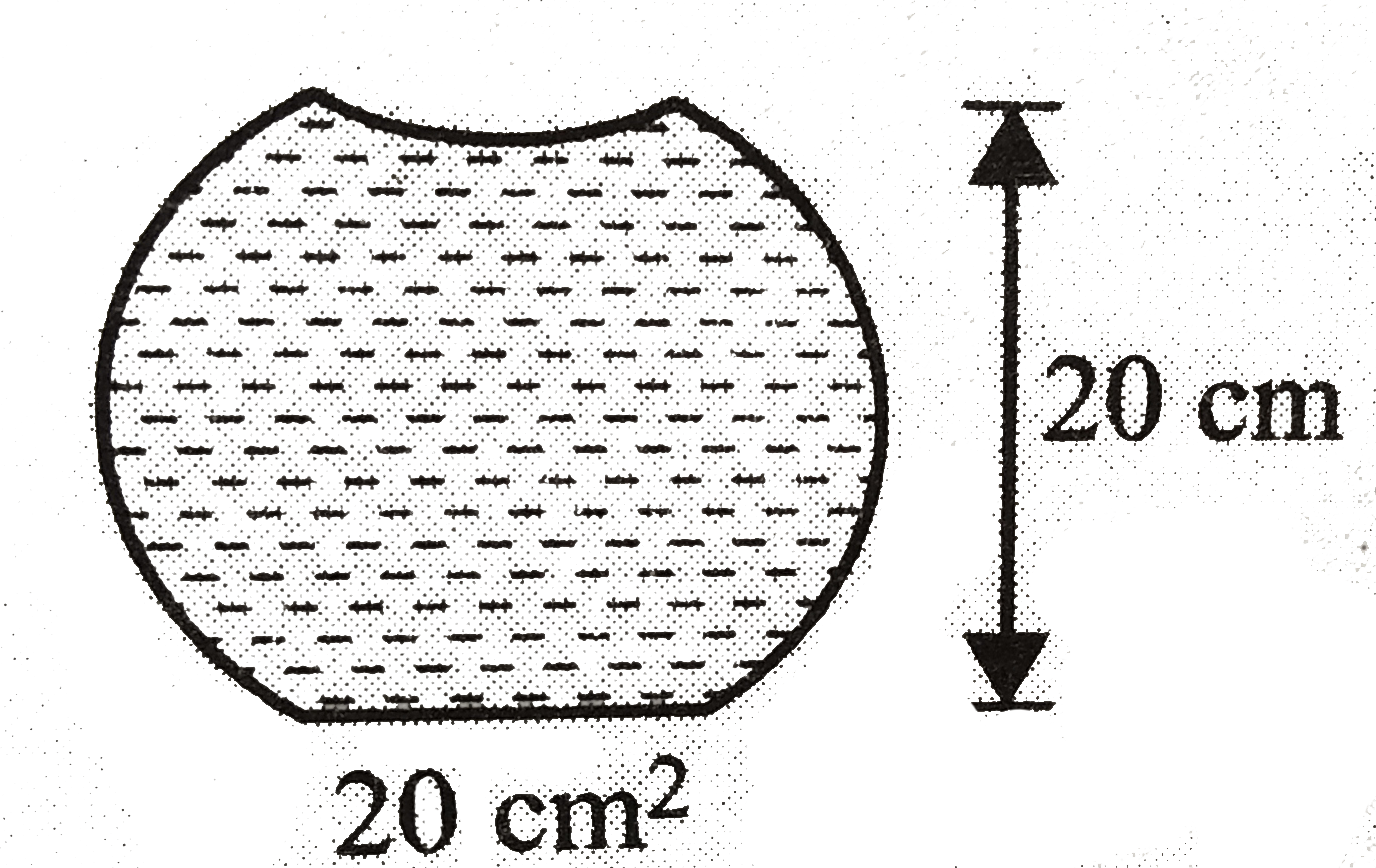
a. Find the force exerted by the water on the bottom.
b. Considering the equilibrium of the v, after. find the resultant force exerted by the side, of the glass on the water. Atmospheric pressure =1.0×105N/m2. Density of water =1000kg/m−3 and g=10m/s2
View SolutionA vessel full of water has a bottom of area 20cm2, top of area 20cm2, height 20 cm and volume half a litre as shown in figure.
(a) find the force exerted by the water on the bottom.

(b) considering the equilibrium of the water, find the resultant force exerted by the sides of the glass vessel on the water. atmospheric pressure =1.0×105N/m2. density of water =1000kg/m3 and g=10m/s2
View SolutionWater is filled in a flask upto a height of 20cm. The bottom of the flask is circular with radius 10cm. If the atmospheric pressure is 1.013×105 Pa, find the force exerted by the water on the bottom . Take g=10ms−2 and density of water =1000kgm−3.
View SolutionWater is filled in a flask up top a heightof 20 cm. The bottom of the flask is circular with radius 10 cm.If the atmospheric pressure is 1.01×105 Pa, find the force exerted by the water on the bottom. Take g=10ms−2 and density of water =1000 kgm^-3.
View Solution
