An AC supply gives 30 Vrms which passes 10Ω resistance. The power dissipated in it is
90√2 W
90 W
45√2 W
45 W
The correct Answer is:B
Power dissipated in circuit
P = /R = (30)2/10 = 90 W
Similar Questions
In the circuit shown power dissipated in 12Ωresistance is 6 watt. What is the power dissipated in the 8Ω resistance.
View SolutionA person with body resistance between his hands of 10KΩ accidentally grasps the terminals of a 18kV power supply.
(i) If the internal resistance of the power supply is 2000Ω, what is the power dissipated in his body?
(ii) What is the power dissipated in his body?
(iii) If the power supply is to be made safe by increasing its internal resistance, what should the internal resistance be for the maximum current in the above situation to be 1.00mA or less?View Solution
Knowledge Check
- Question 1 - Select One
An AC supply gives 30Vrmswhich passes through a 10Ω resistance. The power dissipated in it is
A90√2WB90WC45√2WD45W - Question 2 - Select One
An ac source is connected across a resistance of 10Ω The power dissipated in the resistor is 100W The rms valuse of the current and voltge are .
A√10A,√1000VB2√10A,2√1000VC2√10A,2√1000VD√10A,2√1000V - Question 3 - Select One
An alternating supply of 220 V is applied across a circuit with resistance 22Ω and impedance 44Ω. The power dissipated in the circuit is
A1100 WB550 WC2200 WD(2200/3) W
The emf of the cell is 1.8 V and internal resistance is 2/3Ω, calculate the current in the 3Oemga resistance and the power dissipated in the whole circuit.
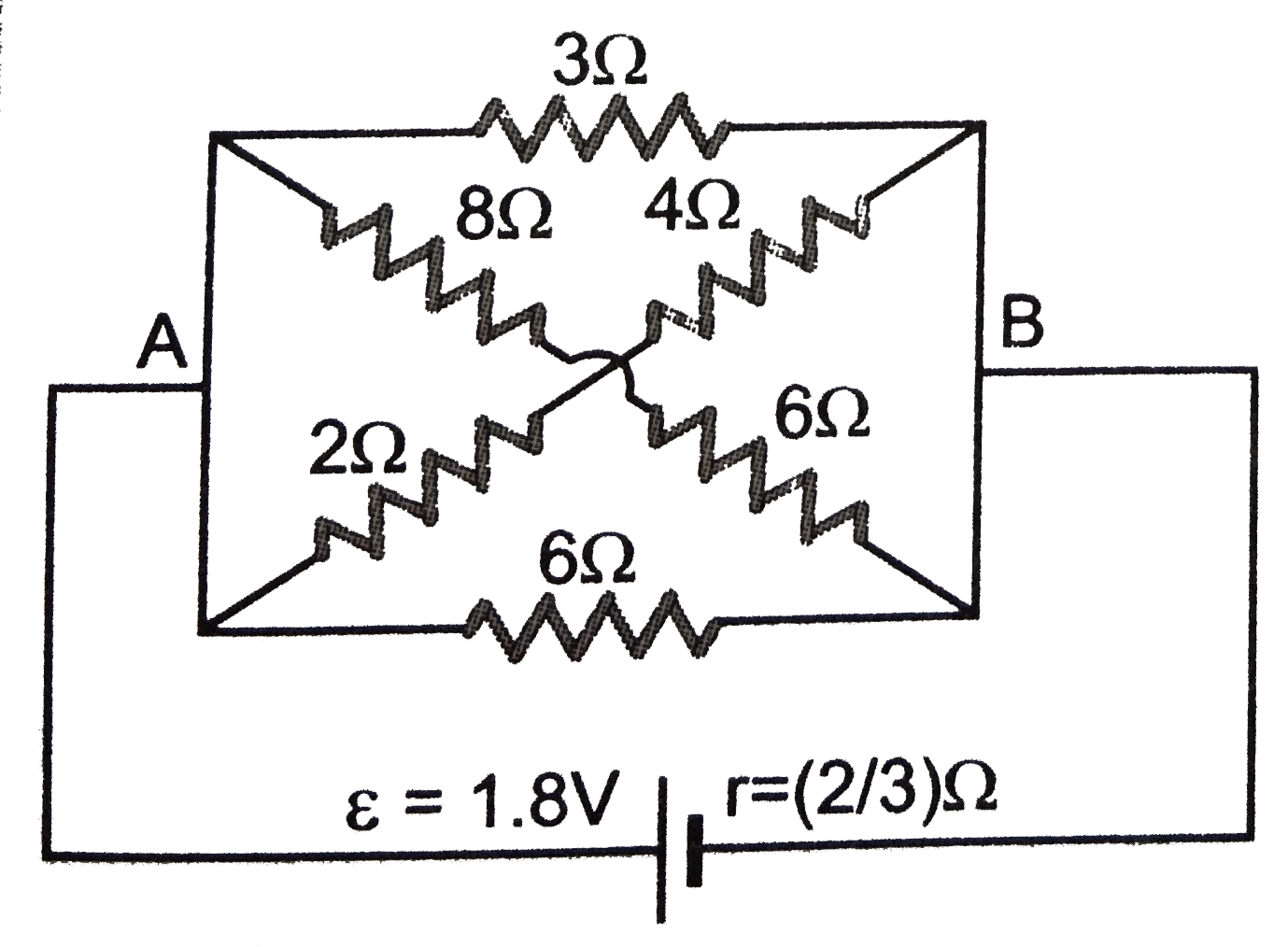 View Solution
View SolutionAs given in the figure a series circuit connected across a 200 V, 60 Hz line consists of a capacitor of capacitive reactance 30Ω , a non-inductive resistor 44Ω , a coil of inductive reactance 9012 and another resistance of resistance 36Ω. The power dissipated in the circuit is
 View Solution
View SolutionAn inductor of reactance 2Ω and a resistor of 4Ω are connected in series to the terminals of a 12 V (rms ) AC source. The power dissipated in the circuit is
View SolutionFour equal resistance dissipated 5 W of power together when connected in series to a battery of negligible internal resistance . The total power dissipated in these resistance when connected in parallel across the same battery would be .
View SolutionAn inductor of reactance 1 Ω and a resistor of 2 Ω are connected in series to the terminals of a 6 V (rms) a.c. source. The power dissipated in the circuit is
View Solution

