A hemispherieal paper weight contains a small flower on its axis of symmetry at a distance of 4 cm from its flat surface. Where is the flower appears to an observer when he looks at it along the axis of symmetry from the top? (Index of refraction of glass = 1.5)

15 cm from top
20 cm from top
5 cm from top
25 cm from top
The correct Answer is:C
(c ) we have, μ2v=μ1u−μ2−μ1R
1v=1.5−6−1−1.5−10
∴ v=-5 cm
Topper's Solved these Questions
- RAY OPTICSBook:DC PANDEYChapter:RAY OPTICSExercise:medical entrance special format question
Explore 25 Videos
- RAY OPTICSBook:DC PANDEYChapter:RAY OPTICSExercise:Medical entrance gallary
Explore 76 Videos
- RAY OPTICSBook:DC PANDEYChapter:RAY OPTICSExercise:Checkpoint 9.7
Explore 10 Videos
- NUCLEIBook:DC PANDEYChapter:NUCLEIExercise:C MADICAL ENTRANCES GALLERY
Explore 46 Videos
- REFLECTION OF LIGHTBook:DC PANDEYChapter:REFLECTION OF LIGHTExercise:Subjective
Explore 9 Videos
Similar Questions
An object is at a distance of d=2.5cm from the surface of a glass sphere with a radius R=10cm. Find the position of the final image produced by the sphere. The refractive index of glass is μ1.5.
View SolutionIn a spherical paper weight (R = 10 cm) made of glass of refractive index μ=32, an object is embedded at a distance 5 cm from its centre. What is the apparent position of the object when seen from the opposite side (see figure)?
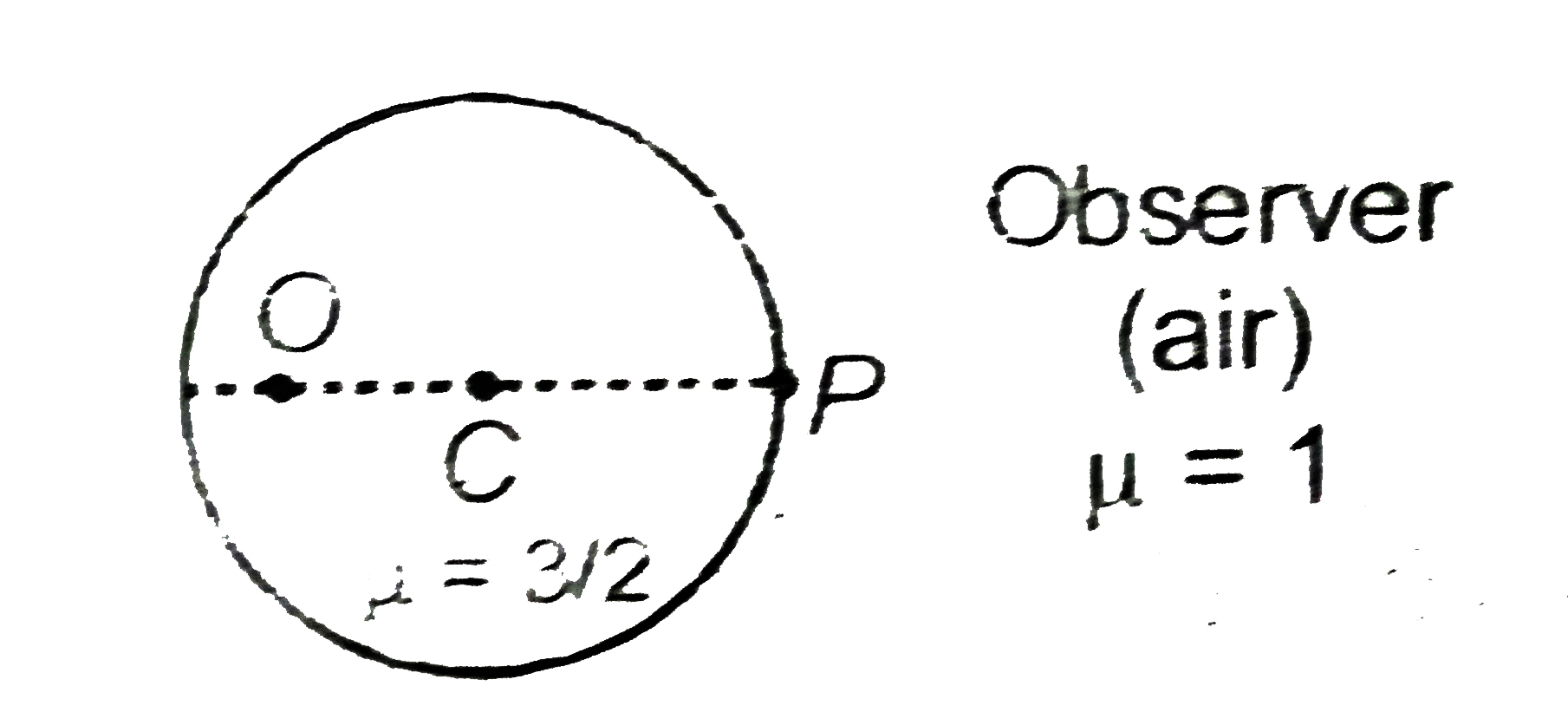
View Solution
Knowledge Check
An image is formed at a distance of 100 cm from the glass surface with refractive index 1.5, when a point object is placed in the air at a distance of 100 cm from the glass surface. The radius of curvature is of the surface is
A20 cmB40 cmC30 cmD50 cmA small ink dot on a paper is seen through s glass slab of thickness 4cm and refractive index 1.5. The dot appears to be raised by
A1cmB2cmC3cmD1.33cm
A glass sphere of radius 5 cm has a small bubble at a distance 2 cm from its centre. The bubble is viewed along a Diameter of the sphere from the side on which it lies.How far from the surface will it appear.Refractive index of glass is 1.5
2.5 cm behind the surface
View SolutionA glass sphere of radius 15 cm has a small bubble 6 cm from its centre. The bubble is viewed along a diameter of the sphere from the side on which it lies. How for from the surface will it appear to be if the refractive index of glass is 1.5 ?
View SolutionA spherical solid glass paper weight of diameter 6 cm has a small air bubble at a distance of 1.5 cm from the centre. If the air bubble be viewed from the side to which it is nearest along the line joining the bubble and the centre, find where will it appear.
View SolutionA glass cube of side 0.6 m contains a small air bubble. It appears to be at a distacne of 0.1 m when observed from the opposite face. Find the actual distance of the bubble from the first face and also the refractive index of glass.
View SolutionRefractive index of the glass slab is 1.5. There is a point object O inside the slab as shown. To eyeE1 object appears at a distance of 6cm (From the top surface) and to eye E2 it appears at a distance of 8cm (from the bottom surface). Find thickness of teh glass slab.
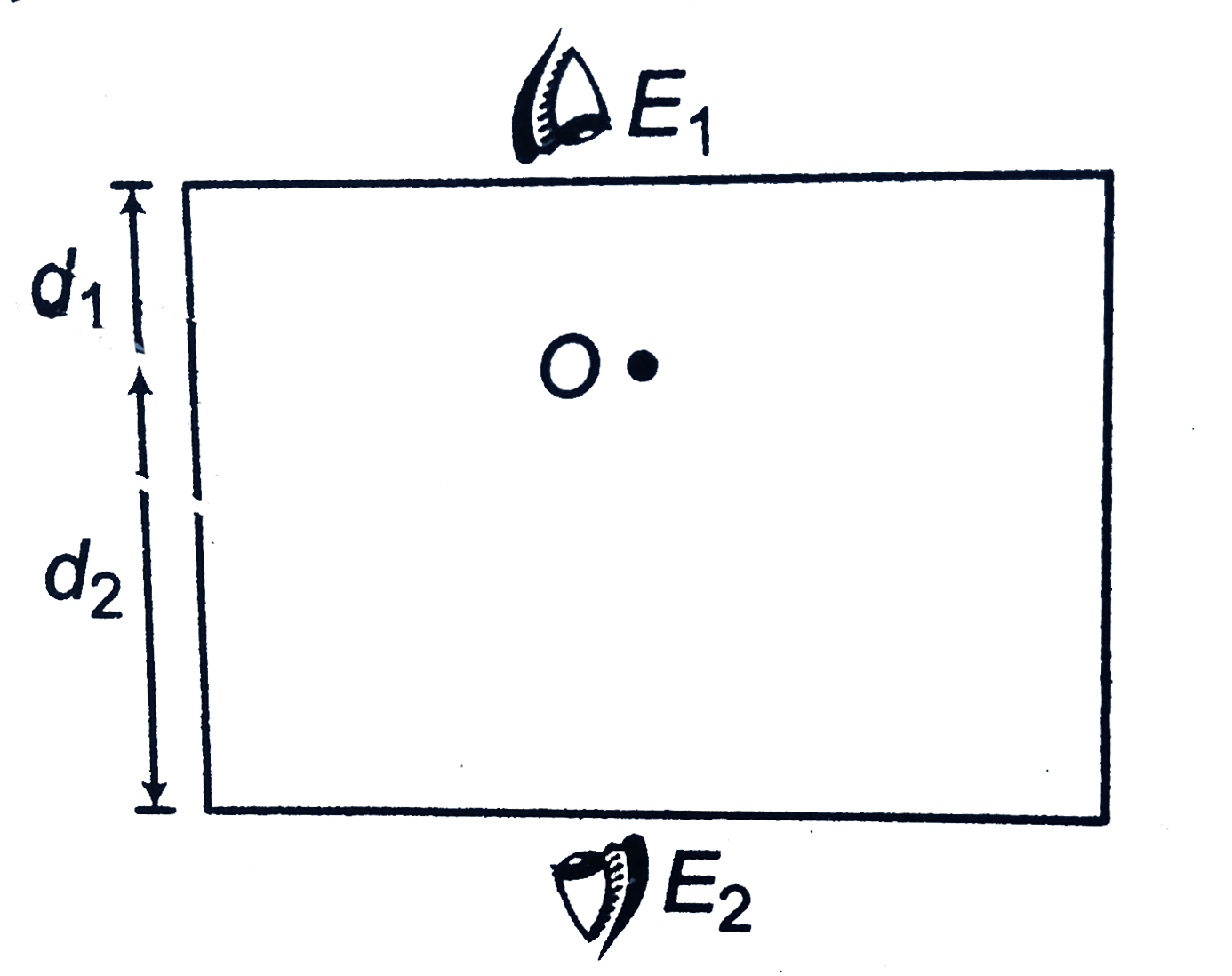
View SolutionA hollow sphere made of a transparent material of refractive index 1.5 has a small mark on its surface as shown. The mark is observed from the farther side. Find the distance of the image of mark from the centre of the sphere.

View Solution
