The Kα X-rays of moylydenum has a wavelength of 71×10−12m. If the energy of molybdenum atom with molybdenum atom when an L-electron removed is (hc=12.42×10−7eV)
17.5 keV
40.82 keV
23.32 keV
5.28 keV
The correct Answer is:D
Given Ek=23.32keV=23.32×1.6×10−19eV
hc=12.42×10−7eV
λhα=71×10−12m
EL−EK=hchkα
EL−23.32×1.6×10−19=12.42×10771×10−12
⇒ EL=5.82keV
Topper's Solved these Questions
Similar Questions
The Kα X-ray of molybdenum has wavelength 0.071 nm. If the energy of a molybdenum atoms with a K electron knocked out is 27.5 keV, the energy of this atom when an L electron is knocked out will be ____________ keV. (Round off to the nearest integer)
[h=4.14×10−15 eVs,c=3×108 ms−1]
View SolutionThe Ka X-ray of molybdenum has wavelength 71 pm. If the energy of a molybdenum atom with a K electron knocked out is 23.32 keV, what will be the energy of this atom when an L electron is knocked out?
View Solution
Knowledge Check
The Kα line from molybdenum (atomic number=42) has a wavelength off .7078Å. The wavelength off Kα line of zinc (atomic number=30) will be
A1ÅB1.3872ÅC0.3541ÅD0.5ÅThe circumference of second orbit of hydrogen atom, if the wavelength of electron is 5×10−9m will be
A5×10−8mB10−8mC10−10mD10−18mIf(0.51×10−104) m is the radius of smallest electron orbit in hydrogen like atom, then this atom is.
Ahydrogen atomBHe+CLi2+DBe3+
The Kα and Kβ X-rays of molybdenum have wavelengths 0⋅71A and0⋅63A respectively. Find the wavelength of Lα X-ray of molybdenum.
View Solutionif λCu is the wavelength of Kα, X-ray line fo copper (atomic number 29) and λMo is the wavelength of the Kα X-ray line of molybdenum (atomic number 42), then the ratio λCuλMois close to
(a) 1.99 (b) 2.14
(c ) 0.50 (d) 0.48
View Solution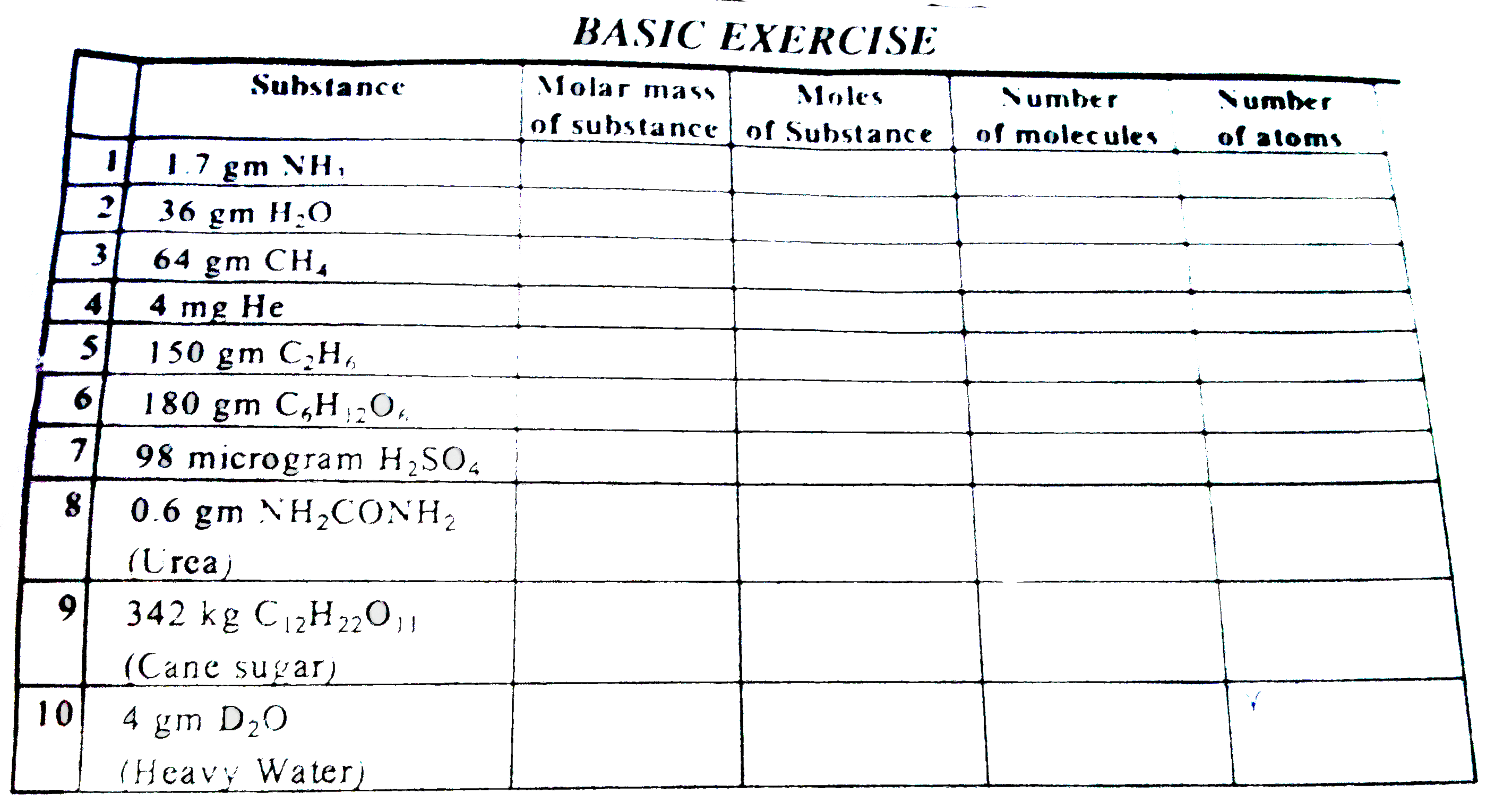
A box contains 12×1022 number of oxygen atoms. Find moles of O-atoms? (NA=6×1023)
View SolutionIf λCu is the wavelength of Kα X-ray line of copper (atomic number 29) and λMo is the wavelength of the KαX-ray line of molybdenum (atomic number 42),then the ratio λCu/λMo is close to
View SolutionDetermine the kinetic energy of an electron that has a de Broglie wavelength equal to twice the diameter of the hydrogen atom. Assume that the hydrogen atom is a sphere of radius 5.3×10−11 m.
View Solution
