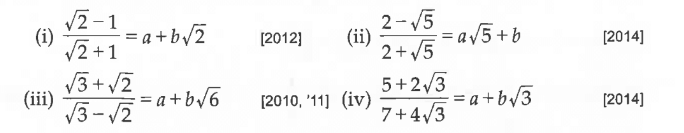Find the number of distinct rational numbers x such that o<x<1andx=p/q , where p,q∈{1,2,3,4,5,6} .
To find the number of distinct rational numbers x such that 0<x<1 and x=pq, where p,q∈{1,2,3,4,5,6}, we can follow these steps:
Step 1: Understand the condition 0<x<1
Since x must be a rational number between 0 and 1, we know that p<q. This means that the numerator p must be less than the denominator q.
Step 2: List possible values for p and q
The values for p and q can be selected from the set {1,2,3,4,5,6}. We will consider each possible value of p and find the corresponding valid values of q.
Step 3: Count valid pairs (p,q)
1. If p=1: Possible values for q are 2,3,4,5,6 (5 options).
2. If p=2: Possible values for q are 3,4,5,6 (4 options).
3. If p=3: Possible values for q are 4,5,6 (3 options).
4. If p=4: Possible values for q are 5,6 (2 options).
5. If p=5: Possible value for q is 6 (1 option).
6. If p=6: No valid q since q must be greater than p.
Step 4: Calculate total combinations
Now, we sum the valid combinations:
- For p=1: 5 options
- For p=2: 4 options
- For p=3: 3 options
- For p=4: 2 options
- For p=5: 1 option
Total combinations = 5+4+3+2+1=15.
Step 5: Identify distinct rational numbers
Next, we need to consider that some combinations may yield the same rational number. We will find distinct values of pq:
- 12,13,14,15,16
- 23,24=12,25,26=13
- 34,35,36=12
- 45,46=23
- 56
Step 6: List distinct values
Now, we will list the distinct rational numbers:
- 16,15,14,13,12,25,23,34,35,45,56
Counting these distinct values gives us a total of 11 distinct rational numbers.
Final Answer
The number of distinct rational numbers x such that 0<x<1 and x=pq is 11.
---
Since x must be a rational number between 0 and 1, we know that p<q. This means that the numerator p must be less than the denominator q.
The values for p and q can be selected from the set {1,2,3,4,5,6}. We will consider each possible value of p and find the corresponding valid values of q.
1. If p=1: Possible values for q are 2,3,4,5,6 (5 options).
2. If p=2: Possible values for q are 3,4,5,6 (4 options).
3. If p=3: Possible values for q are 4,5,6 (3 options).
4. If p=4: Possible values for q are 5,6 (2 options).
5. If p=5: Possible value for q is 6 (1 option).
6. If p=6: No valid q since q must be greater than p.
Now, we sum the valid combinations:
- For p=1: 5 options
- For p=2: 4 options
- For p=3: 3 options
- For p=4: 2 options
- For p=5: 1 option
Next, we need to consider that some combinations may yield the same rational number. We will find distinct values of pq:
- 23,24=12,25,26=13
- 34,35,36=12
- 45,46=23
- 56
Now, we will list the distinct rational numbers:
- 16,15,14,13,12,25,23,34,35,45,56
The number of distinct rational numbers x such that 0<x<1 and x=pq is 11.
Similar Questions
Knowledge Check
Assume that θ is a rational multiple of π such that cosθ is a distinct rational. The number of values of cosθ is
A3B4C5D6
If A={x∣x is prime number and x<30}, find the number of different rational numbers whose numerator and denominator belong to A.
View SolutionFind the number of distinct real solutions of the equation f(f(f(x))=0, where f(x)=x2−1
View SolutionFind the number of distinct solution of secx+tanx=√3
View SolutionFind the cube of rational number 3.1.
View SolutionAre the following pairs of statements negations of each other:
(i) The number x is not a rational number. The number x is not an irrational number.
(ii) The number x is a rational number. The number x is an irrational number.
View SolutionBetween two distinct irrational numbers there lie______rational numbers.
View SolutionShow that −21642875 is the cube of a rational number. Also find the rational number.
View Solution