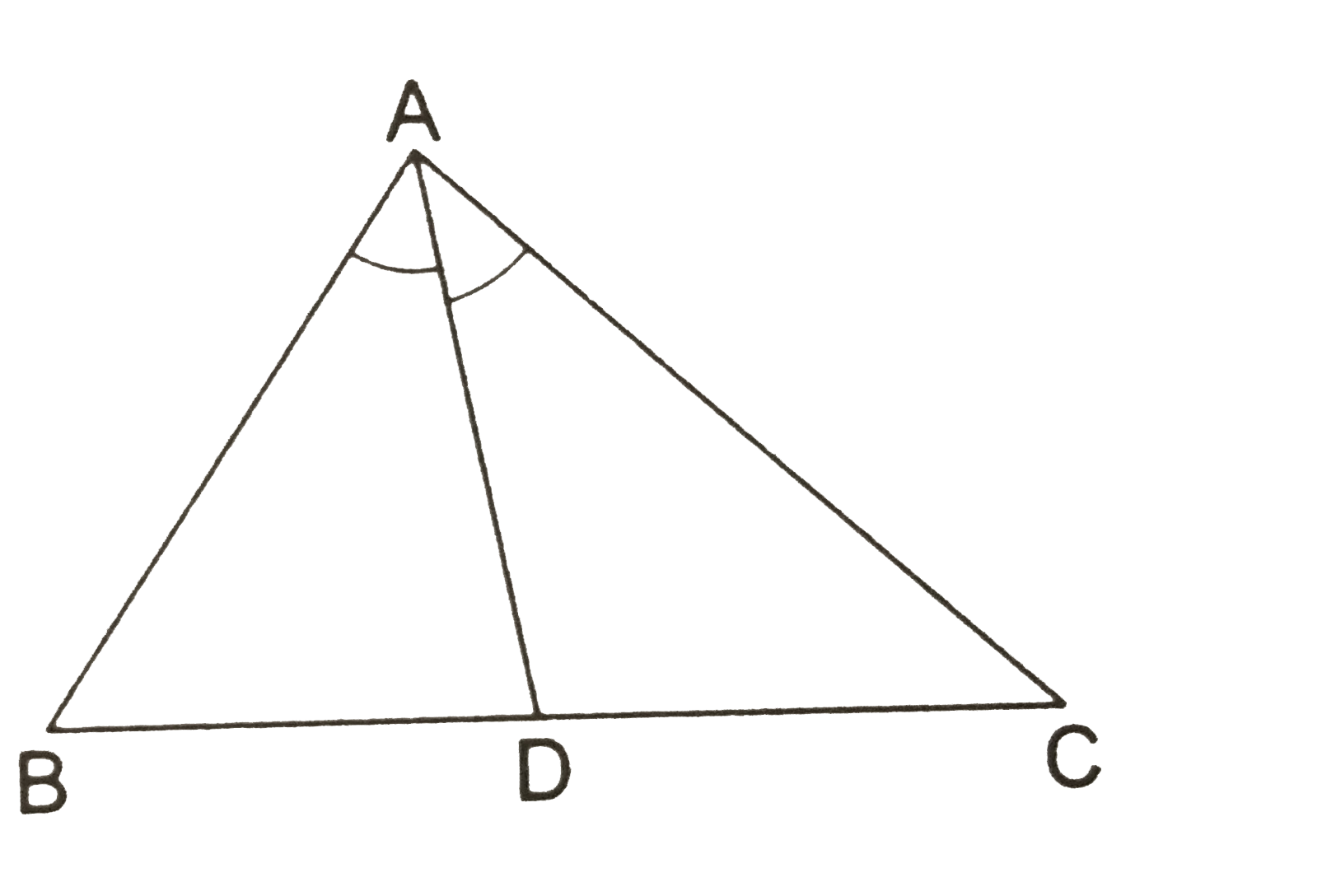
In ΔABC,AD is the bisector of ∠A. Write AB, BD and DC in descending order of their length.
Video Solution
Text Solution
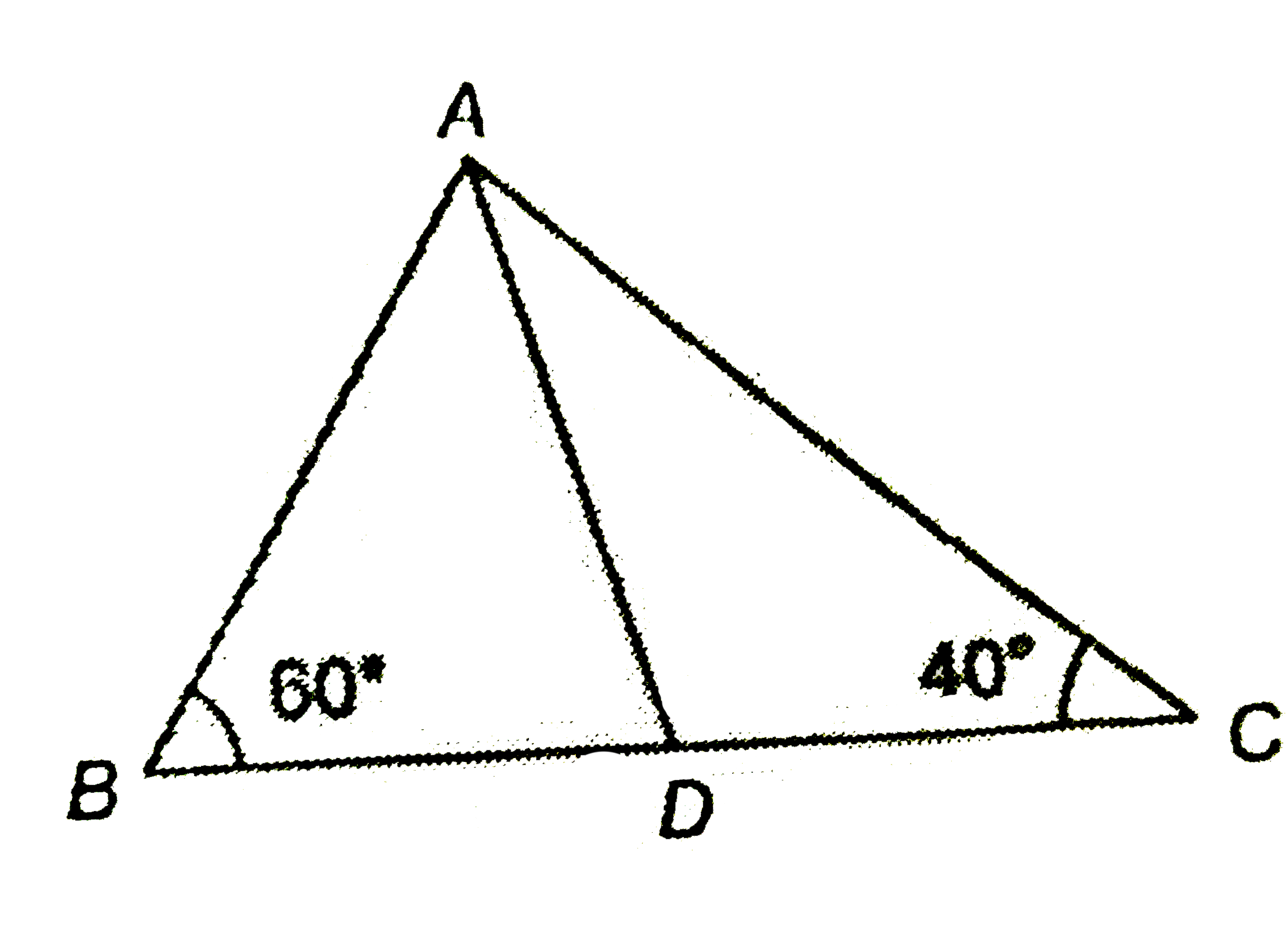
In ΔABC
∠BAC=180∘−∠ABC−∠ACB
=180∘−60∘−40∘=80∘
∴∠BAD=∠CAD=12∠BAC=12×80∘=40∘
In ΔABD
∠ADB=180∘−∠ABD−∠BAD
=180∘−60∘−40∘=80∘
In ΔACD
∠CAD=∠ACD
⇒ CD=AD ...(1)
In ΔABD
∠ADB>∠ABD>∠BAD
⇒ AB>AD>BD
⇒ AB>CD>BD [from (1)]
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLESEXERCISE - Problems From NCERT/exemplar5videos
TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLESEXERCISE - Exercise 7a35videos
TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - SURFACE AREA AND VOLUMEEXERCISE - Revision Exercise (long Answer Questions)10videos
SURFACE AREA AND VOLUME
Similar Questions
Knowledge Check
- Question 1 - Select One
In the adjoining figure, write the sides of ΔABC in descending order of their length.
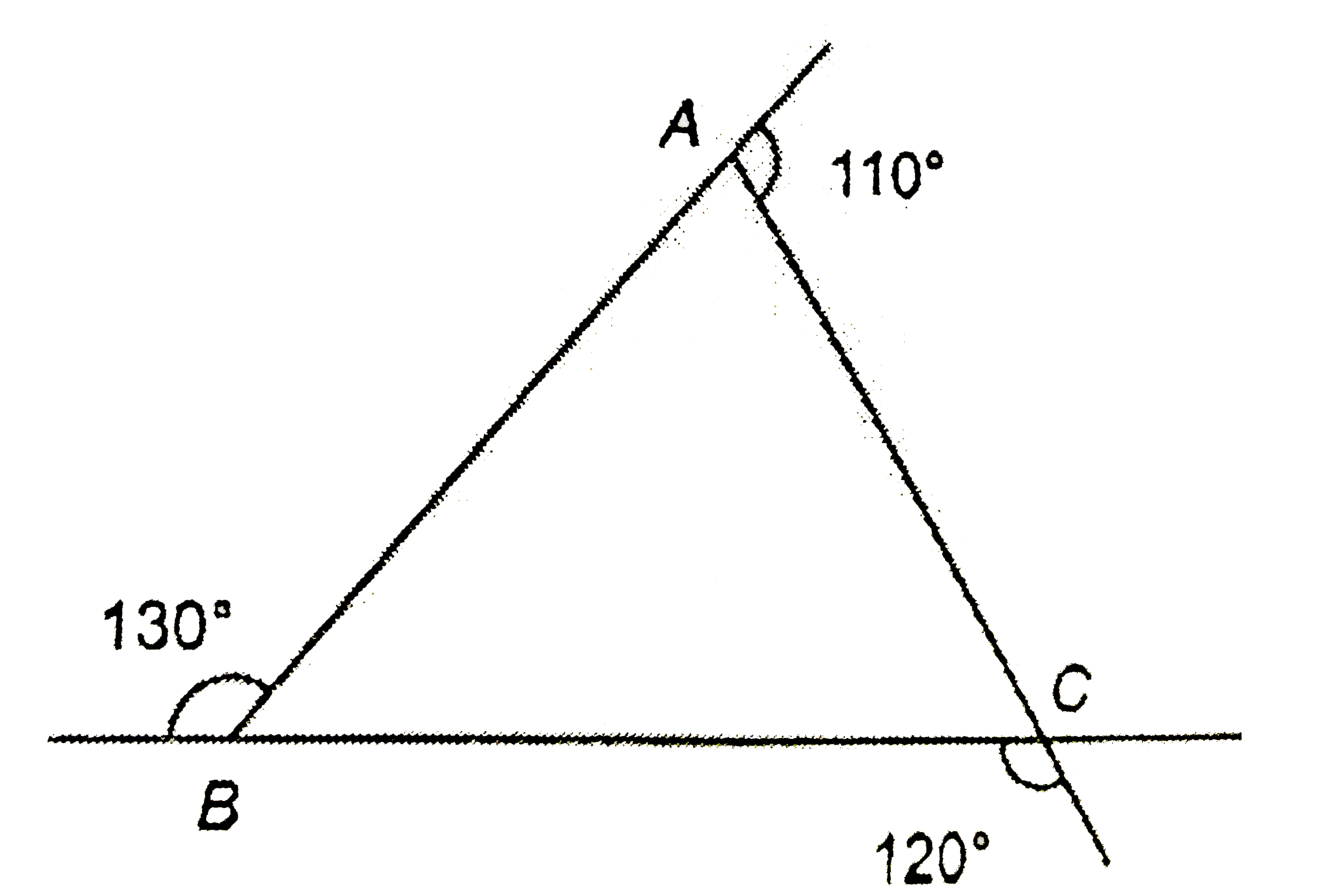 ABC, AB, ACBBC, AC, ABCAB, AC, BCDnone of these
ABC, AB, ACBBC, AC, ABCAB, AC, BCDnone of these - Question 2 - Select One
In ΔABC,AD is the bisector of ∠A . Then , ar(ΔABD)ar(ΔACD)=
AAB2AC2BABACCBMCMDNone of these - Question 3 - Select One
In ΔABC,AB=5cm,AC=7cm. If AD is the angle bisector of ∠A Then BD:CD is :
A25:49B49:25C6:1D5:7
In the figure, AD is the bisector of angle BAC. Prove that AB>BD.
View SolutionIn ΔABC,∠B=35∘,∠C=65∘ and the bisector of ∠BAC meets BC in X. Arrange AX, BX and CX in descending order.
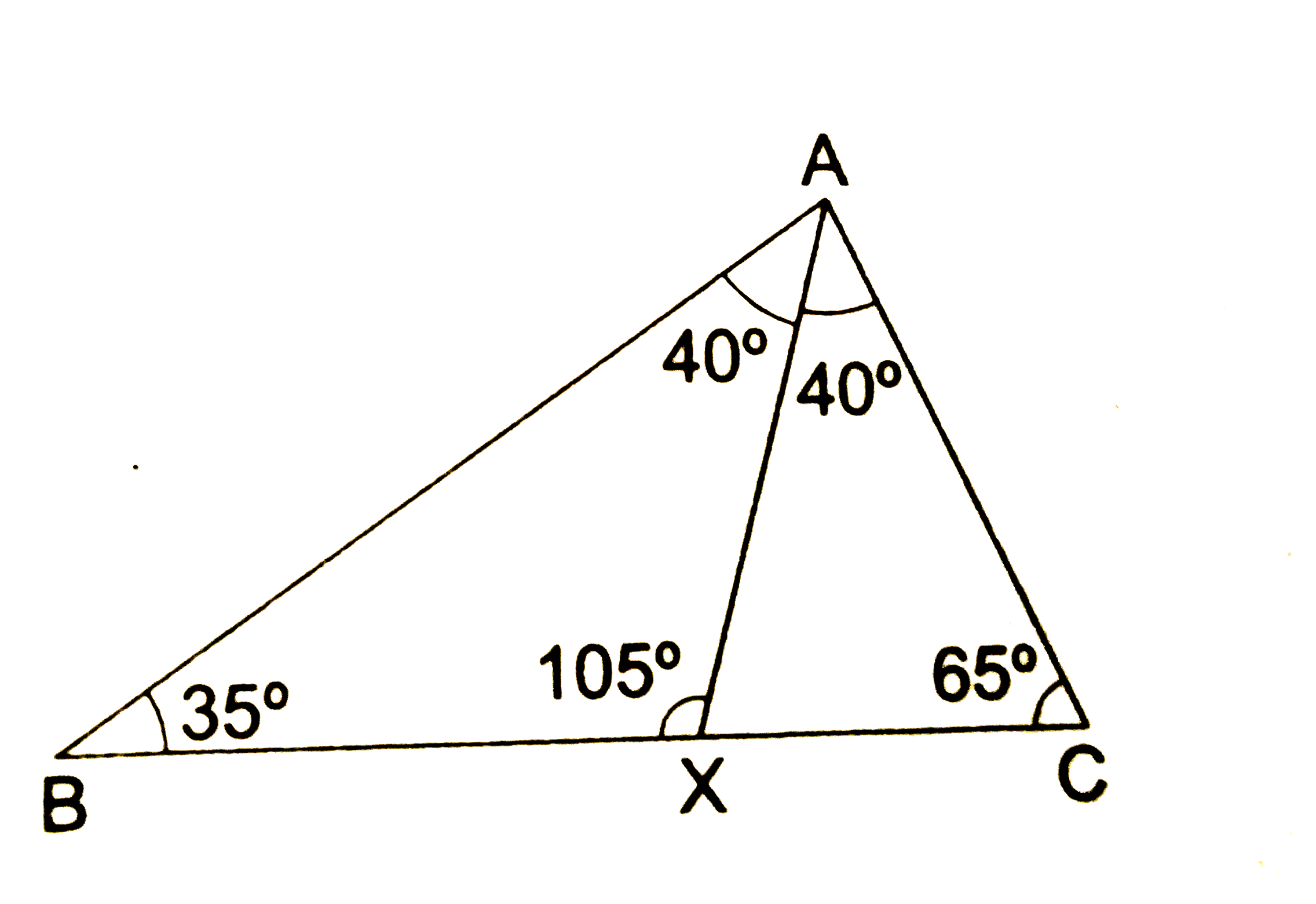 View Solution
View SolutionRay BD is the angle bisector of ∠ABC. The perimeter of ΔABC is
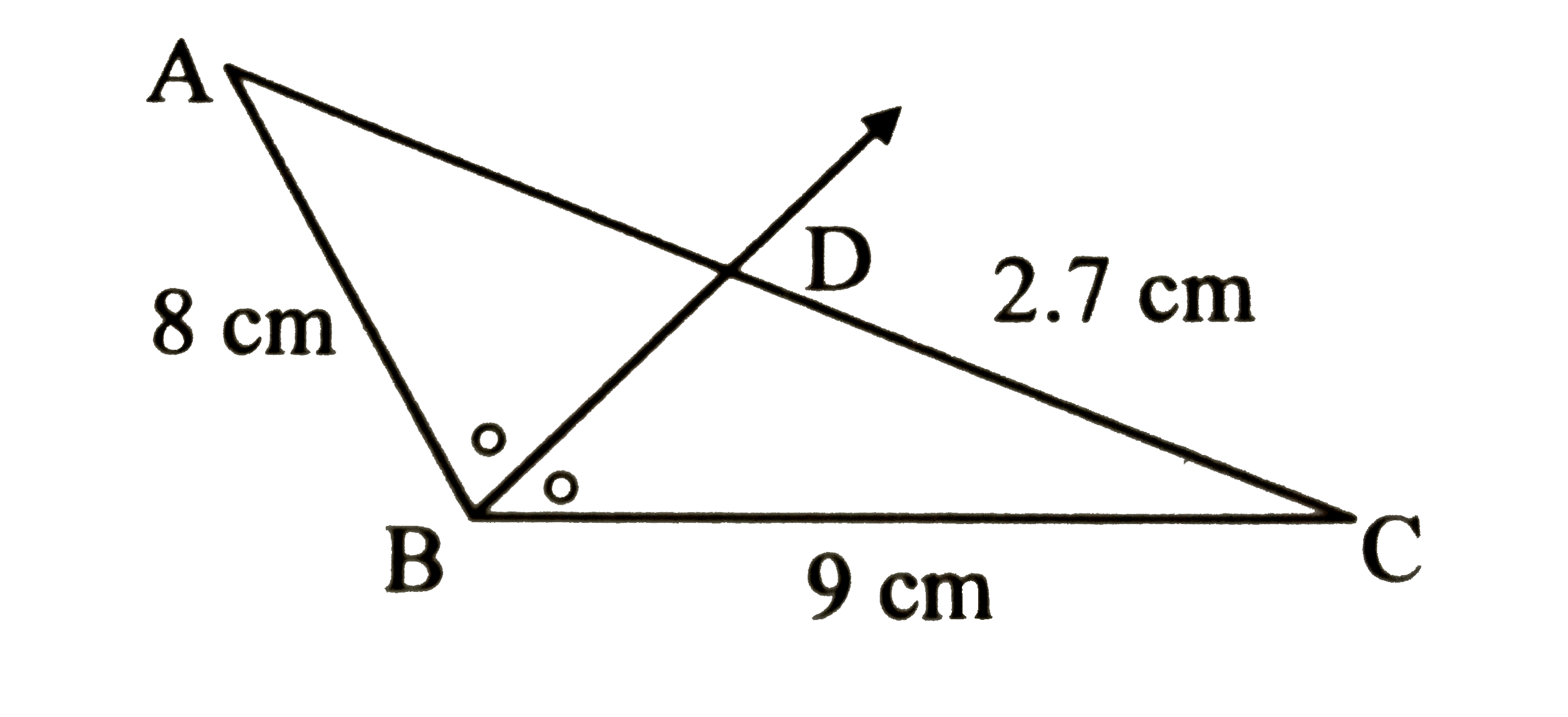 View Solution
View SolutionIn a ΔABC,AD is the bisector of ∠A.
(i) If AB=6.4cm,AC=8cmandBD=5.6cm, find DC.
(ii) If AB=10cm,AC=14cmandBC=6cm, find BD and DC.
(iii) If AB=5.6cm,BD=3.2cm,andBC=6cm, find AC.
(iv) If AB=5.6cm,AC=4cm,andDC3cm, find BC.
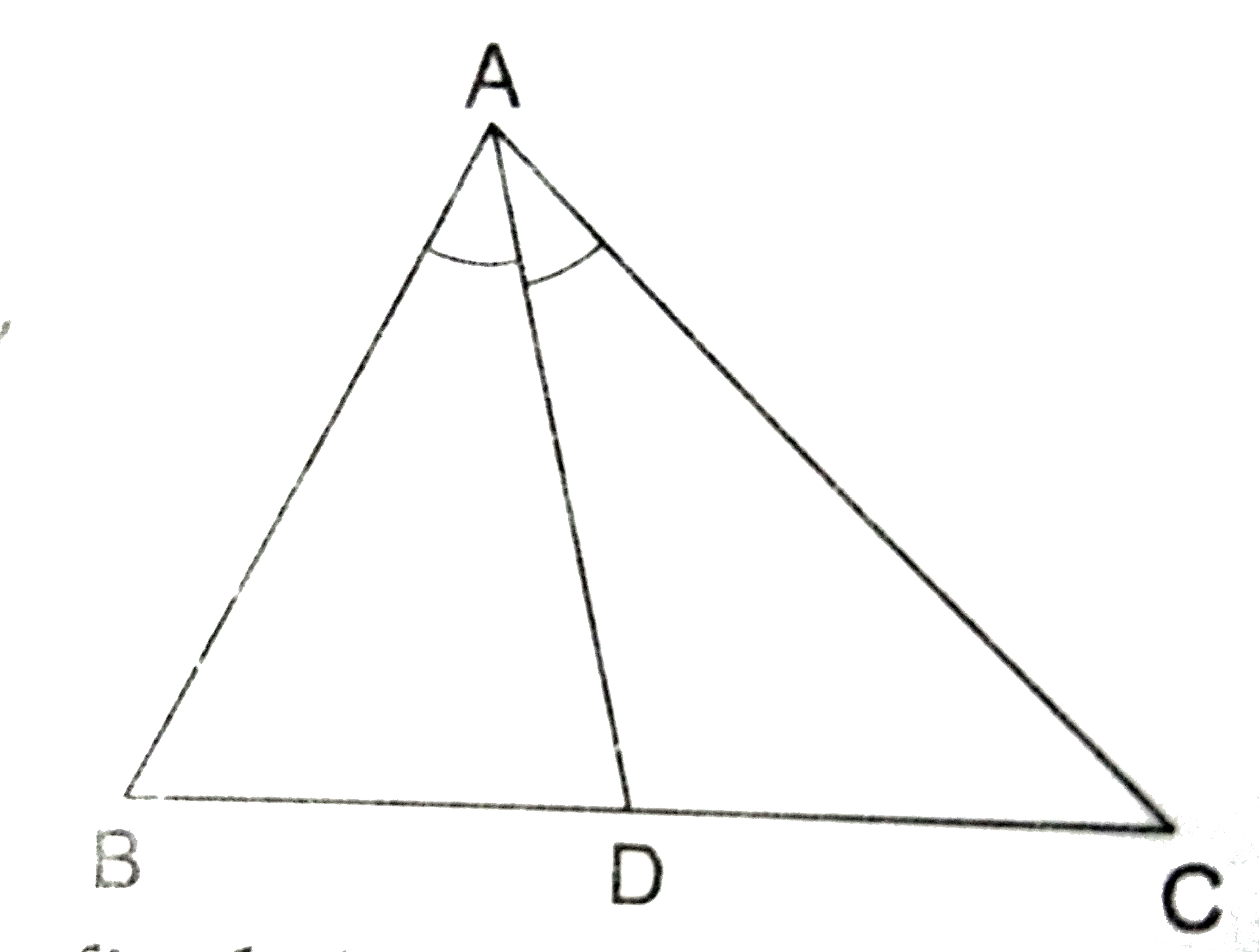 View Solution
View SolutionIn ΔABC,AD is the inernal besector of ∠A. If BD = 5cm , BC=7.5 cm , then AB:AC=
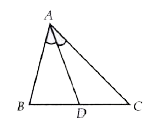 View Solution
View Solution