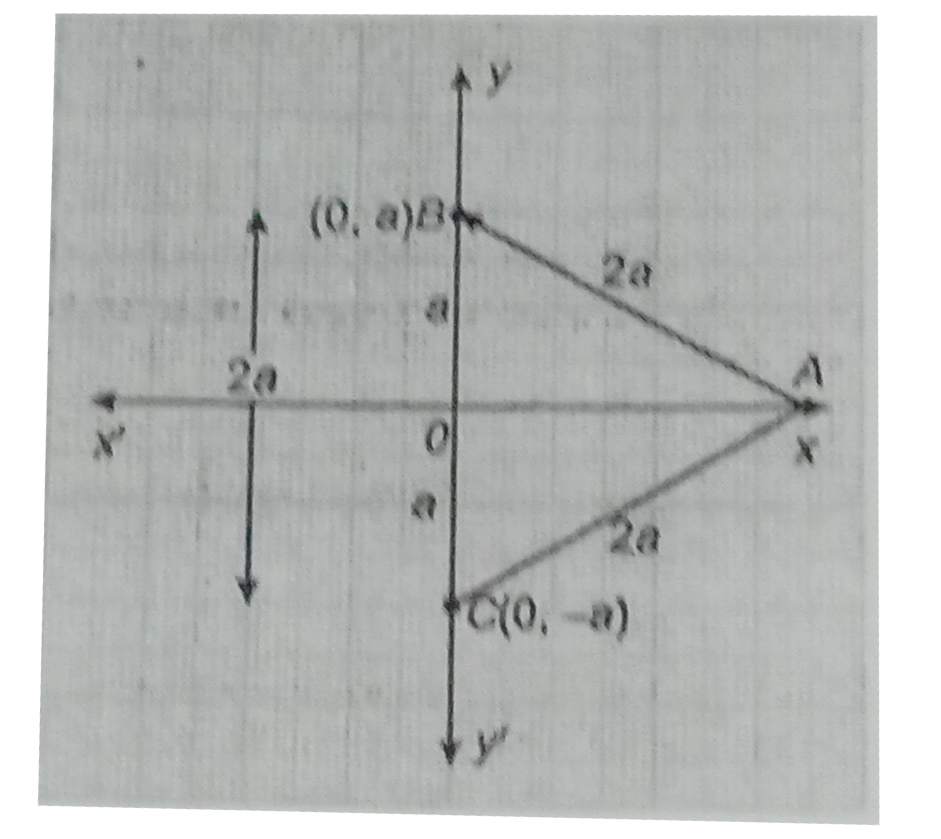
The base f an equilateral triangle with side $2 a$ lies along the y-axis such that the mid point of the base is at the origin. Find the vertices of the triangle.

Video Solution

Text Solution

Verified by Experts

Since, O is the mid- point of baic BC of length $2 a$ .
therefore,
$O B = O C = a$
Also, since it is equilateral triangle, therefore
Now, by Pythagoras theorem,
$A B^{2} = O A^{2} + O B^{2}$
$\Rightarrow O A^{2} = A B^{2} - O B^{2}$
$= {(2 a)}^{2} - a^{2} = 3 a^{2}$
$∴ O A = \sqrt{3 a^{2}} = a \sqrt{3}$
$∴ A = (a \sqrt{3, 0}, B = (0, a) C = (0, - a)$

|

Updated on:21/07/2023

Class 9 MATHS COORDINATE GEOMETRY

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The base f an equilateral triangle with side $2 a$ lies along the y-axis such that the mid point of the base is at the origin. Find the vertices of the triangle.
A $(\sqrt{3} a, 0)$ , (0, a),(0, -a)
B( $\sqrt{3} a$ , 0), (a, 0), (1, -a)
C( $\sqrt{3} a$ , 1), (a, 0), (-a, 0)
D( $\sqrt{3} a$ , 0), (0, a), (-a, 0)
Question 2 - Select One
The vertex of an equilateral triangle is (2,-1) and the equation of its base is x+2y=1, the length of its side is :
A $\frac{2}{\sqrt{15}}$
B $\frac{4}{3 \sqrt{3}}$
C $\frac{1}{\sqrt{15}}$
D $\frac{4}{\sqrt{15}}$

NAGEEN PRAKASHAN-CO-ORDINATE GEOMETRY -Exercise

Home
Ask Doubt
Profile