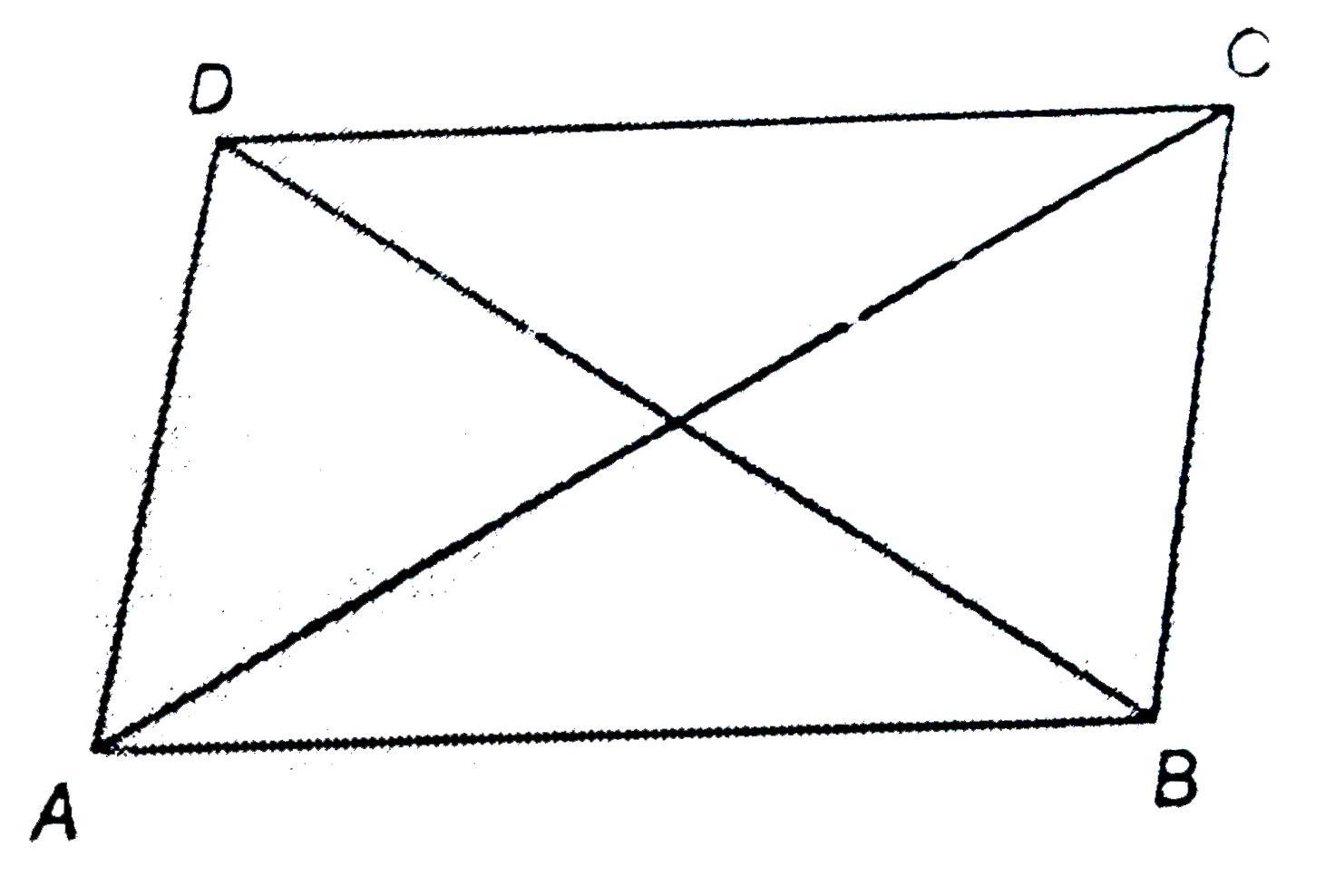
If each diagonals of a quadrilateral separates it into two triangles of equal area then show that the quadrilateral is a parallelogram.
Let AC and BD are two diagonals of quadrilateral ABCD.
Given that, the diagonal AC divides □ABCD into two triangles of equal areas.
∴ area of ΔABC= area of ΔACD
⇒ area of ΔABC=12× Area of □ABCD...(1)
Similarly, the diagonal BD of □ABCD divides it into two triangles of equal areas.
∴ area of ΔABD= area of ΔBCD
⇒ area of ΔABD=12× area of □ABCD...(2)
From (1) and (2), we get
area of ΔABC= area of ΔABD
But these are on the same base AB. (by theorem 5)
∴ Their corresponding height will be same.
⇒ABandDC are parallel.
Similarly, we can prove that AD∣∣BC
∴□ABCD is a parallelogram
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHANCHAPTER - AREA OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Problems From NCERT/exemplar12videos
AREA OF PARALLELOGRAMS AND TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - AREA OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Exercise34videos
AREA OF PARALLELOGRAMS AND TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - CIRCLE EXERCISE - Revision Exercise (long Answer Questions )5videos
CIRCLE
Similar Questions
Prove that If each diagonal of a quadrilateral separates it into two triangles of equal area then show that the quadrilateral is a parallelogram.
View SolutionA diagonal of a parallelogram divides it into two triangles of equal area.
View SolutionIf both the diagonals of a quadrilateral divides it into four triangles of equal area, then the quadrilateral is a rhombus.
View SolutionIf the diagonals AC, BD of a quadrilateral ABCD, intersect at O, and separate the quadrilateral into four triangles of equal area, show that quadrilateral ABCD is a parallelogram.
View SolutionIf the diagonals AC,BD of a quadrilateral ABCD, intersect at O, and seqarate the quadrilateral into four triangles of equal area, show that quadrilateral ABCD is a parallelogram. GIVEN : A quadrilateral ABCD such that its diagonals AC and BD intersect at O and separate it into four parts such that ar(AOB)=ar(BOC)=ar(COD)=ar(AOD) TO PROVE : Quadrilateral ABCD is a parallelogram.
View SolutionShow that the diagonals of a parallelogram divide it into four triangles of equal area.
View SolutionShow that the diagonals of a parallelogram divide it into four triangles of equal area.
View SolutionShow that the diagonals of a parallelogram divide it into four triangles of equal area.
View SolutionIf the diagonals of a quadrilateral bisect each other, then the quadrilateral is a parallelogram.
View SolutionIf the opposite sides of a quadrilateral are equal, prove that the quadrilateral is a parallelogram.
View Solution

