A steel wire of lenght 0.20 m and uniform cross section 10−4m2 is tied rigidly at both ends.The temperature of the wire is change from 40∘ to 20∘C . Calculate the thermal tension in the wire given α=1.1×103/∘C. and Y =2×1011Nm2Solution in Kannada
Step by step video & image solution for A steel wire of lenght 0.20 m and uniform cross section 10^(-4) m^2 is tied rigidly at both ends.The temperature of the wire is change from 40^@ to 20^@C . Calculate the thermal tension in the wire given alpha= 1.1 xx 10^(3)//""^@C. and Y = 2 xx 10^(11)Nm^(2) by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SUPER MODEL QUESTION PAPER 2BOOK - SUBHASH PUBLICATIONCHAPTER - SUPER MODEL QUESTION PAPER 2EXERCISE - QUESTION PAPER -2 (FOR PRACTICE) (PART-D)
10 Videos
- SUPER MODEL QUESTION PAPER 2BOOK - SUBHASH PUBLICATIONCHAPTER - SUPER MODEL QUESTION PAPER 2EXERCISE - QUESTION PAPER -2 (FOR PRACTICE) (PART-B)
8 Videos
- SUPER MODEL QUESTION PAPER 1BOOK - SUBHASH PUBLICATIONCHAPTER - SUPER MODEL QUESTION PAPER 1EXERCISE - QUESTION PAPER -2 (FOR PRACTICE) (PART-A)
1 Video
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - SUBHASH PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - NUMERICAL WITH SOLUTIONS
34 Videos
Similar Questions
State the law of conservation of mechanical energy. Show that total mechanical energy of a body falling freely under gravity is conserved.
View SolutionMention any two contributions of physics to the society.
View SolutionWhat is a dimensional formula ? Give an example for a physical quantity.
View SolutionA non-uniform bar of weight 'w' is suspended at rest by two strings of negligible weight. The angles made by the strings with the vertical are 36.9∘ and 53.1∘C respectively. The bar is 2m long. Calculate the distance 'd' of the centre of gravity of the bar from its left. (refer fig).-NCERT

View SolutionA steel wire of length 1m , mass 0.1kg and uniform cross-sectional area 10^(-6)m^(2) is rigidly fixed at both ends. The temperature of the wire is lowered by 20^(@)C . If transverse calculate the frequency of the fundamental mode of vibration. Given for steel Y = 2 xx 10^(11)N//m^(2) alpha = 1.21 xx 10^(-5) per ^(@)C
View SolutionA steel wire of length 20 cm and uniform cross-section 1mm^(2) is tied rigidly at both the ends. If the temperature of the wire is altered from 40^(@)C to 20^(@)C , the change in tension. [Given coefficient of linear expansion of steel is 1.1xx10^(5) .^(@)C^(-1) and Young's modulus for steel is 2.0xx10^(11) Nm^(-2) ]
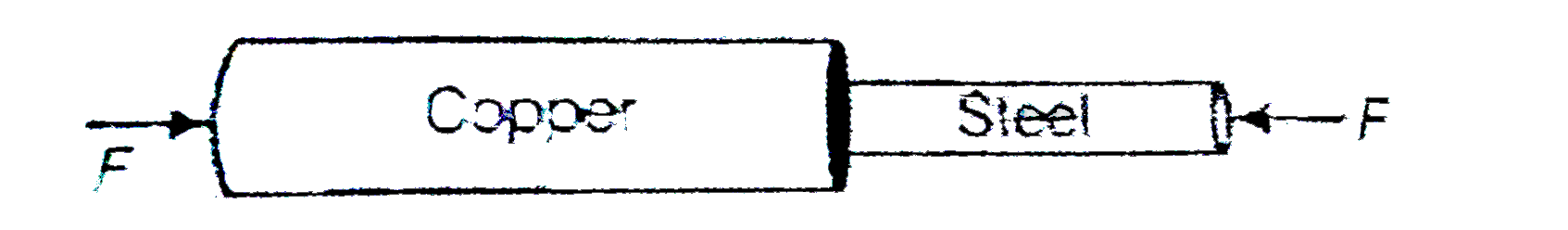
View SolutionTwo wires, one made of capper and other of steel are joined end to end. (as show in figure). The area of cross-section of copper wire is twice that of steel wire, They ae placed under compressive force of magnitude F. Find the ratio of their lenghts such that change in lenght of both wire are same (YS=2×1011N/m2andYC=1.1×1011N/m2)
View SolutionA steel wire of length 20 cm and uniform cross-sectional 1mm2 is tied rigidly at both the ends. The temperature of the wire is altered from 40∘C to 20∘C. Coefficient of linear expansion of steel is α=1.1×10−5.∘C−1 and Y for steel is 2.0×1011Nm2, the tension in the wire is
View SolutionA steel wire of length 20 cm and uniform cross-sectional area of 1 mm^(2) is tied rigidly at both the ends at 45^(@)C . If the temperature of the wire is decreased to 20^(@)C , then the change in the tension of the wire will be [Y for steel = 2 xx 10^(11 Nm^(-2) , the coefficient of linear expansion for steel = 1.1 xx 10^(-5)//.^(@)CC^(-1) ]
View SolutionA force of 20 N is applied at one end of a wire of length 2 m having area of cross-section 10^(-2) cm^(2) . The other end of the wire is rigidly fixed. If coefficient of linear ezpanison of the wire alpha = 8 xx 10^(-6) .^(@)C^(-1) and Young's modulus Y = 22 xx 10^(11) Nm^(-2) and its temperature is increased by 5^(@)C , then the increase in the tension of the wire will be
View SolutionA wire of cross sectional area 3 mm2 is just stretched between two fixed points at a temperature of 20∘C . Then the tension in the wire when the temperature falls to 10∘C is,
(α=1.2×10−5/∘C,Y=2×1011N/m2)
View SolutionA steel wire of length 0.20m and uniform cross section 10^(-6)m^(2) is tied rigidly at both ends. The temperature of the wire is changed from 40^(@) C to 20^(@)C . Calculate the thermal tension in the wire given alpha =1.1. xx 10^(-5)//.^(@)C and Y=2xx10^(11)Nm^(-2)
View SolutionTwo ends of a wire are rigidly clamped. If its temperature is decreased by 10^(@)C , find the change in the tension of the wire. Area of cross-section of the wire =0.01cm^(2) , alpha=16 times 10^(-6@)C^(-1), Y=20 times 10^(11) dyn.cm^(-2)
View SolutionA steel wire of length 20 cm and cross - section 1 mm^(2) is tied rigidly at both ends at room temperature 30^(@)C . If the temperature falls 10^(@)C , what will be the change in tension ? Given that Y = 2xx10^(11)N//m^(2) and alpha = 1.1xx10^(-5)//^(@)C
View Solutionएक स्टील के तार की लम्बाई 20 सेमी तथा एकसमान अनुप्रस्थ परिच्छेद का क्षेत्रफल 1 मिमी2 है। यह अपने दोनों सिरों पर कसा है, तार का तापमान 40∘C से 20∘C तक परिवर्तित होता है। स्टील का रेखीय प्रसार गुणांक α=1.1×10−5/∘C है तथा स्टील का Y=2×1011 न्यूटन/मी2 है। तार में तनाव है
View Solution