Arrive at the expression for the wavelength of electron in terms of electric potential. Solution in Kannada
We know that the wavelength associated with the accelerating electron,
i.e. λe=h√2emV=12.27√V Å
Hence λe∝1√V where V= is the electric potential.
Topper's Solved these Questions
- DUAL NATURE OF RADIATION AND MATTERBOOK - SUBHASH PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - THREE MARK QUESTIONS WITH ANSWERS
5 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - SUBHASH PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - FIVE MARK QUESTIONS WITH ANSWERS
5 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - SUBHASH PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - NUMERICALS WITH SOLUTIONS
21 Videos
- CURRENT ELECTRICITYBOOK - SUBHASH PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - NUMERICAL PROBLEMS AND ANSWERS
29 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - SUBHASH PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - NUMERICALS WITH SOLUTIONS
21 Videos
Similar Questions
Mention any three properties of a photon.
View SolutionWrite any three experimental observations of photoelectric effect
View SolutionA photo has a wavelength of 5000 Å. Calculate its momentum.
View SolutionIn the given circuit, calculate the effective resistance between A and B.
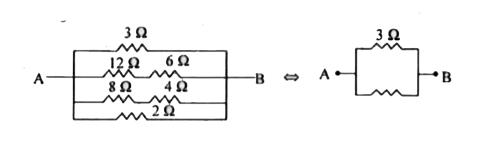
View SolutionTwo point charges −2nC and +8nC are separated in air by a distance of 0.10m.Find the position of the neutral point along the line joining the 2 point charges.
View SolutionExpress the unit of electric potential in terms of the basic units of S.I.
View SolutionState the expression of the electrical conductivity of the material of a semiconductor in terms of the mobilities of electrons and holes.
View SolutionWrite an expression for the wavelength acquired by an electron accelerated by a potential of V volts.
View SolutionExpress electrostatic potential energy in terms of electric potential.
View SolutionArrive at the expression for electric current established due to the motion of electron in its orbit.
View SolutionArrive at the expression for the magnetic flux density at the centre of the circular path of an electron in terms of magnetic moment.
View SolutionArrive at the expression for electric potential at a point due to a dipole and hence apply the expression for electric potential at a point on the dipole axis and on the equatorial lines.
View SolutionDefine electric potential due to a point charge and arrive at the expression for the electric potential at a point due to a point charge.
View SolutionWrite the expression for de-Broglie wavelength of electrons interms of electric potential and explain the terms used.
View SolutionExpress electric potential (V) in terms of work done (W).
View Solution
