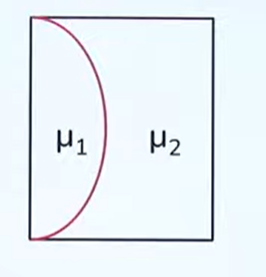
Assertion : The focal length of an equiconvex lens placed in air to radius of curvature of either face.
Reason : For an equiconvex lens radius of curvature of both the faces is same.

A

If both assertion and reason are true and reason is the correct explanation of assertion.

B

If both assertion and reason are true and reason is not the correct explanation of assertion.

C

If assertion is true but reason is false.

D

If both assertion and reason are false.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

For an equiconvex lens, $R_{1} = R_{2} = R$
From, $\frac{1}{f} = (μ - 1) (\frac{1}{R_{1}} - \frac{1}{R_{2}})$
[using lens maker's formula]
For lens, $μ = 1.5$ placed in air.
Therefore, $\frac{1}{f} = (1.5 - 1) \frac{2}{R} \Rightarrow f = R$ .

|

Updated on:21/07/2023

Class 12 PHYSICS GEOMETRICAL OPTICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The focal length of equiconvex lens of retractive index, $μ = 1.5$ and radius of curvature, R is
A $\frac{R}{1.5}$
B $\frac{1.5}{R}$
CR
D1.5R
Question 2 - Select One
The focal length of a double convex lens is equal to radius of curvature of either surface. The refractive index of its material is
A $3 / 2$
B $1$
C $4 / 3$
Dnone of these
Question 3 - Select One
The focal length of a plano-convex lens is equal to its radius of curvature. The value of the refractive index of its material is
A1.33
B1.6
C1.5
D2

NCERT FINGERTIPS-RAY OPTICS AND OPTICAL INSTRUMENTS-Assertion And Reason

Home
Ask Doubt
Profile