A solid cylinder of length L and radius r is heat upto same temperature as that of a cube of edge length a. If both have same material, volume and allowed to cool under similar conditions, then ratio of amount of radiations radiated will be (Neglect radiation emitted from flat surfaces of the cylinder)
a3r
2arL
a2rL
πa22rL
To solve the problem, we need to find the ratio of the amount of radiation emitted by a solid cylinder and a cube, given that they have the same material, volume, and are allowed to cool under similar conditions.
1. Understand the Problem: We have a solid cylinder of length L and radius r, and a cube with edge length a. Both are made of the same material and have the same volume. We need to find the ratio of the heat radiated by the cylinder to that radiated by the cube.
2. Volume Equivalence: Since both objects have the same volume, we can set up the equation for their volumes:
Vcube=a3
Vcylinder=πr2L
Setting these equal gives:
a3=πr2L(1)
3. Surface Area Calculation:
- The surface area of the cube Acube is:
Acube=6a2
- The surface area of the cylinder Acylinder (considering only the curved surface area as per the problem statement) is:
Acylinder=2πrL(2)
4. Apply Stefan-Boltzmann Law: According to the Stefan-Boltzmann law, the amount of heat radiated Q is proportional to the surface area A and the fourth power of the temperature difference. For both bodies, we can write:
Qcube=σAcube(T4−T4surroundings)
Qcylinder=σAcylinder(T4−T4surroundings)
Here, σ is the Stefan-Boltzmann constant.
5. Finding the Ratio of Heat Radiated:
The ratio of heat radiated by the cube to that by the cylinder is:
QcubeQcylinder=AcubeAcylinder
Substituting the areas from (1) and (2):
QcubeQcylinder=6a22πrL
6. Substituting for L: From equation (1), we can express L in terms of a and r:
L=a3πr2
Substituting this into the ratio gives:
QcubeQcylinder=6a22πr(a3πr2)=6a2⋅πr22πra3
Simplifying this:
=6r2a=3ra
7. Final Ratio: Therefore, the ratio of the amount of radiation emitted by the cylinder to that emitted by the cube is:
QcylinderQcube=a3r
Conclusion:
The final answer for the ratio of the amount of radiation emitted by the cylinder to that emitted by the cube is:
QcylinderQcube=a3r
Vcube=a3
Vcylinder=πr2L
Setting these equal gives:
a3=πr2L(1)
- The surface area of the cube Acube is:
Acube=6a2
- The surface area of the cylinder Acylinder (considering only the curved surface area as per the problem statement) is:
Acylinder=2πrL(2)
Qcube=σAcube(T4−T4surroundings)
Qcylinder=σAcylinder(T4−T4surroundings)
Here, σ is the Stefan-Boltzmann constant.
The ratio of heat radiated by the cube to that by the cylinder is:
QcubeQcylinder=AcubeAcylinder
Substituting the areas from (1) and (2):
QcubeQcylinder=6a22πrL
L=a3πr2
Substituting this into the ratio gives:
QcubeQcylinder=6a22πr(a3πr2)=6a2⋅πr22πra3
Simplifying this:
=6r2a=3ra
QcylinderQcube=a3r
The final answer for the ratio of the amount of radiation emitted by the cylinder to that emitted by the cube is:
QcylinderQcube=a3r
Similar Questions
Two solid spheres of radii 5cm and 3cm are made of same material and have similar surface. The spheres are raised to the same temperature and then allowed to cool under identical conditions. The ratio of rate of fall of temperature is
View SolutionA cube, sphere and a cylinder made of same material shown in figure are allowed to cool under identical conditions. Determine which of these will cool at a faster rate?
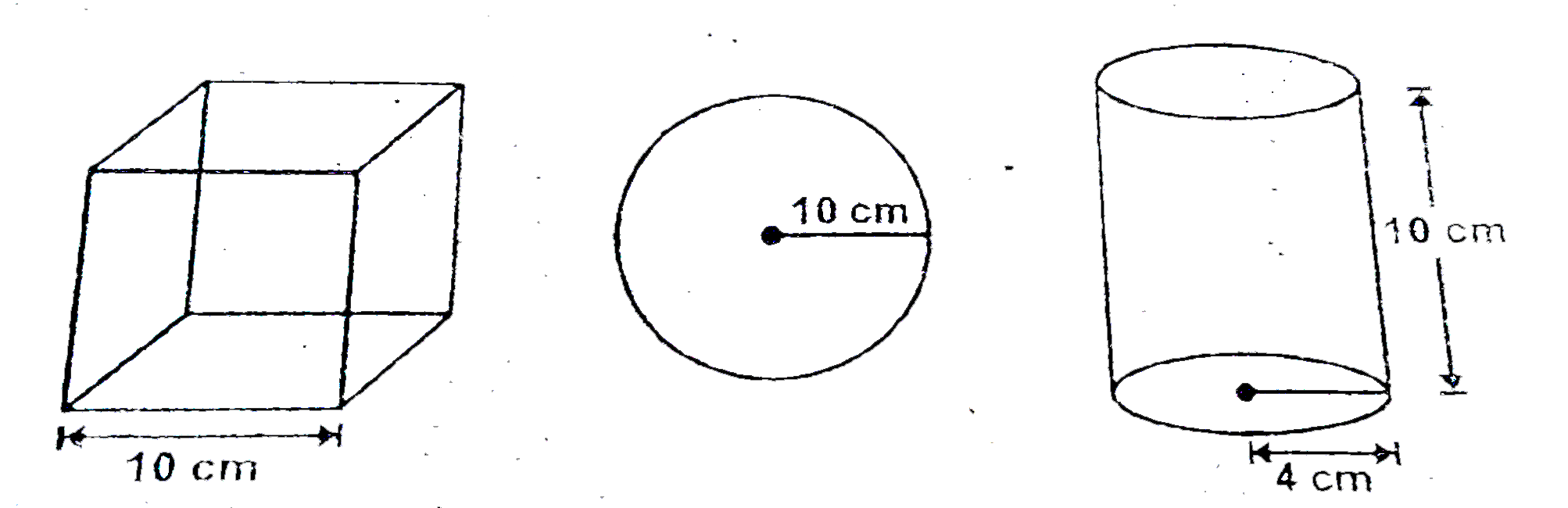 .
.
View Solution
Knowledge Check
A sphere and a cube of same material and same volume are heated up to same temperature and allowed to cool in the same sorroundings. The radio of the amounts of radiations emitted in equal time intervals will be
A1:1B4π3:186400/∘C1C(π6)1/3:1D12(4π3)2/3:1Two solid spheres of radii R1 and R2 are made of the same material and have similar surfaces. These are raised to the same temperature and then allowed to cool under identical conditions. The ratio of their initial rates of loss of heat are
AR1R2BR2R1CR21R22DR22R21A cylinder of length l and radius r is heated to temperature T. A longitudeinal compressive force F is applied on cylinder to keep its length same. Find coefficient of volume expansion.
AFYtπr2B3FYtπr2C2FYtπr2DNone of these
Two ends of rods of length L and radius R of the same material of kept at the same temperature. Which of the following rods conducts the maximum heat?
View SolutionTwo balls of same material and finish have their diameters in the ratio 2:1. Both are heated to the same temperature and allowed to cool by radiation. Rate of cooling of big ball as compared to smaller one will be in the ratio:
View SolutionTwo solid spheres of radii R1 and R2 are made of same material and have similar surface. The spheres are raised to the same temperature and then allowed to cool under identical conditions. Assuming spheres to be perfect conductors of heat, their initial ratio of rates of loss of heat is:
View SolutionTwo spheres A and B have radius but the heat capacity of A is greater than that of B. The surfaces of both are painted black. They are heated to the same temperature and allowed to cool. Then initially
View SolutionTwo unifrom brass rod A and B of length l and 2l and radii 2r respectively are heated to the same temperature. The ratio of the increase in the volume of A to that of B is
View Solution
