For the damped oscillator shown in previous figure, k=180Nm−1 and the damping constant b is 40gs−1 .Period of oscillation is given as 0.3 s, find the mass of the block . (Assume b is much less than √km).
To find the mass of the block in the damped oscillator, we can use the relationship between the period of oscillation, the spring constant k, and the mass m. The formula we will use is derived from the damped oscillation equations.
1. Identify the Given Values:
- Spring constant k=180N/m
- Damping constant b=40g/s=0.04kg/s (convert grams to kilograms)
- Period of oscillation T=0.3s
2. Relate Period to Angular Frequency:
The angular frequency ω′ for a damped oscillator is given by:
ω′=2πT
Substituting the value of T:
ω′=2π0.3
3. Calculate ω′:
ω′=2×3.140.3≈20.94rad/s
4. Use the Approximation for Damped Oscillation:
Since b is much less than √km, we can use the approximation:
ω′≈√km
5. Square Both Sides:
ω′2=km
Rearranging gives:
m=kω′2
6. Substitute the Values:
Substitute k=180N/m and ω′≈20.94rad/s:
m=180(20.94)2
7. Calculate m:
m=180438.58≈0.410kg
8. Final Answer:
The mass of the block is approximately 0.41kg.
- Spring constant k=180N/m
- Damping constant b=40g/s=0.04kg/s (convert grams to kilograms)
- Period of oscillation T=0.3s
The angular frequency ω′ for a damped oscillator is given by:
ω′=2πT
Substituting the value of T:
ω′=2π0.3
ω′=2×3.140.3≈20.94rad/s
Since b is much less than √km, we can use the approximation:
ω′≈√km
ω′2=km
Rearranging gives:
m=kω′2
Substitute k=180N/m and ω′≈20.94rad/s:
m=180(20.94)2
m=180438.58≈0.410kg
The mass of the block is approximately 0.41kg.
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTECHAPTER - OSCILLATIONSEXERCISE - ASSIGNMENT ( SECTION -A)58videos
OSCILLATIONS
- BOOK - AAKASH INSTITUTECHAPTER - OSCILLATIONSEXERCISE - ASSIGNMENT (SECTION-B )25videos
OSCILLATIONS
- BOOK - AAKASH INSTITUTECHAPTER - OSCILLATIONSEXERCISE - Assignment (Section D) (ASSERTION-REASON TYPE QUESTIONS)13videos
OSCILLATIONS
- BOOK - AAKASH INSTITUTECHAPTER - NUCLEIEXERCISE - ASSIGNMENT (SECTION-D)10videos
NUCLEI
- BOOK - AAKASH INSTITUTECHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)5videos
PHYSICAL WORLD
Similar Questions
For the damped oscillator shown in Fig, the mass of the block is 200g,k=80Nm−1 and the damping constant b is 40gs−1 Calculate
(a) The period of oscillation
(b) Time taken for its amplitude of vibrations to drop to half of its initial values
(c) The time for the mechanical energy to drop to half initial values
 View Solution
View SolutionFor the damped oscillator shown in previous Figure, the mass m of the block is 400 g, k=45Nm−1 and the damping constant b is 80gs−1. Calculate .
(a) The period of osciallation ,
(b) Time taken for its amplitude of vibrations to drop to half of its initial value and
(c ) The time taken for its mechanical energy to drop to half its initial value.View Solution
Knowledge Check
- Question 1 - Select One
For a damped oscillator the mass 'm' of the block is 200 g, k = 90 N m−1 and the damping constant b is 40 g s−1. Calculate the period of oscillation.
A0.2B0.3C0.5D0.1 - Question 2 - Select One
For the small damping oscillator, the mass of the block is 500 g and value of spring constant is k = 50 N/m and damping constant is 10 g s^(-1) The time period of oscillation is (approx.)
A2πsB2π13sCπ5sDπ√5s - Question 3 - Select One
The angular frequency of the damped oscillator is given by ω=√km−r24m2 ,where k is the spring constant, m is the mass of the oscillator and r is the damping constant. If the ratio r2/(mk) is 8% ,the change in the time period compared to the undamped oscillator
Aincreases by 1%Bincreases by 8%Cdecreased by 1%Ddecrease
For the damped oscillator of Fig. 15.20, m= 250g, k= 85N/m, and b= 70g/s
What is the period of the motion?View SolutionIn damped oscillatory motion a block of mass 20kg is suspended to a spring of force constant 90N/m in a medium and damping constant is 40g/s. Find (a) time period of oscillation (b) time taken for amplitude of oscillation to drop to half of its intial value (c) time taken for its mechanical energy to drop to half of its initial value.
View SolutionIn the given spring block system if k=25π2Nm−1, find time period of oscillation.
 View Solution
View Solution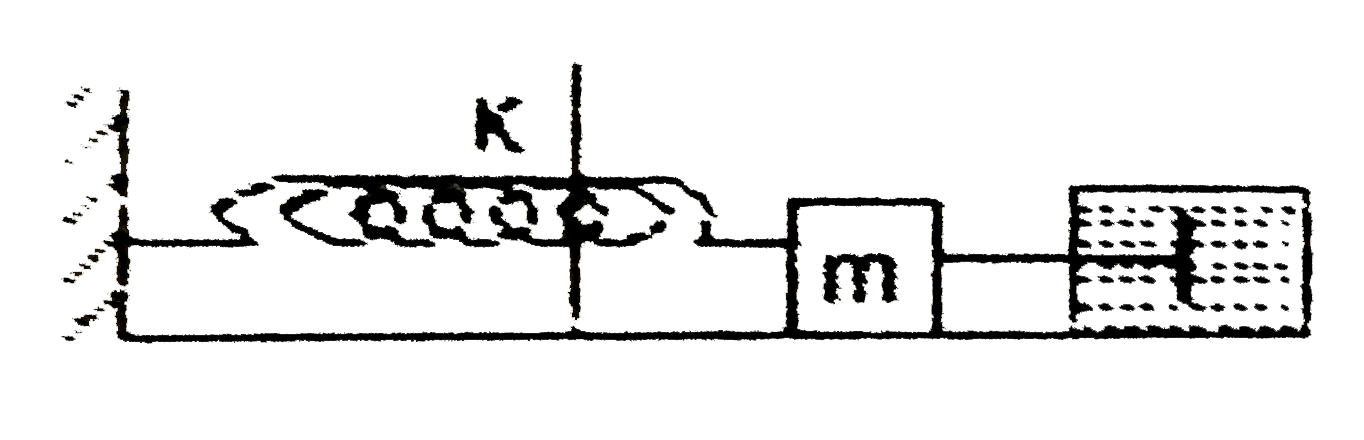
For a damped oscillator which follow the equation →F=−k→x−b→V
the mass of the block is 100 gm K=100N/M and the damping constant is 20 gm/sec. thhen find the time taken for its mechanical energy to drop to one-fourth of its initial value.View SolutionA block off mass 200 g executing SHM under the unfluence of a spring of spring constant k=90Nm−1 and a damping constant gb=40gs−1. The time elaspsed for its amplitude to drop to halff of its initial value is (Given, ln (1/2)=−0.693)
View Solution

