Doubtnut Promotions Sticky

Induced charge q can never be greater than inducing charge Q. Why ?

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

When a charged rod (inducing body) is held near one end, but the flow of electron ceases after some-time (though there is an almost inexhaustible supply of electrons in the metal sphere) because induced electrons repel movement of fresh electrons getting induced.

|
Updated on:21/07/2023
Class 12PHYSICSELECTROSTATICS

Similar Questions

Knowledge Check

  • Question 1 - Select One

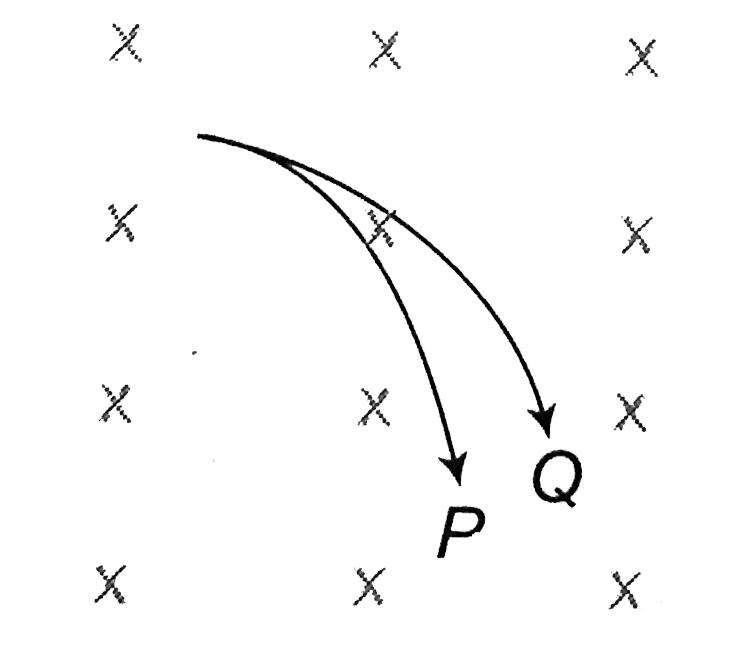
    Two charged particles P and Q enter a uniform magnetic field normally with the same speed. Their paths in the field are as shown in the figure. It can be concluded that ocr_image
    (i) the charge of P is greater than of Q
    (ii) specific charge of P is > that of Q
    (iii) both P and Q are positively charged
    (iv) both P and Q are negatively charged

    A(i), (ii)
    B(ii), (iv)
    C(ii), (iii)
    D(i), (iv)
  • Question 2 - Select One

    Statement - I : When two charged spheres are touched, then total charge is always devides equally.
    Statement - II : Induced charge always equal to inducing charge.

    AIf both Statement-I and Statement-II are true, and Statement-II is the correct explanation of Statement -I.
    BIf both Statement-I and Statement-II are true but Statement-II is not the correct explanation of Statement -I.
    CIf Statement -I is true but Statement-II is false
    DIf Statement-I is false but Statement -II is true
  • Question 3 - Select One

    Which of following result gives correct relation between charge induced (q) and inducing charge (q0) : -

    Aq0=q(1−1∈r)
    Bq=q0(1−1∈r)
    Cq+q0=1∈r
    Dq=q0(1−∈r)