In a uniform electric field if a charge is fired in a direction different from the line of electric field then the trajectory of the charge will be a
Straight line
Circle
Parabola
Ellipse
The correct Answer is:C
To determine the trajectory of a charge moving in a uniform electric field at an angle to the field lines, we can break down the problem step by step.
Step 1: Understand the Setup
We have a uniform electric field E directed along the x-axis. A charge q with mass m is fired with an initial velocity v at an angle θ to the x-axis.
Step 2: Break Down the Initial Velocity
The initial velocity v can be resolved into two components:
- The x-component (along the electric field):
ux=vcosθ
- The y-component (perpendicular to the electric field):
uy=vsinθ
Step 3: Determine the Accelerations
The charge experiences an acceleration due to the electric field:
- The acceleration in the x-direction (due to the electric field):
ax=qEm
- The acceleration in the y-direction is zero because there are no forces acting in that direction:
ay=0
Step 4: Write the Equations of Motion
Using the equations of motion, we can express the position of the charge in both the x and y directions as functions of time t:
- For the x-direction:
x=uxt+12axt2
Substituting for ux and ax:
x=(vcosθ)t+12(qEm)t2
- For the y-direction:
y=uyt+12ayt2
Since ay=0:
y=(vsinθ)t
Step 5: Eliminate Time to Find the Trajectory
To find the relationship between x and y, we need to eliminate t from the equations. From the y-equation, we can express t:
t=yvsinθ
Substituting this expression for t into the x-equation:
x=(vcosθ)(yvsinθ)+12(qEm)(yvsinθ)2
Simplifying this gives:
x=cosθsinθy+12(qEm)y2(v2sin2θ)
This is a quadratic equation in y, which can be expressed as:
y=ax+by2
where a and b are constants derived from the coefficients.
Conclusion
The resulting equation indicates that the trajectory of the charge is a parabola.
We have a uniform electric field E directed along the x-axis. A charge q with mass m is fired with an initial velocity v at an angle θ to the x-axis.
The initial velocity v can be resolved into two components:
- The x-component (along the electric field):
ux=vcosθ
- The y-component (perpendicular to the electric field):
uy=vsinθ
The charge experiences an acceleration due to the electric field:
- The acceleration in the x-direction (due to the electric field):
ax=qEm
- The acceleration in the y-direction is zero because there are no forces acting in that direction:
ay=0
Using the equations of motion, we can express the position of the charge in both the x and y directions as functions of time t:
- For the x-direction:
x=uxt+12axt2
Substituting for ux and ax:
x=(vcosθ)t+12(qEm)t2
y=uyt+12ayt2
Since ay=0:
y=(vsinθ)t
To find the relationship between x and y, we need to eliminate t from the equations. From the y-equation, we can express t:
t=yvsinθ
Substituting this expression for t into the x-equation:
x=(vcosθ)(yvsinθ)+12(qEm)(yvsinθ)2
Simplifying this gives:
x=cosθsinθy+12(qEm)y2(v2sin2θ)
This is a quadratic equation in y, which can be expressed as:
y=ax+by2
where a and b are constants derived from the coefficients.
The resulting equation indicates that the trajectory of the charge is a parabola.
Similar Questions
Knowledge Check
- Question 1 - Select One or More
When a negative charge moves in a direction opposite to the direction of an electric field,
AThe field does work on the chargeBThe charge does work on the fieldCThe charge gains potential energyDThe charge loses potential energy - Question 2 - Select One
A negative charge is moved by an external agent in the direction of electric field. Then
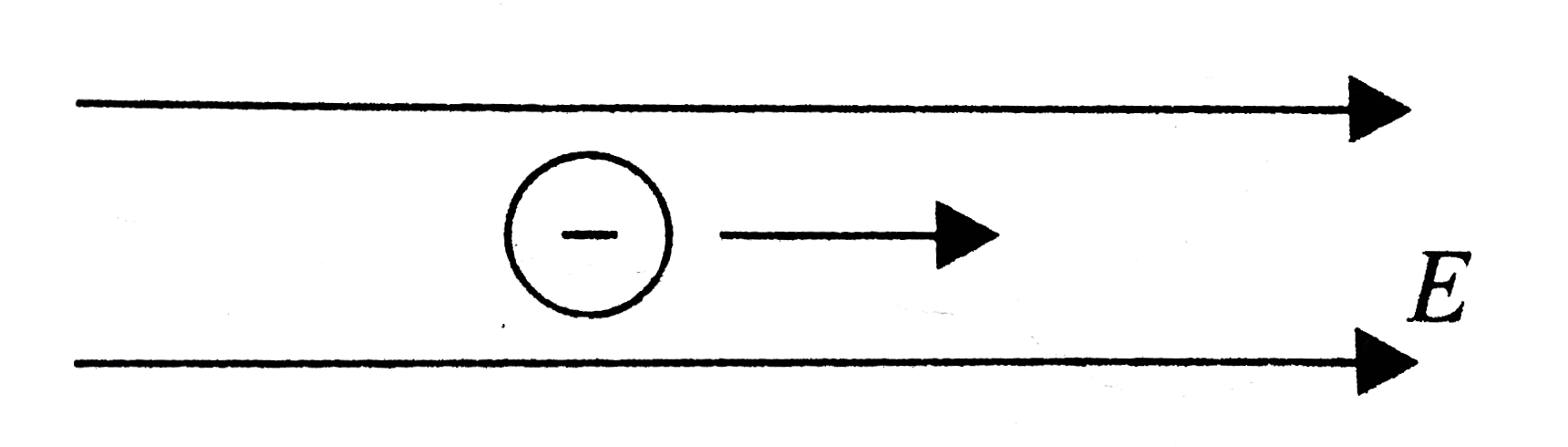 .APotential energy of the charge increases.BPotential energy of the charge decreases.CPositive work is done by the electric fieldDnegative work is done by the electric field
.APotential energy of the charge increases.BPotential energy of the charge decreases.CPositive work is done by the electric fieldDnegative work is done by the electric field - Question 3 - Select One
A small point mass carrying some positive charge on it, is released from the edge of a table. There is a uniform electric field in this region in the horizontal direction. Which of the following options then correctly describe the trajectory of the mass? (Curves are drawn schematically and are not to scale).
 A
A B
B C
C D
D
Electric field due to point charge | Electric field lines|Force on a charge in uniform electric field
View SolutionA uniform electric field E exists in a region of space. A charged particle is projected in the plane of electric field making an angle 0 with the direction of field. Is it possible to make the particle move in a straight line by applying a uniform magnetic field?
View SolutionA Point Charge In An Electric Field
View SolutionElectric lines of force about negative point charge are
View SolutionA charged particle is projected perpendicular to the direction of uniform electric field. It's path in the region of the field will be
View Solution

