An optical fibre having core of refractive index √3 and cladding of refractive index 1.5 is kept in air. The maximum angle of acceptance is
60∘
45∘
30∘
15∘
The correct Answer is:A
To find the maximum angle of acceptance (θA) for the optical fiber, we can use the formula:
sin(θA)=√n21−n22
where:
- n1 is the refractive index of the core,
- n2 is the refractive index of the cladding.
Step 1: Identify the given values
- The refractive index of the core, n1=√3
- The refractive index of the cladding, n2=1.5
Step 2: Square the refractive indices
Calculate n21 and n22:
- n21=(√3)2=3
- n22=(1.5)2=2.25
Step 3: Substitute into the formula
Now substitute these values into the formula for sine:
sin(θA)=√n21−n22=√3−2.25
Step 4: Calculate the difference
Calculate 3−2.25:
3−2.25=0.75
Step 5: Find the square root
Now find the square root:
sin(θA)=√0.75
Step 6: Simplify the square root
We can express 0.75 as:
0.75=34so√0.75=√34=√32
Step 7: Find the angle
Now, we need to find θA:
θA=sin−1(√32)
Step 8: Determine the angle
From trigonometric values, we know:
sin(60∘)=√32
Thus,
θA=60∘
Final Answer
The maximum angle of acceptance is 60∘.
---
- n1 is the refractive index of the core,
- n2 is the refractive index of the cladding.
- The refractive index of the core, n1=√3
- The refractive index of the cladding, n2=1.5
Calculate n21 and n22:
- n21=(√3)2=3
- n22=(1.5)2=2.25
Now substitute these values into the formula for sine:
sin(θA)=√n21−n22=√3−2.25
Calculate 3−2.25:
3−2.25=0.75
Now find the square root:
sin(θA)=√0.75
We can express 0.75 as:
0.75=34so√0.75=√34=√32
Now, we need to find θA:
θA=sin−1(√32)
From trigonometric values, we know:
sin(60∘)=√32
Thus,
θA=60∘
The maximum angle of acceptance is 60∘.
Similar Questions
High refractive index : cool air :: low refractive index : ____
View SolutionA fibre optic cable has a transparent core of refractive index 1.6 and the cladding has a refractive index of 1.5. An optical signal travels along path A and another signal travels along path B such that it strikes the core – cladding interface at an angle of incidence θ that is just greater than the critical angle. Length of the cable is 1500 m.
(a) Find the time difference between the two signals reaching the other end of the cable.
(b) A digital signal shown in the Figure. is transmitted through the cable. Find maximum frequency so that the crest from path A never arrives with a trough from path B at the receiving end of the cable.
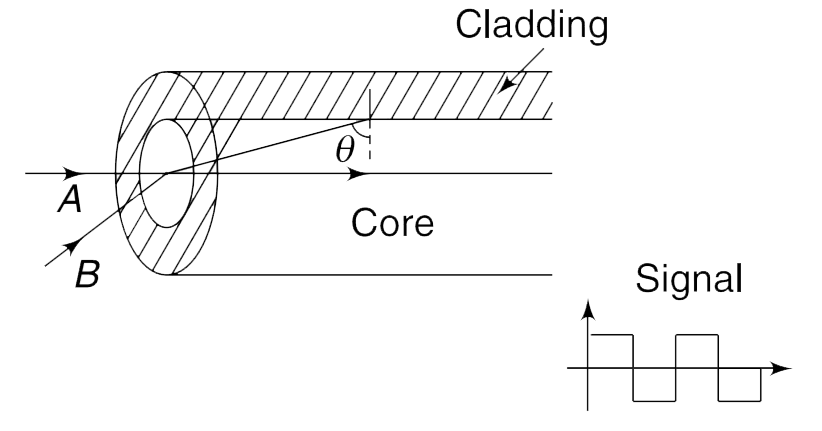
View Solution
Knowledge Check
In optical fibre refractive index of core is
Aless than R.I of claddingBmore than R.I of claddingCequal to R.I. of claddingDhalf of R.I. of claddingIn opticle fibres,the refractive index of the core is
Agreater than that of the claddingBequal to that of the claddingCsmaller than that of the claddingDindependent of that of the claddingRefractive index is maximum for
AglassBwaterCairDdiamond
A silicon optical fibre with a core diameter large enough has a core refractive index of 1.50 and a cladding refrcative index 1.47.Determine(i)the critical angle at the core cladding interface.(ii)the numerical aperture for the fibre.(iii)the acceptance angle in air for the fibre.
View SolutionA silicon optical fibre with a core diameter large enough has a core refractive index of 1.50 and a cladding refrcative index 1.47.Determine(i)the critical angle at the core cladding interface.(ii)the numerical aperture for the fibre.(iii)the acceptance angle in air for the fibre.
View SolutionThe glass of optical fibre has a refractive index 1.55 and cladding with another glass of refractive index 1.51. When the surrounding medium is air, the numerical aperture will be
View SolutionIn an optical fibre ,the refractive index of the core material is
View SolutionFor an optical fibre ,the ratio of the refractive index of the core to the refractive index of the cladding is 1.25 . What is the critical angle at the core cladding interface ?
View Solution
