The cost of 2kg of apples and 1kg of grapes on a day was found to be Rs 160. After a month the cost of 4kg of apples and 2kg of grapes is Rs300. Represent the situation algebrically and geometrically.Solution in Kannada
Step by step video & image solution for The cost of 2kg of apples and 1kg of grapes on a day was found to be Rs 160. After a month the cost of 4kg of apples and 2kg of grapes is Rs300. Represent the situation algebrically and geometrically. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PAIR OF LINEAR EQUATION IN TWO VARIABLES BOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - PAIR OF LINEAR EQUATION IN TWO VARIABLES EXERCISE - EXERCISE 3.2
18 Videos
- PAIR OF LINEAR EQUATION IN TWO VARIABLES BOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - PAIR OF LINEAR EQUATION IN TWO VARIABLES EXERCISE - EXERCISE 3.3
13 Videos
- MOST LIKELY QUESTION PAPER 8 BOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - MOST LIKELY QUESTION PAPER 8 EXERCISE - QUESTIONS
33 Videos
- POLYNOMIALSBOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - POLYNOMIALSEXERCISE - Exercise 9.4
5 Videos
Similar Questions
From the pair of linear equations in the following and problems, and find their solutions graphically.
10 students, of Class X took part in a Mathematics quiz. If the number of girs is 4 more than the number of boys, find the number of boys and girls who took part in the quiz.
View SolutionSolve the following pair of linear equations by the substitution method.
x+y=14
x−y=4
View Solution10sec2A−10tan2A=_______
View Solution(i) Verify that the numbers given alongside of the cubic polynomials below are their zeroes. Also verify the relationship between the zeroes and the coefficients in this case:
2x2+x2−5x+2,12,1,−2
(ii) Verify that the numbers given alongside of the cubic polynomials below are their zeroes. Also verify the relationship between the zeroes and the coefficients in this case:
x3−4x2+5x−2,2,1,1
View SolutionThe cost of 2 kg of apples and 1kg of grapes on a day was found to be Rs 160. After a month, the cost of 4 kg of apples and 2 kg of grapes is Rs 300. Represent the situation algebraically and geometrically.
View SolutionThe cost of 2kg of apples and 1 kg of grapes on a day was found to be Rs 160. After a month, the cost of 4 kg of apples and 2 kg of grapes is Rs 300. Represent the situation algebraically and geometrically.
View Solution2 किग्रा सेब और किग्रा अंगूर का मूल्य किसी दिन रु160 था । एक महीने बाद 4 किग्रा सेब और 2 किग्रा अंगूर का मूल्य रु300 हो जाता है । इस स्थिति को बीजगणितीय तथा ज्यामितीय रूपों में व्यक्त कीजिए ।
View Solutionकिग्रा सेब और 1 किग्रा अंगूर का मूल्य किसी दिन ₹ 160 था । एक महीने बाद 4 किग्रा सेब और 2 किग्रा अंगूर का मूल्य ₹ 300 हो जाता है । इस स्थिति को बीजणितीय तथा ज्यामितीय रूपों में व्यव कीजिए ।
View Solutionकिसी दिन 2 किलो सेब और 1 किलो अंगूर का मूल्य 160 रु था। एक महीने बाद 4 किलो सेब तथा 2 किलो अंगूर का मूल्य 300 रु हो जाता है। इस स्थिति को बीजगणितीय तथा ज्यामितीय रूपों में व्यक्त कीजिए।
View Solution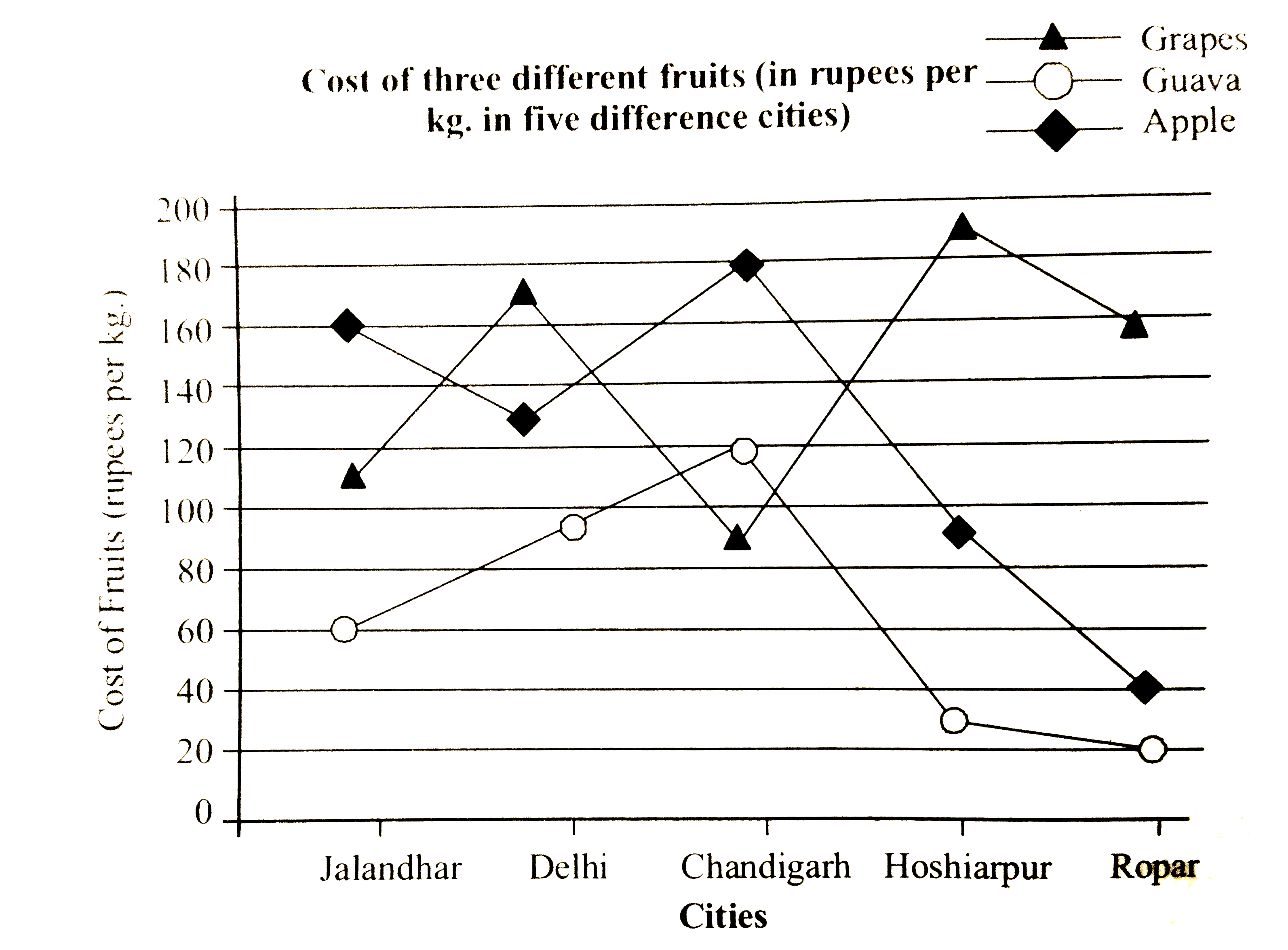
What is the respective ratio between the cost of one kg of apples from Ropar and the cost of one kg of grapes from Chandigarh ?
View SolutionThe cost of 2kg onion 4kg of wheat and 3kg of rice Is Rs.280 .The cost of 1kg of onion 3kg of wheat and "4kg" of rice is Rs 270.The cost of 3kg of onion,2kg of wheat and 1kg of rice is Rs.160 .Find the cost of each item per kg by matrix method.
View SolutionThe price of 2kg apples and 1kg of grapes a day was found to be Rs.160. A month later the price of 4kg apples and 2kg grapes was Rs.300. Represent the situation algebraically and geometrically.
View SolutionGrape, apple _____
View SolutionThe cost of 2 kg of apples and 1 kg of grapes on a day was found to be Rs. 160. After a month, the cost of 4 kg of apples and 2 kg of grapes is Rs. 300. Represent the situation algebraically.
View SolutionThe cost of 2 kg of apples and 1 kg of grapes on a day was found to be Rs. 160. After a month, the coast of 4 kg of apples and 2 kg of grapes is Rs. 300. Represent the situation algebraically and geometrically.
View Solution
