Activation energy (Ea) and rate constants (k1andk2) of a chemical reaction at two different temperature (T1andT2) are related by
In k2k1=−EaR(1T1−1T2)
In k2k1=−EaR(1T2−1T1)
In k2k1=−EaR(1T2+1T1)
In k2k1=EaR(1T1−1T2)
The correct Answer is:B, D
To derive the relationship between the activation energy (Ea) and the rate constants (k1andk2) of a chemical reaction at two different temperatures (T1andT2), we will use the Arrhenius equation. Here’s a step-by-step solution:
Step 1: Write the Arrhenius Equation
The Arrhenius equation relates the rate constant k to the temperature T and the activation energy Ea:
\(
k = A e^{-\frac{E_a}{RT}}
\)
where:
- \( k \) = rate constant
- \( A \) = Arrhenius constant (frequency factor)
- \( E_a \) = activation energy
- \( R \) = universal gas constant
- \( T \) = temperature in Kelvin
Step 2: Write the Equations for Two Different Temperatures
For two different temperatures \( T_1 \) and \( T_2 \), we can write:
\(
k_1 = A e^{-\frac{E_a}{RT_1}} \quad \text{(1)}
\)
\(
k_2 = A e^{-\frac{E_a}{RT_2}} \quad \text{(2)}
\)
Step 3: Take Natural Logarithm of Both Equations
Taking the natural logarithm of both equations, we have:
\(
\ln k_1 = \ln A - \frac{E_a}{RT_1} \quad \text{(3)}
\)
\(
\ln k_2 = \ln A - \frac{E_a}{RT_2} \quad \text{(4)}
\)
Step 4: Subtract the Two Equations
Now, we subtract equation (4) from equation (3):
\(
\ln k_1 - \ln k_2 = \left(\ln A - \frac{E_a}{RT_1}\right) - \left(\ln A - \frac{E_a}{RT_2}\right)
\)
This simplifies to:
\(
\ln k_1 - \ln k_2 = -\frac{E_a}{RT_1} + \frac{E_a}{RT_2}
\)
Step 5: Simplify the Expression
Rearranging gives:
\(
\ln k_1 - \ln k_2 = E_a \left(\frac{1}{RT_2} - \frac{1}{RT_1}\right)
\)
Using the property of logarithms, we can express this as:
\(
\ln \left(\frac{k_1}{k_2}\right) = E_a \left(\frac{1}{RT_2} - \frac{1}{RT_1}\right)
\)
Step 6: Final Relation
Thus, we arrive at the final relation:
\(
\ln \left(\frac{k_1}{k_2}\right) = \frac{E_a}{R} \left(\frac{1}{T_2} - \frac{1}{T_1}\right)
\)
The Arrhenius equation relates the rate constant k to the temperature T and the activation energy Ea:
\(
k = A e^{-\frac{E_a}{RT}}
\)
where:
- \( k \) = rate constant
- \( A \) = Arrhenius constant (frequency factor)
- \( E_a \) = activation energy
- \( R \) = universal gas constant
- \( T \) = temperature in Kelvin
For two different temperatures \( T_1 \) and \( T_2 \), we can write:
\(
k_1 = A e^{-\frac{E_a}{RT_1}} \quad \text{(1)}
\)
\(
k_2 = A e^{-\frac{E_a}{RT_2}} \quad \text{(2)}
\)
Taking the natural logarithm of both equations, we have:
\(
\ln k_1 = \ln A - \frac{E_a}{RT_1} \quad \text{(3)}
\)
\(
\ln k_2 = \ln A - \frac{E_a}{RT_2} \quad \text{(4)}
\)
Now, we subtract equation (4) from equation (3):
\(
\ln k_1 - \ln k_2 = \left(\ln A - \frac{E_a}{RT_1}\right) - \left(\ln A - \frac{E_a}{RT_2}\right)
\)
This simplifies to:
\(
\ln k_1 - \ln k_2 = -\frac{E_a}{RT_1} + \frac{E_a}{RT_2}
\)
Rearranging gives:
\(
\ln k_1 - \ln k_2 = E_a \left(\frac{1}{RT_2} - \frac{1}{RT_1}\right)
\)
Using the property of logarithms, we can express this as:
\(
\ln \left(\frac{k_1}{k_2}\right) = E_a \left(\frac{1}{RT_2} - \frac{1}{RT_1}\right)
\)
Thus, we arrive at the final relation:
\(
\ln \left(\frac{k_1}{k_2}\right) = \frac{E_a}{R} \left(\frac{1}{T_2} - \frac{1}{T_1}\right)
\)
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - AAKASH INSTITUTECHAPTER - CHEMICAL KINETICSEXERCISE - ASSIGNMENT (SECTION D : Assertion - Reason Type Questions)
15 Videos
- CHEMICAL KINETICSBOOK - AAKASH INSTITUTECHAPTER - CHEMICAL KINETICSEXERCISE - ASSIGNMENT (SECTION B : Objective Type Questions)
20 Videos
- CHEMICAL BONDING AND MOLECULAR STRUCTURE BOOK - AAKASH INSTITUTECHAPTER - CHEMICAL BONDING AND MOLECULAR STRUCTURE EXERCISE - Assignment Section J (Aakash Challengers Questions)
10 Videos
- CHEMISTRY IN EVERYDAY LIFE BOOK - AAKASH INSTITUTECHAPTER - CHEMISTRY IN EVERYDAY LIFE EXERCISE - Assignment ( SECTION - A)
45 Videos
Similar Questions
Knowledge Check
Activation energy (Ea) and rate constants (k1 and k2) of a chemical reaction at two different temperatures (T1 and T2) are related by
AIn k2k1=−EaR(1T1−1T2)BIn k2k1=−EaR(1T2−1T1)CIn k2k1=−EaR(1T1+1T2)DIn k2k1=EaR(1T1−1T2)Activation energy (Ea) and rate constant (k1andk2) of chemical reaction at two different temperatures (T1andT2) are related by :
AInk2k1=EaR[1T1−1T2]BInk2k1=EaR[1T2−1T1]CInk2k1=EaR[1T2+1T1]DInk2k1=EaR[1T1+1T2]Activation energy (Ea) and rate constant (K1 and (K2) for a chemical reaction at two different temperatures T1 and T2 are related by:
AIn K2K1=EaR(1T1−1T2)BIn k2k1=−EaR(1T2−1T1)CIn k2k1=−EaR(1T2+1T1)DIn k2k1=EaR(1T1−1T2)
Activation energy (Ea) and rate constants (k1 and k2) of a chemical reaction at two different temperatures (T1 and T2) are related by
View SolutionA colliison between reactant molecules must occur with a certain minimum energy before it is effective in yielding Product molecules. This minimum energy is called activation energy Ea Large the value of activation energy, smaller the value of rate constant k. Larger is the value of activation energy, greater is the effect of temperature rise on rate constant k.
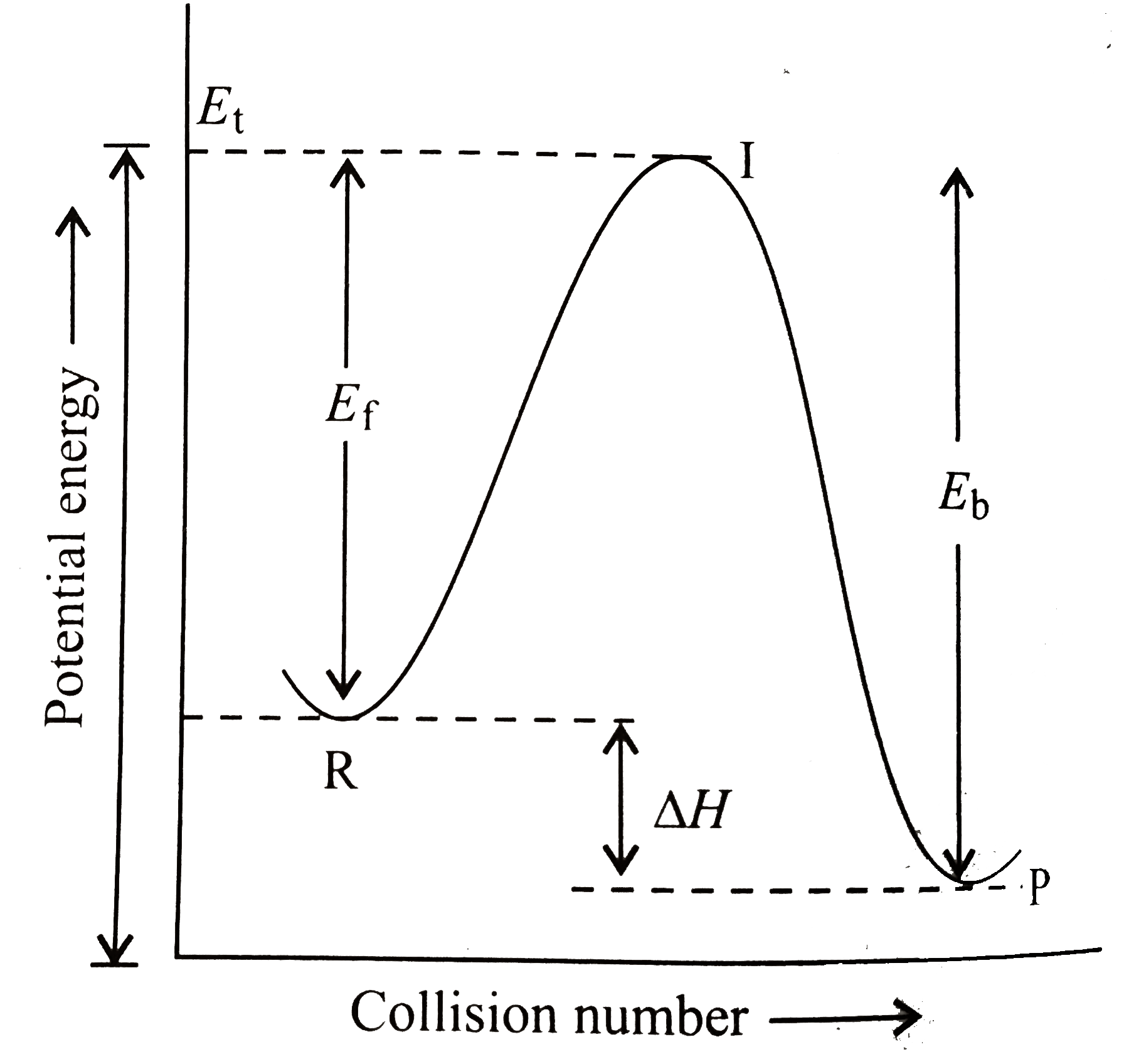
Ef= Activation energy of forward reaction
Eb= Activation energy of backward reaction
ΔH=Ef−Eb
Ef= threshold energy
For two reactions, activation energies are Ea1 and Ea2, rate constant are k1 and k2 at the same temperature. If k1>k2, then
View SolutionThe rate constant K1 of a reaction is found to be double that of rate constant K2 of another reaction. The relationship between corresponding activation energies of the two reaction at same temperature (E1andE2) can be represented as:
View SolutionSTATEMENT-1 : Fraction of total molecules having energy equal to or greater than activation energy =K/A=e−Ea/RT
and
STATEMENT-2 : Activation energy of a chemical reaction is always constant at a given temperature.
View SolutionA reaction can take place by two paths, k1andk2 are rate constants for the two paths & E1andE2 are their respective activation energies.
At temperature Ta:k1>k2,E1<E2.
if temperature is raised to Tb, the rate constants change to k1 & k2 Which relation is correct between k1,k2,k1 & k2 (considering activation energy does not change with temperature )
View Solution
