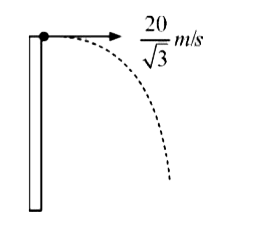
A particle is projected horizontally with speed 20√3m/s, from some height at t=0. At what time its velocity will make 60∘ angle with initial velocity ?
1 sec
2 sec
1.5 sec
2.5 sec
The correct Answer is:B
To solve the problem, we need to determine the time at which the velocity of a horizontally projected particle makes a 60-degree angle with its initial velocity.
1. Identify the Initial Conditions:
- The particle is projected horizontally with an initial speed u=20√3m/s.
- The initial vertical velocity uy=0m/s since the projection is horizontal.
2. Understanding the Velocity Components:
- The horizontal component of the velocity Vx remains constant throughout the motion:
Vx=u=20√3m/s
- The vertical component of the velocity Vy at time t can be calculated using the formula:
Vy=gt
where g is the acceleration due to gravity, approximately 10m/s2.
3. Setting Up the Angle Condition:
- We need to find the time t when the resultant velocity V makes a 60∘ angle with the horizontal. The angle condition can be expressed using the tangent function:
tan(60∘)=VyVx
- Since tan(60∘)=√3, we have:
√3=VyVx
4. Substituting the Velocity Components:
- Substitute Vy and Vx:
√3=gt20√3
- Rearranging gives:
√3⋅20√3=gt
- Simplifying:
20=10t
5. Solving for Time:
- From the equation 20=10t:
t=2010=2seconds
Final Answer:
The time at which the velocity of the particle makes a 60∘ angle with the initial velocity is t=2seconds.
---
- The particle is projected horizontally with an initial speed u=20√3m/s.
- The initial vertical velocity uy=0m/s since the projection is horizontal.
- The horizontal component of the velocity Vx remains constant throughout the motion:
Vx=u=20√3m/s
- The vertical component of the velocity Vy at time t can be calculated using the formula:
Vy=gt
where g is the acceleration due to gravity, approximately 10m/s2.
- We need to find the time t when the resultant velocity V makes a 60∘ angle with the horizontal. The angle condition can be expressed using the tangent function:
tan(60∘)=VyVx
- Since tan(60∘)=√3, we have:
√3=VyVx
- Substitute Vy and Vx:
√3=gt20√3
- Rearranging gives:
√3⋅20√3=gt
- Simplifying:
20=10t
- From the equation 20=10t:
t=2010=2seconds
The time at which the velocity of the particle makes a 60∘ angle with the initial velocity is t=2seconds.
Similar Questions
A particle is projected horizontally with a speed of 40√3m/s, from some height t=0. At what time its velocity makes 60∘ angle with initial velocity.
(g=10m/s2)
 View Solution
View SolutionA particle is projected from the ground at an angle of 60∘ with the horizontal with a speed by 20 m/s. The radius of curvature of the path of the particle, when its velocity makes an angle of 30∘ with horizontal is 809.√xm Find x .
View Solution
Knowledge Check
- Question 1 - Select One
A particle is projected horizontally will speed 20ms−1 from the top of a tower. After what time velocity of particle will be at 45∘ angle from initial direction of projection.
A1sB2sC3sD4s - Question 2 - Select One
Two particles are projected horizontally with same speed 30m/s but in opposite directions from same height at time t=0. Calculate angle between velocity vectors of particles at time t=3s.
A0∘B30∘C60∘D90∘ - Question 3 - Select One or More
A particle is projected from ground with velocity 40√2m/s at 45∘. At time t=2s
Adisplacement of particle is 100mBvertical component of velocity is 20m/sCvelocity makes an angle tan−1(2) with verticalDparticle is at height of 60m from ground
A particle is projected horizontally with a speed V=5m/s from the top of a plane inclined at an angle θ=37∘ to the horizontal (g=10m/s2)
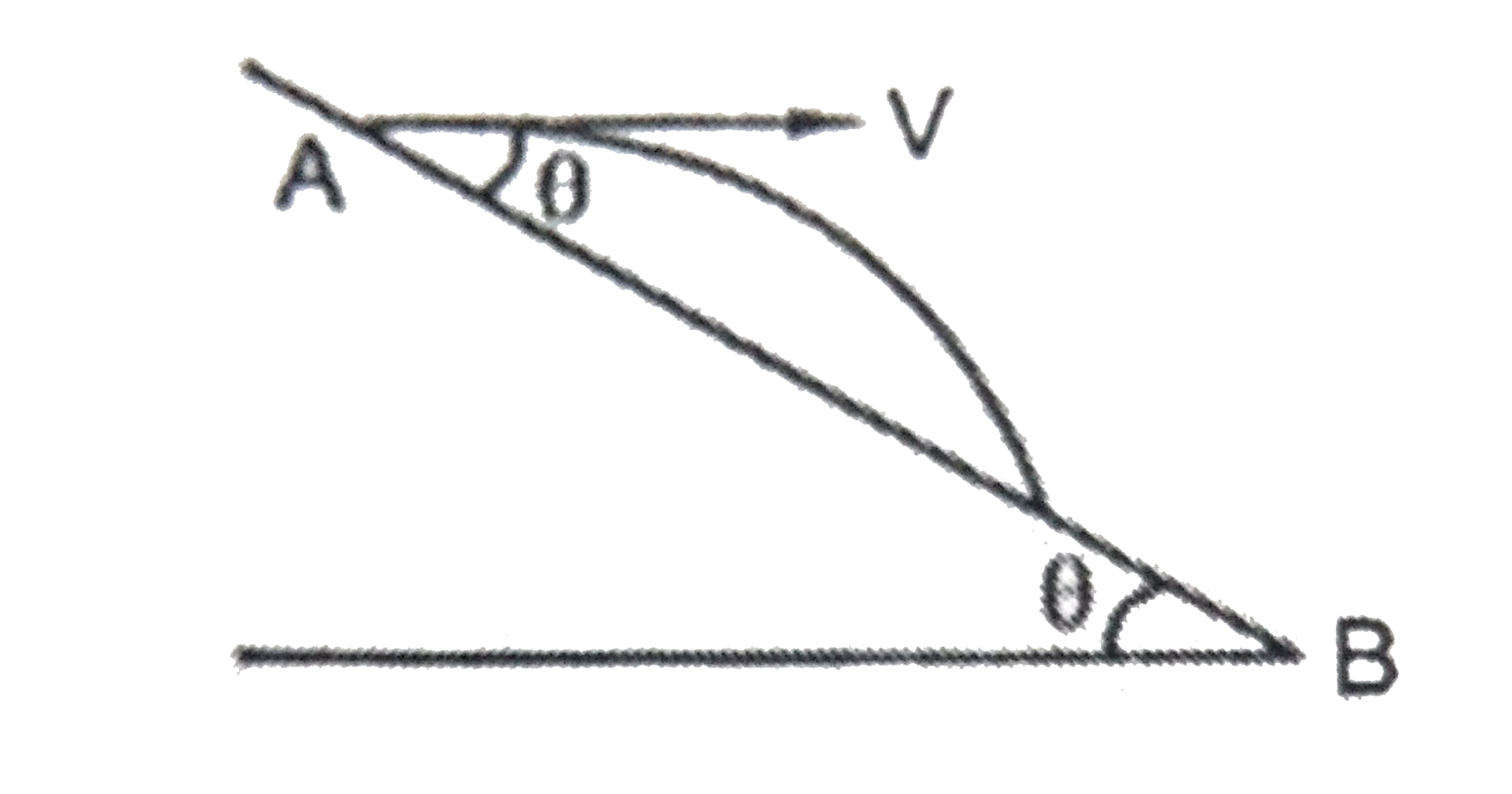
What is the velocity of the particle just before it hits the plane?View SolutionA particle is projected from ground with velocity 40√2m at 45∘.At time t=2s
View SolutionA particle is projected at an angle of 60∘ above the horizontal with a speed of 10m/s. After some time the direction of its velocity makes an angle of 30∘ above the horizontal. The speed of the particle at this instant is s
View SolutionIn the above question what will be the displacement of particle in x-direction when its velocity makes 60∘ angle with initial velocity?
 View Solution
View SolutionA particle is projected with velocity 50 m/s at an angle 60∘ with the horizontal from the ground. The time after which its velocity will make an angle 45∘ with the horizontal is
View Solution

