If and are roots of then is : Solution in Tamil
44
The correct Answer is:B
Step by step video, text & image solution for If alpha and beta are roots of x^(2)+8x+10=0 then (alpha)/(beta)+(beta)/(alpha) is : by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ALGEBRABook:PREMIERS PUBLISHERSChapter:ALGEBRAExercise:OTHER IMPORTANT OBJECTIVE TYPE QUESTIONS (Match the following)
Explore 4 Videos
- ALGEBRABook:PREMIERS PUBLISHERSChapter:ALGEBRAExercise:SOLUTION TO ACTIVITIES
Explore 7 Videos
- CO-ORDINATE GEOMETRY Book:PREMIERS PUBLISHERSChapter:CO-ORDINATE GEOMETRY Exercise:Other Important Objective
Explore 25 Videos
Similar Questions
x4−8x2+k is a perfect square then k is
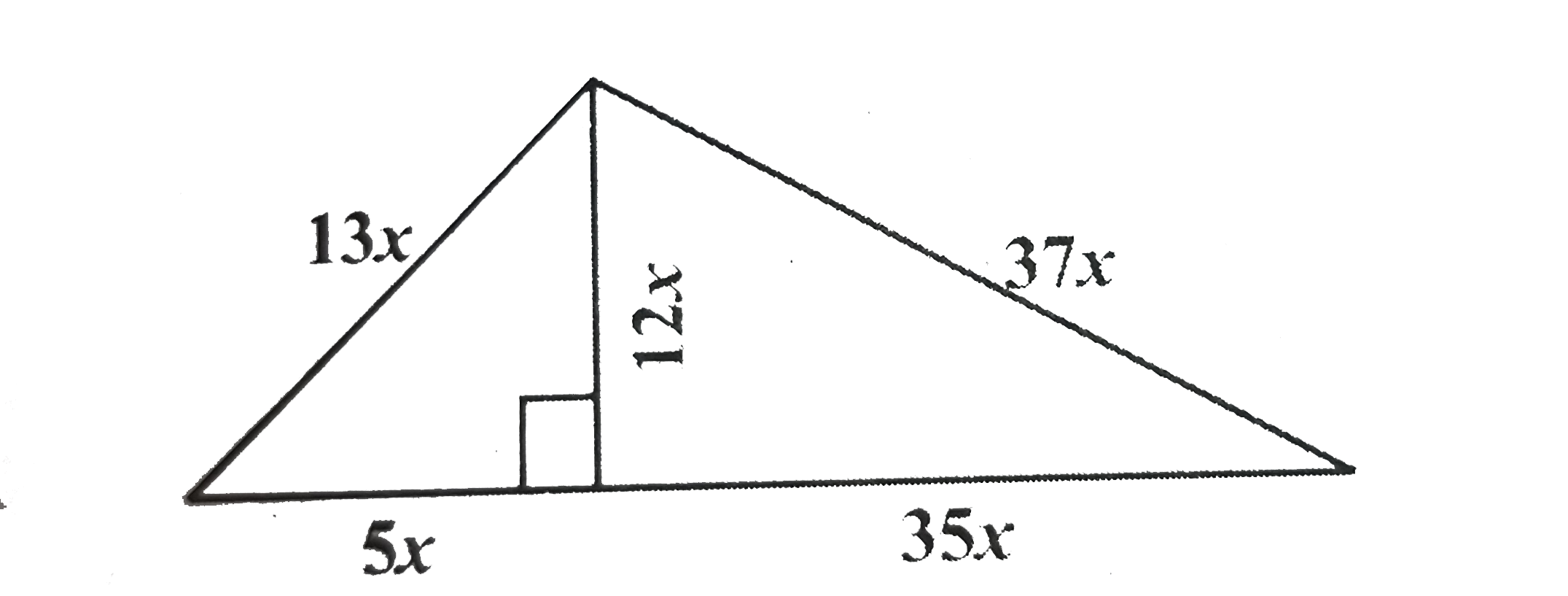
View SolutionFind the ratio of the perimeter to the area of the given triangle.
View SolutionThe area of the triangle formed by the points (0,0),(3,0) and (0,4) is :
View SolutionIf alpha,beta are the roots of 2x^(2)+x+7=0 find the value of alpha^(2)beta+alpha beta^(2)
View SolutionIf alpha,beta are the roots of ax^(2)+bx+c-0 then alpha beta^(2)+alpha^(2)beta+alpha beta=
View SolutionIf alpha,beta are the roots of x^(2)-px+q=0 then the equation whose roots are alpha beta+alpha+beta,alpha beta-alpha-beta
View SolutionIf α,β are roots of the equation x2+x+1=0, then the equation whose roots are αβandβα, is
View SolutionIF α,β are the roots of x2−x+2=0 then α2β+αβ2 =
View SolutionIf alpha , beta are the roots of ax^2 + bx + c=0 then alpha beta ^(2) + alpha ^2 beta + alpha beta =
View SolutionIF α,β are the roots of x2−ax+b=0 , then the whose roots are α+βα,α+ββ is
View SolutionIf the roots of
x4−10x3+37x2−60x+36=0 are α,α,β,β(α<β), then 2α+3β−2αβ=
View SolutionIf (α,β) are the roots of the equation 15x2−22x+8=0and(α',β') are the roots of the equation 8x2−22x+15=0, then the equation of the circle on A(α,α')andB(β,β') as diameter is
View SolutionIf α,β,γ are the roots of ax3+bx2+cx+d=0 and ∣∣ ∣ ∣∣αβγβγαγαβ∣∣ ∣ ∣∣=0,α≠β≠γ then find the equation whose roots are α+β−γ,β+γ−α, and γ+α−β.
View SolutionIf α,β are the roots of the equation x2+x+1=0, then the equation whose roots are αβ and βα is
View SolutionIf α,β are the roots of the quadratic equation x2+bx−c=0, the equation whose roots are b and c, is a. x2+αx−β=0 b. x2−[(α+β)+αβ]x−αβ(α+β)=0 c. x2+[(α+β)+αβ]x+αβ(α+β)=0 d. x2+[(α+β)+αβ)]x−αβ(α+β)=0
View Solution