[Tamil] A salesperson whose annual earnings can be represented by the

Let A and B be two sets such that n (A) = 3 and n(B) =2. If (x,1) (y,2) (z,1) are in $A \times B$ , find A and B, where x,y,z are distinct elements.

View Solution

For the curve y = $x^{3}$ given in figure draw,
y = - $x^{3}$

View Solution

State whether the following sets are finite or infinite.
(iv) { $x \in R : x$ is a rational number}.

View Solution

If $a_{i j} = i^{2} - j$ and $A = {(a_{i j})}_{2 \times 2}$ is:

View Solution

$\frac{1}{{cos 80}^{o}} - \frac{\sqrt{3}}{{sin 80}^{o}}$ =

View Solution

Manufacturer can sell x items at a price of rupees $(5 - \frac{x}{100})$ each. The cost price of x items is Rs $(\frac{x}{5} + 500)$ . Find the number of items he should sell to earn maximum profit

View Solution

A manufacturer can sell x items at a price of Rs. $(5 - \frac{x}{100})$ each. The cost price of $x$ items is Rs. $(\frac{x}{5} + 500) .$ Find the number of items he should sell to earn maximum profit.

View Solution

A salesperson whose annual earnings can be represented by the function A (x) = 30,000+0.04x, where x is the rupee value of the merchandise he sells. His son is also in sales and his earnings are represented by the function S(x) = 25,000 +0.05 x. Find (A+S) (x) and determine the total family income if they each sell Rs 1,50,00,000 worth of merchandise.

View Solution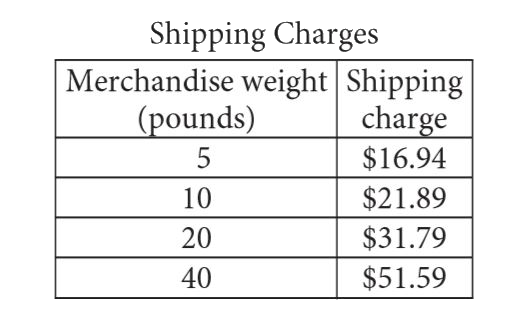

ocr_image
The table above shows shipping charges for an online retailer that sells sporting goods. There is a linear relationship between the shipping charge and the weight of the merchandise. Which function can be used to determine the total shipping charge f (x) , in dollars, for an order with a merchandise weight of x pounds?

View Solution

Manufacturer can sell x items at a price of Rs. $(5 - \frac{x}{100})$ each . The cost price is Rs. $(\frac{x}{5} + 500)$ . Find the number of items he should sell to earn maximum profit.

View Solution

ஒரு விற்பனை பிரதிநிதியின் ஆண்டு வருமானத்தைக் குறிக்கும் சார்பு $A (x) = 30000 + 0.04 x$ .இங்கு $x$ என்பது அவர் விற்கும் பொருளின் விலைமதிப்பை ரூபாயகக் குறிக்கின்றது. விற்பனைத் துறையில் உள்ள அவர் மகனின் வருமானம் $S (x) = 25, 000 + 0.05 x$ எனும் சார்பாகக் குறிக்கப்படுகிறது எனில், $(A + S) (x)$ காண்க. மேலும் $1, 50, 00, 000$ மதிப்புள்ள பொருட்களை அவர்களிருவரும் தனித்தனியே விற்றால் வருமானம்

View Solution

Manufacturer can sell x items at a price of rupees $(5 - \frac{x}{100})$ each. Thecost price of x items is Rs $(\frac{x}{5} + 500)$ . Find the number of items he should sell to earn maximum profit.

View Solution

Manufacturer can sell x items at a price of rupees $(5 - \frac{x}{100})$ each. Thecost price of x items is Rs $(\frac{x}{5} + 500)$ . Find the number of items he should sell to earn maximum profit.

View Solution

Manufacturer can sell x items at a price of rupees $(5 - \frac{x}{100})$ each. The cost price of x items is Rs. $(\frac{x}{5} + 500)$ . Find the number of items he should sell to earn maximum prodit.

View Solution

ਕਿਸੇ ਉਤਪਾਦ ਦੀਆਂ x ਇਕਾਈਆਂ ਦੇ ਵੇਚਣ ਤੇ ਪ੍ਰਾਪਤ ਕੁੱਲ ਆਮਦਨ $R (x)$ ਰੁਪਏ ਵਿੱਚ $R (x) = 13 x^{2} + 26 x + 15$ ਨਾਲ ਦਰਸਾਈ ਗਈ ਹੈ। ਸੀਮਾਂਤ ਆਮਦਨ ਪਤਾ ਕਰੋ ਜਦੋਂ x = 7 ਹੈ।

View Solution

ਇੱਕ ਉਤਪਾਦ ਦੀਆਂ x ਇਕਾਈਆਂ ਦੇ ਵੇਚਣ ਨਾਲ ਪ੍ਰਾਪਤ ਕੁੱਲ ਆਮਦਨ ਰੁਪਏ ਵਿੱਚ $R (x) = 3 x^{2} + 36 x + 5$ ਨਾਲ ਦਰਸਾਈ ਗਈ ਹੈ। ਜਦੋਂ x = 15 ਹੈ ਦਾਂ ਸੀਮਾਂਤ ਆਮਦਨ ਹੈ :

View Solution

Topper's Solved these Questions

Similar Questions

PREMIERS PUBLISHERS-SETS,RELATIONS AND FUNCATIONS-SOLUTION TO EXERCISE 1.3