Derive an expression for the potential energy of an elastic stretched spring. Solution in Tamil

Video Solution

Text Solution

Verified by Experts

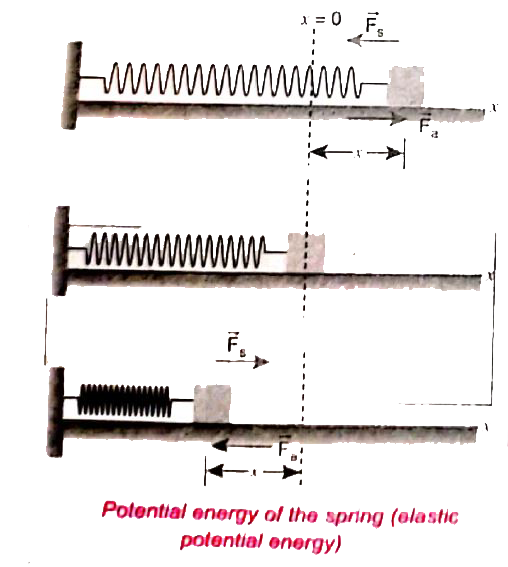
Let us consider a spring-mass system. It is assumed that a mass, m lying on a smooth horizontal table as shown in the figure. Here, $x = 0$ is the equilibrium position. One end of the spring is connected to a rigid wall and the other end to the mass.
Now an external force ${\to F}_{a}$ is applied so that it is stretched by a distance (x) in the direction of the force as long as the spring remains in equilibrium position. Its potential energy is zero.
A restoring force called spring force ${\to F}_{S}$ is developed in the spring. It tries to bring the mass back to its original position. This applied force and the spring force are equal in magnitude but opposite in direction i.e., ${\to F}_{a} = - {\to F}_{s}$ .

According to Hooke's law, the restoring force developed in the spring is given by
${\to F}_{s} = - k \to x ... (1)$
The negative sign in the above expression implies that hte spring force is always opposite to that of displacement $\to x and k$ is the force constant. Hence applied force is ${\to F}_{a} = + k \to x$ . It is implied from the positive sign, that the applied force is in the direction of displacement $\to$ . The spring force is an example of variable force as it depends on the displacement $\to x$ . Let the spring be stretched to a small distance $d \to x$ . The work done by the applied force on the spring to stretch it by a displacement x is stored as elastic potential energy.
$U = \int {\to F}_{a} . d \to r = x \int 0 ∣ ∣ {\to F}_{a} ∣ ∣ | d \to r | cos θ$
$U = x \int 0 F_{a} d x cos θ ... (2)$
The applied force ${\to F}_{a}$ and the displacement dx are in the same direction. As, the initial position is taken as the equilibrium position or mean position, $x = 0$ is the lower limit of integration.
$U = x \int 0 k x d x ... (3)$
$U = k {[\frac{x^{2}}{2}]}_{0}^{x} ... (4)$
$U = \frac{1}{2} k x^{2} ... (5)$
If the initial position is not zero, and if the mass is changed from position $x_{i}$ to $x_{f}$ , then the elastic potential energy is
$U = \frac{1}{2} k (x_{f}^{2} - x_{i}^{2}) ... (6)$
From equation (5) and (6), it is observed that the potential energy of the stretched spring depends on the force constant k and elongation or compression x.

|

Updated on:21/07/2023

Class 11 PHYSICS WORK, ENERGY AND POWER

Topper's Solved these Questions

Similar Questions

PREMIERS PUBLISHERS-WORK, ENERGY AND POWER -LONG ANSWER QUESTIONS

Home
Ask Doubt
Profile