Question

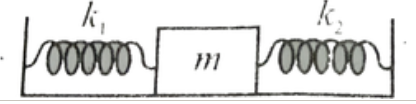
If both spring constant $k_1$ and $k_2$ are increased to $4k_1$ and $4k_2$ respectively, then new frequency in terms of original frequency f is :

Options

A. 2f
B. f
C. 4f
D. `(f)/(2)`

Answer

A

Explanation

`F=F_(1)+F_(2)=-k_(1)x-k_(2)x`
`=-(k_(1)+k_(2))x`
`K_(eff)=k_(1)+K_(2)`
When spring constant are increased four times,
`f'=(1)/(2pi)sqrt((4k_(1)+4k_(2))/(m))`
`=(1)/(2pi)sqrt(4((k_(1)+k_(2)))/(m))`
`=((1)/(2) sqrt((K_(1)+k_(2))/(m)))xx2=2f`