In Newton's second →F=m→a (for constant mass m), →a is the acceleration of the mass m with respect to
any observer
any inertial observer
an observer at rest only
an observer moving with uniform speed only
The correct Answer is:C
To solve the question regarding Newton's second law of motion, we need to analyze the statement given in the question and the options provided.
1. Understanding Newton's Second Law:
Newton's second law states that the force acting on an object is equal to the mass of that object multiplied by its acceleration, expressed mathematically as →F=m→a. Here, →F is the net force acting on the object, m is the mass, and →a is the acceleration.
2. Acceleration as a Vector:
The acceleration →a is a vector quantity, which means it has both magnitude and direction. It describes how the velocity of an object changes over time.
3. Reference Frame:
The question asks with respect to what the acceleration →a is defined. In classical mechanics, acceleration is typically measured with respect to an inertial frame of reference.
4. Inertial Frame of Reference:
An inertial frame of reference is one in which an object either remains at rest or moves at a constant velocity unless acted upon by a net external force. This means that the laws of physics, including Newton's laws, hold true in such frames.
5. Evaluating the Options:
- Option A: Any observer - Incorrect, as this does not specify the type of observer.
- Option B: Any inertial observer - This is correct, as Newton's laws apply in inertial frames.
- Option C: An observer at rest only - While this is true, it is too restrictive since an inertial observer can also be moving at a constant velocity.
- Option D: An observer moving with uniform speed only - This is also correct, but again, it is too restrictive.
6. Conclusion:
The most accurate answer is Option B: any inertial observer, as it encompasses both observers at rest and those moving with uniform speed.
Final Answer:
The acceleration →a in Newton's second law →F=m→a is the acceleration of the mass m with respect to any inertial observer.
Newton's second law states that the force acting on an object is equal to the mass of that object multiplied by its acceleration, expressed mathematically as →F=m→a. Here, →F is the net force acting on the object, m is the mass, and →a is the acceleration.
The acceleration →a is a vector quantity, which means it has both magnitude and direction. It describes how the velocity of an object changes over time.
The question asks with respect to what the acceleration →a is defined. In classical mechanics, acceleration is typically measured with respect to an inertial frame of reference.
An inertial frame of reference is one in which an object either remains at rest or moves at a constant velocity unless acted upon by a net external force. This means that the laws of physics, including Newton's laws, hold true in such frames.
- Option A: Any observer - Incorrect, as this does not specify the type of observer.
- Option B: Any inertial observer - This is correct, as Newton's laws apply in inertial frames.
- Option C: An observer at rest only - While this is true, it is too restrictive since an inertial observer can also be moving at a constant velocity.
- Option D: An observer moving with uniform speed only - This is also correct, but again, it is too restrictive.
The most accurate answer is Option B: any inertial observer, as it encompasses both observers at rest and those moving with uniform speed.
The acceleration →a in Newton's second law →F=m→a is the acceleration of the mass m with respect to any inertial observer.
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-II
112 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - SWOE
44 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (JA)
4 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
25 Videos
Similar Questions
Knowledge Check
In the above question, the acceleration of mass m is
AFmBF−TmCF+TmDFMA balloon of mass m is rising up with an acceleration a.
AThe upthrust on the balloon is m(g+a).BThe upthrust on the balloon is ma.Cma/(2a+g) mass must be detached in order to double its acceleration.Dm/2 mass must be detached in order to double its acceleration.If the string & all the pulleys are ideal, acceleration of mass m is :-
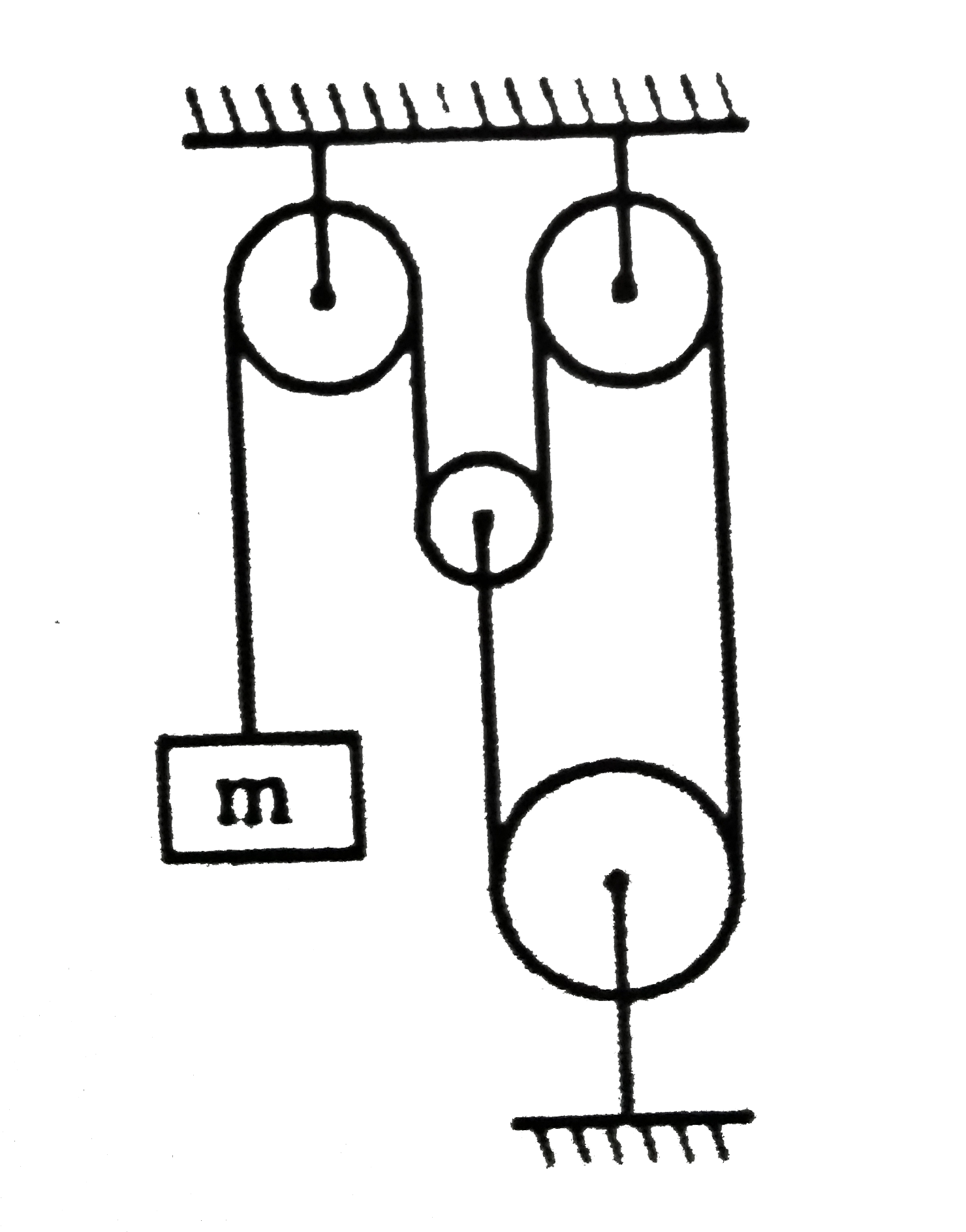 Ag2B0CgDdependent on m
Ag2B0CgDdependent on m
In the arrangement shown in the fig. the block of mass m lies on the wedge. Wedge is moving with acceleration a = 5 m/sec Find the wedge acceleration of the block with respect to wedge.
View SolutionIn the arrangement shown in the fig. the block of mass m lies on the wedge. Wedge is moving with acceleration a = 5 m/sec . Find the wedge acceleration of the block with respect to wedge.
View SolutionTwo point masses, each of mass 'm' are connected to the end of spring. If force F is applied on one of the mass, then find the acceleration of this mass if the acceleration of other mass is a ?
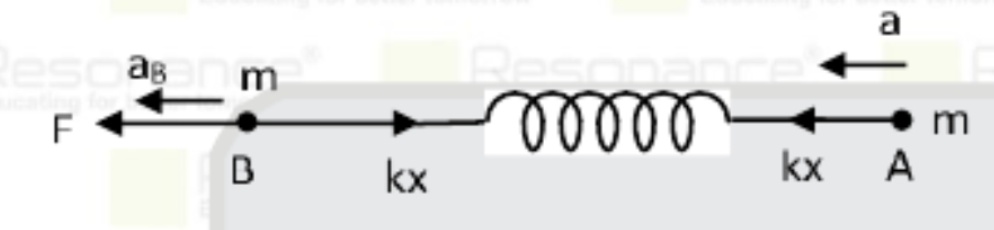
View SolutionWhen a stone of mass m is falling on the Earth of mass M, find the acceleration of Earth if any?
View SolutionA body A mass m1 exerts a force on another body B of mass m2 . If the acceleration of B be a2 , then the acceleration (in magnitude) of A is
View Solution