A meter washer has a hole of diameter $d_{1}$ and external diameter $d_{2}$ , where $d_{2} = 3 d_{1}$ . On heating , $d_{2}$ increases by $0.3 %$ . Then $d_{1}$ will :-

A

decreases by $0.1 %$

B

decrease by $0.3 %$

C

increases by $0.1 %$

D

increase by $0.3 %$ .

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:D

To solve the problem step by step, we need to analyze the relationship between the diameters of the washer and how they change with temperature.
Step 1: Understand the relationship between diameters
Given that the external diameter $d_{2}$ is three times the internal diameter $d_{1}$ :
$d_{2} = 3 d_{1}$
Step 2: Determine the change in external diameter
The problem states that the external diameter $d_{2}$ increases by $0.3 %$ . We can express this change mathematically:
$Δ d_{2} = \frac{0.3}{100} \times d_{2}$
Step 3: Calculate the new external diameter
The new external diameter $d_{2}^{'}$ after the increase can be calculated as:
$d_{2}^{'} = d_{2} + Δ d_{2} = d_{2} + \frac{0.3}{100} \times d_{2} = d_{2} (1 + \frac{0.3}{100}) = d_{2} \times 1.003$
Step 4: Relate the change in internal diameter to the external diameter
Since the washer expands uniformly, the change in the internal diameter $d_{1}$ will be proportional to the change in the external diameter. The fractional change in both diameters can be related as:
$\frac{Δ d_{1}}{d_{1}} = \frac{Δ d_{2}}{d_{2}}$
Step 5: Substitute the known values
From Step 2, we know that:
$Δ d_{2} = \frac{0.3}{100} \times d_{2}$
Thus, substituting this into the equation gives:
$\frac{Δ d_{1}}{d_{1}} = \frac{\frac{0.3}{100} \times d_{2}}{d_{2}}$
This simplifies to:
$\frac{Δ d_{1}}{d_{1}} = \frac{0.3}{100}$
Step 6: Calculate the change in internal diameter
Now we can express the change in internal diameter $Δ d_{1}$ :
$Δ d_{1} = \frac{0.3}{100} \times d_{1}$
Step 7: Determine the new internal diameter
The new internal diameter $d_{1}^{'}$ after the increase can be calculated as:
$d_{1}^{'} = d_{1} + Δ d_{1} = d_{1} + \frac{0.3}{100} \times d_{1} = d_{1} (1 + \frac{0.3}{100}) = d_{1} \times 1.003$
Conclusion
Thus, the internal diameter $d_{1}$ will also increase by $0.3 %$ when the external diameter $d_{2}$ increases by $0.3 %$ .

|

Updated on:7/8/2024

Class 12 PHYSICS RAY OPTICS AND OPTICAL INSTRUMENTS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Two holes of unequal diameters $d_{1}$ and $d_{2} (d_{1} > d_{2})$ are cut in metal sheet is heated

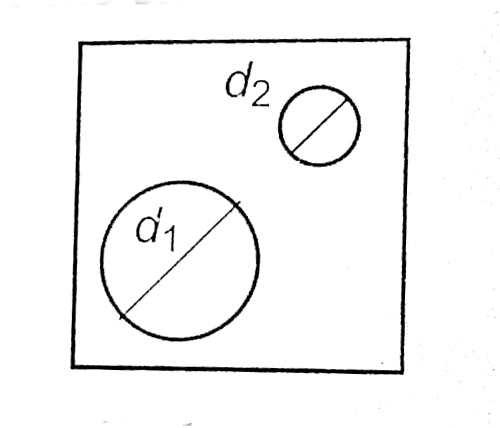Aboth $d_{1}$ and $d_{2}$ will decreases
Bboth $d_{1}$ and $d_{2}$ will increases
C $d_{1}$ will increase, $d_{2}$ will decreases
D $d_{1}$ will increase, $d_{2}$ will increases
Question 2 - Select One
The metal sheet shown in figure with two holes cut of unequal diameters $d_{1} and d_{2} (d_{1} > d_{2})$ . If the sheet is heated

Aboth $d_{1} and d_{2}$ will decrease
Bboth $d_{1} and d_{2}$ will increase
C $d_{1}$ will increase, $d_{2}$ will decrease
D $d_{1}$ will decrease $d_{2}$ will increase
Question 3 - Select One
The term 0.3d, where d being inner diameter of tube is called as
ALaplace's correction
BRayleigh's correction
CRadiation correction
DEpoch

ALLEN-GEOMETRICAL OPTICS-EXERCISE -01

Home
Ask Doubt
Profile