Two plane mirrors are placed parallel to each other at a distance L apart . A point object O is placed between them, at a distance L/3 from one mirror . Both mirrors form multiple images. The distance between any 'two images cannot be
3L/2
2L/2
2L
None of these
The correct Answer is:A
To solve the problem of determining the distance between any two images formed by two parallel mirrors, we can follow these steps:
1. Understanding the Setup:
- We have two plane mirrors placed parallel to each other at a distance L.
- A point object O is placed between the mirrors at a distance L3 from one mirror (let's call it Mirror 1).
2. Calculating Distances:
- The distance from the object O to Mirror 1 is L3.
- The distance from O to Mirror 2 is L−L3=2L3.
3. Finding the First Set of Images:
- The first image I1 formed by Mirror 1 will be at a distance L3 behind Mirror 1. Therefore, the position of I1 is:
I1=−L3
- The first image I2 formed by Mirror 2 will be at a distance 2L3 behind Mirror 2. Therefore, the position of I2 is:
I2=L+2L3=5L3
4. Finding Subsequent Images:
- The image I1 acts as an object for Mirror 2, and it will form another image I′2 at a distance L+L3=4L3 from Mirror 2.
- The image I2 acts as an object for Mirror 1, forming another image I′1 at a distance L+2L3=5L3 from Mirror 1.
5. Generalizing the Image Positions:
- The positions of the images can be generalized. The n-th image from Mirror 1 can be represented as:
In=(−1)n+1(nL+L3)
- The n-th image from Mirror 2 can be represented as:
In=(−1)n(nL+2L3)
6. Calculating Distances Between Images:
- The distance between two consecutive images can be calculated. For example, the distance between I1 and I2 is:
d=|I2−I1|=∣∣∣5L3−(−L3)∣∣∣=6L3=2L
- Similarly, the distance between any two images can be generalized as d=L or d=2L.
7. Conclusion:
- The distance between any two images cannot be 3L2, as it does not fit into the pattern of distances derived from the image positions.
Final Answer:
The distance between any two images cannot be 3L2.
- We have two plane mirrors placed parallel to each other at a distance L.
- A point object O is placed between the mirrors at a distance L3 from one mirror (let's call it Mirror 1).
- The distance from the object O to Mirror 1 is L3.
- The distance from O to Mirror 2 is L−L3=2L3.
- The first image I1 formed by Mirror 1 will be at a distance L3 behind Mirror 1. Therefore, the position of I1 is:
I1=−L3
- The first image I2 formed by Mirror 2 will be at a distance 2L3 behind Mirror 2. Therefore, the position of I2 is:
I2=L+2L3=5L3
- The image I1 acts as an object for Mirror 2, and it will form another image I′2 at a distance L+L3=4L3 from Mirror 2.
- The image I2 acts as an object for Mirror 1, forming another image I′1 at a distance L+2L3=5L3 from Mirror 1.
- The positions of the images can be generalized. The n-th image from Mirror 1 can be represented as:
In=(−1)n+1(nL+L3)
- The n-th image from Mirror 2 can be represented as:
In=(−1)n(nL+2L3)
- The distance between two consecutive images can be calculated. For example, the distance between I1 and I2 is:
d=|I2−I1|=∣∣∣5L3−(−L3)∣∣∣=6L3=2L
- Similarly, the distance between any two images can be generalized as d=L or d=2L.
- The distance between any two images cannot be 3L2, as it does not fit into the pattern of distances derived from the image positions.
The distance between any two images cannot be 3L2.
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE-312videos
GEOMETRICAL OPTICS
- BOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE-04[A]23videos
GEOMETRICAL OPTICS
- BOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE- 0145videos
GEOMETRICAL OPTICS
- BOOK - ALLENCHAPTER - CURRENT ELECTRICITYEXERCISE - All Questions427videos
CURRENT ELECTRICITY
- BOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - EXERCISE 49videos
GRAVITATION
Similar Questions
Two plane mirrors are placed parallel to each other. The distance between the mirrors is 10cm. An object is placed between the mirrors at a distance of 4cm from one of them, say M1. What is the distance between the first time formed at M1 and the second image formed at M2 ?
View SolutionTwo plane mirrors M1 and M2 are placed parallel to each other 20 cm apart. A luminous point object 'O' is placed between them at 5 cm from M1 as shown in figure :
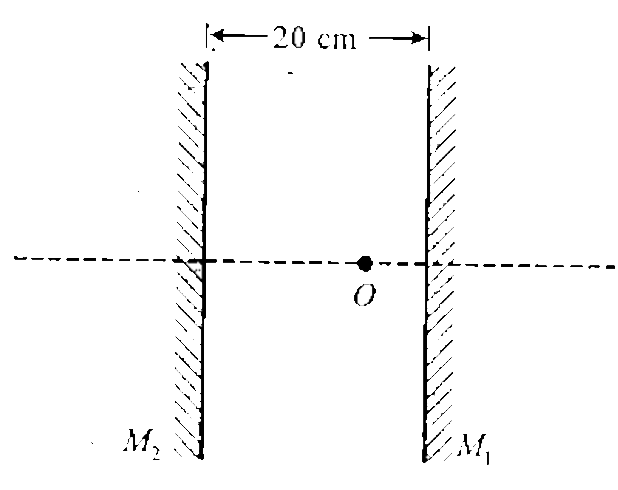 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Two plane mirrors are placed parallel to each other at a distance L apart.A point object O placed between them, at a distance L3 from one mirror.Both mirrors form multiple images.The distance between any two images cannot be
A(a)3L/2B(b)2L/2C(c)2LD(d)None of these - Question 2 - Select One
Two plane mirrors are placed parallel to each other at a distance L apart. A point object O placed between them, at a distance L/3 from the mirror. Both mirrors form multiple image. The distance between any two images cannot be
A(a)3L/2B(b)2L/2C(c)2LD(d)None of these - Question 3 - Select One
Two plane mirrors are placed parallel to each other at a distance L apart . A point object O is placed between them, at a distance L/3 from one mirror . Both mirrors form multiple images. The distance between any 'two images cannot be
A3L2B2L3C2LDNone of these
Two plane mirrors X and Y are placed parallel to each other and are separated by a distance of 20 cm. An object is placed between the two mirrors at a distance 5 cm from the mirror X. Find the distance of the first three image formed in the mirror X.
View SolutionWhen the two plane mirror are parallel to each other, then the number of images of an object placed between them is
View SolutionTwo plane mirrors A and B are placed at a distance 12 cm apart facing towards each other . An object is placed in between them at a distance 8 cm from the mirror A . What will be the distance between them at a distance 8 cm from the mirror A . What will be the distance between the first two images formed in the mirror B
View SolutionTwo plane mirrors parallel to each other and an object O placed between them. Then the distance of the first three images from the mirror M2 will be (in cm) –
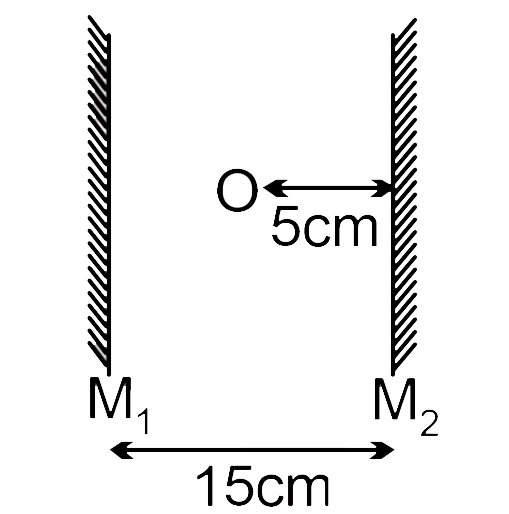 View Solution
View SolutionTwo plane mirrors are kept parallel at 20 cm from each other. A point object O is placed exactly in between them. Calculate distance between second images formed by two mirros.
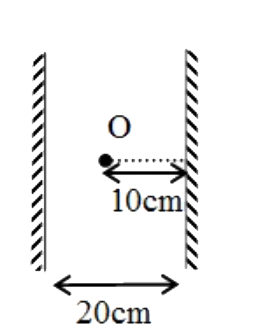 View Solution
View Solution

