Calculate the period of small oscillations of a floting box as shown in figure, which was pushed down inverical direction. The mass of box is m, area of its base is A and the density of liquid is ρ. The resistance of the liquid is assumed to be negligible.
_S01_033_Q01.png)
Initially when box is floating in liquid, if its h depth is sumerged in liquid then buoyancy force on it is
FB= weight of liquid displaced =Ahρg
_S01_033_S01.png)
As the box is in equilibrium, we have Ahρg=mg
Now if box is further pushed down by a distance x, net restorinn gorce ono it in upward (toward mean position) direction is
FB=−[A(h+x)ρg−mg]=−Axρg [ as mg=Ahρg]
It a is the accleration of box in upward direction we have a=−(Aρgm)x
Equation shows that the box executes SHM with angular frequency ω given as ω=√Aρgm
Thus time peiod of its equilibrium can be given as T=2πω=2π√mAρg
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-01117videos
SIMPLE HARMONIC MOTION
- BOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-0219videos
SIMPLE HARMONIC MOTION
- BOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)25videos
RACE
- BOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS4videos
TEST PAPER
Similar Questions
Calculate th eperiod of small oscillations of a hydrometer which was slightly pushed down in the vertical direction. The mass of the hydrometer is m=50g, the radius of its tube is r=3.2mm, the density of the liquuid is ρ=1.00g/cm3 . The rsistance of the liquid is assumed to be negligible.
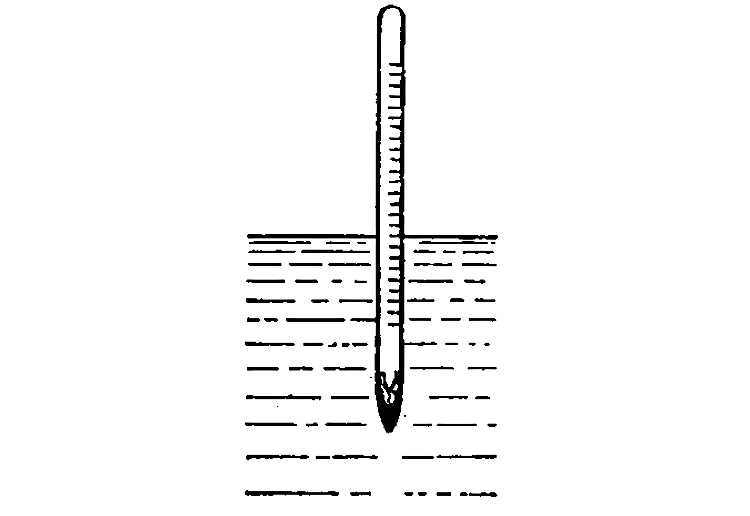View SolutionDetermine the period of small oscillations of a mathematical pendulum, that is a ball suspended by a thread l=20cm in length, if it is located in a liquuid whose density is η=3.0 times less than that of the ball. The resistance of the liquid is to be neglected.
View Solution
Knowledge Check
- Question 1 - Select One
The period of the free oscillations of the system shown here if mass m1 is pulled down a little and force constant of the spring is k and masses of the fixed pulleys are negligible, is
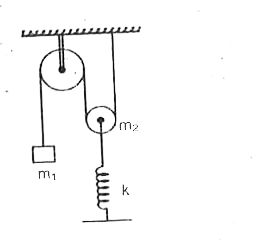AT=2π√m1+m2kBT=2π√m1+4m2kCT=2π√4m1+m2kDT=2π√3m1+m2k - Question 2 - Select One
A rectangular container moves with an acceleration a along the positive direction as shown in figure. The pressure at the A in excess of the atmospheric pressure p0 is (take ρ as the density of liquid)
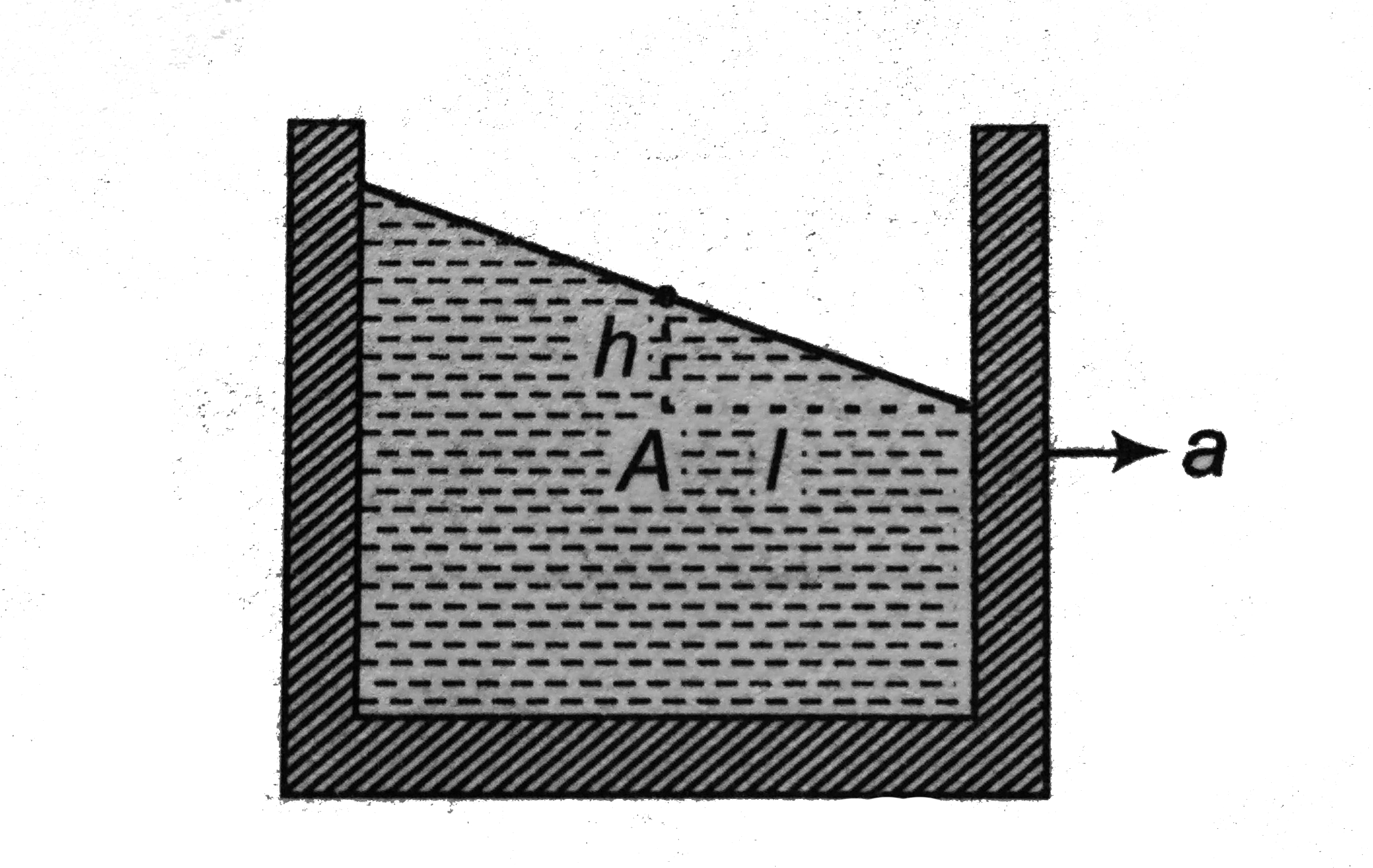AρghBρalCρ(gh+al)DBoth (a) and (b) - Question 3 - Select One
A uniform cube of mass M is floating on the surface of a liquid with three fourth of its volume immersed in the liquid (density=ρ). The length of the side of the cube is equal to
A(4M/3ρ)2/3B(M/3ρ)2/3C(M/4ρ)2/3DNone of these
Find the period of small vertical oscillations of a body with mass m in the system illustrated in figure. The stiffness values of the springs are x1 and x2, their masses are negligible.
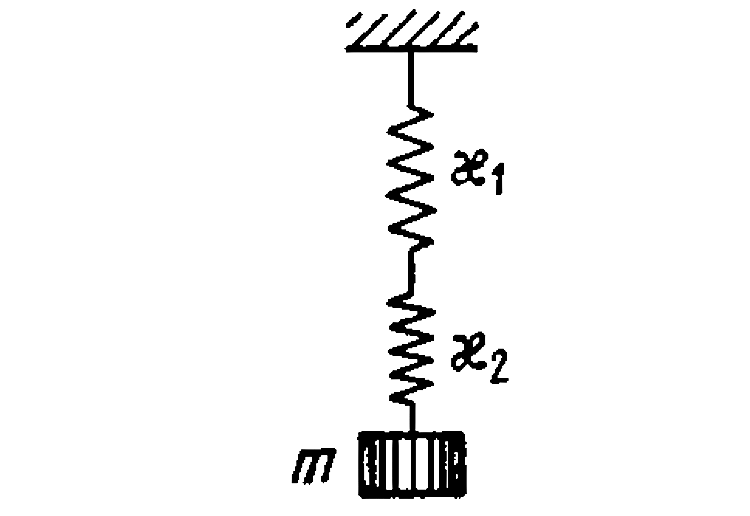View SolutionDetermine the period of small longitudinal oscillations of a body with mass m in the system shown in figure. The stiffness values of the springs are x1 and x2. The friction and the masses of the springs are negligible.
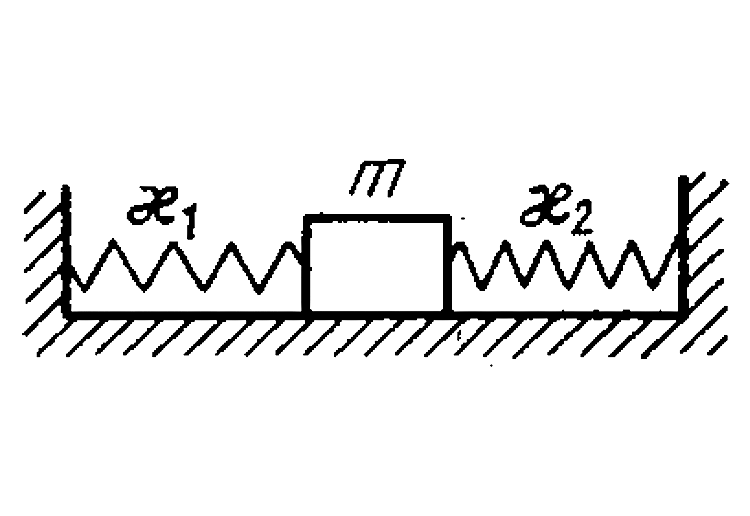View Solution_S01_075_Q01.png)
A cylinderical container of length L is full to the brim with a liquid which has mass density ρ. It is placed on a weight scale, the scale reading is w. A light wall ball which would float on the liquid if allowed to do so, of volume V and mass m is pushed gently down and held beneath the surface of the liquid with a rigid rod of negligible volume as shown on the left
Q. What is the mass M of liquid which overflowed while the ball was being pushed into the liquid?
(a). ρV
(b). m
(c). m−ρV
(d). none of theseView Solution_S01_076_Q01.png)
A cylinderical container of length L is full to the brim with a liquid which has mass density ρ. It is placed on a weight scale, the scale reading is w. A light wall ball which would float on the liquid if allowed to do so, of volume V and mass m is pushed gently down and held beneath the surface of the liquid with a rigid rod of negligible volume as shown on the left
Q. What is the reading of the scale when the ball is fully immersed
(a). w−ρVg
(b). w
(c). w+mg−ρVg
(d). none of theseView SolutionA triangular lamina of area A and height h is immersed in a liquid of density ρ in a vertical plane with its base on the surface of the liquid. The thrust on the lamina is.
View Solution