Calculate the enthalpy change for the given reaction from data provided (kJ/mole)
$\begin{matrix} H A (g) & + & B (g) & - - \to & A H B (s) (weak acid) & (weak acid) & (Salt) \end{matrix}$
$Δ H_{neutralization} {H A (a q) [at infinite dilution] / B (a q) [at infinite dilution]} = - 40 kJ/mole$
$\begin{matrix} Δ H_{solution} [H A (g)] & = - 10 kJ/mole & {at infinite dilution} Δ H_{solution} [B (g)] & = - 5 kJ/mole & {at infinite dilution} Δ H_{solution} [H A B (s)] & = + 8 kJ/mole & {at infinite dilution} \end{matrix}$

A

$- 36$

B

$- 63$

C

$- 45$

D

$- 37$

Text Solution

Verified by Experts

The correct Answer is:B

$\begin{matrix} H A (g) & + & B (g) & Δ H - ---- \to & A H B (s) + n H_{2} O ↓ Δ H_{1} & + n H_{2} O ↓ Δ H_{2} & + n H_{2} O ↓ Δ H_{4} H A (a q) & + & B (a q) & Δ H_{3} - ---- \to & A^{-} (a q) + B^{+} (a q) \end{matrix}$
$Δ H = Δ H_{1} + Δ H_{2} + Δ H_{3} - Δ H_{4}$
$= - 10 - 5 - 8 - 40 = - 63 kJ/mole$

Updated on:21/07/2023

|

Class 12 CHEMISTRY THERMOCHEMISTRY

Topper's Solved these Questions

Similar Questions

Knowledge Check

Calculate the ethanly change for the given reaction from data provided (KJ/mole)
$\begin{matrix} H A (g) & + & B (g) & - - \to & A H B (s) (weak acid) & (Weak base) & (Salt) \end{matrix}$
$Δ H_{neutralization} {H A (a q) [at infinte dilution] / B (a q)$
$[at infinite dilution] = - 40 k J / mole$
$Δ H_{solution} [H A (a q)] = - 10 k J / mole {at infinite dilution}$
$Δ H_{soltuion} [B (g) = - 5 K J / mole {at infinite dilution}$
$Δ H_{solution} [H A B (s)] = + 8 K J / mole$
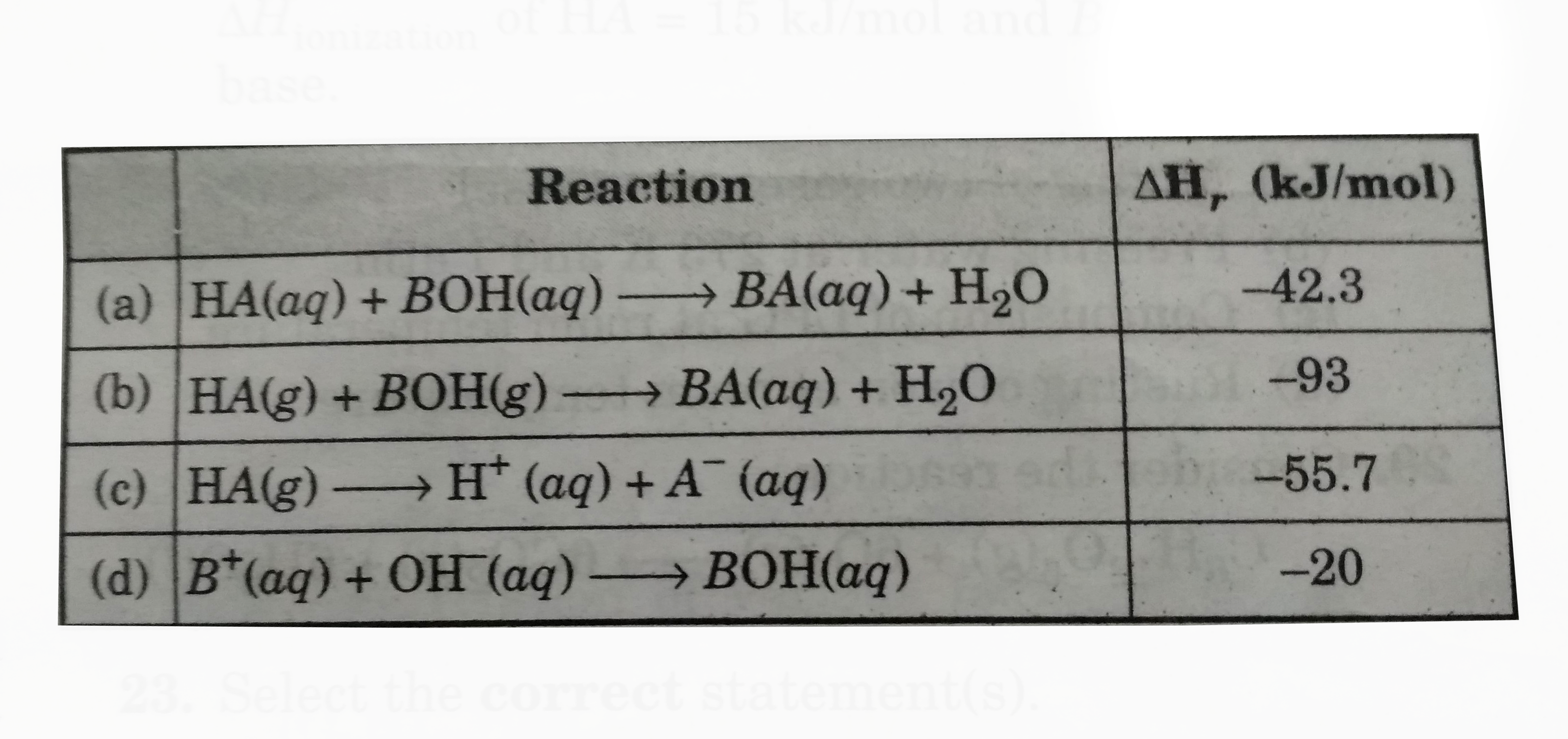
${at infinite dilution}$
A $- 36$
B $- 63$
C $- 45$
D $- 37$
From the following data, mark the option (s) where $Δ H$ is correctly written for the given reaction. Given:
$H^{+} (a q) + O H^{-} (a q) \to H_{2} O (l) Δ H = - 57.3 k J$
$Δ H_{solution}$ of $H A (g) = - 70.7$ kJ/mol
$Δ_{solution}$ of $B O H (g) = 20$ kJ/mol
$Δ H_{ionization}$ of $H A = 15$ kJ/mol & $B O H$ is a strong base
A $\begin{matrix} Reaction & Δ H_{R} (kJ/mole) H A (a q) + B O H (a q) \to B A (a q) + H_{2} O & - 42.3 \end{matrix}$
B $\begin{matrix} Reaction & Δ H_{R} (kJ/mole) H A (g) + B O H (g) \to B A (a q) + H_{2} O & - 93 \end{matrix}$
C $\begin{matrix} Reaction & Δ H_{R} (kJ/mole) H A (g) \to H^{+} (a q) + A^{-} (a q) & - 55.7 \end{matrix}$
D $\begin{matrix} Reaction & Δ H_{R} (kJ/mole) B^{+} (a q) + O H^{-} (a q) \to B O H (a q) & - 20 \end{matrix}$
Calculate bond energy of $X - X$ bond from the following data.

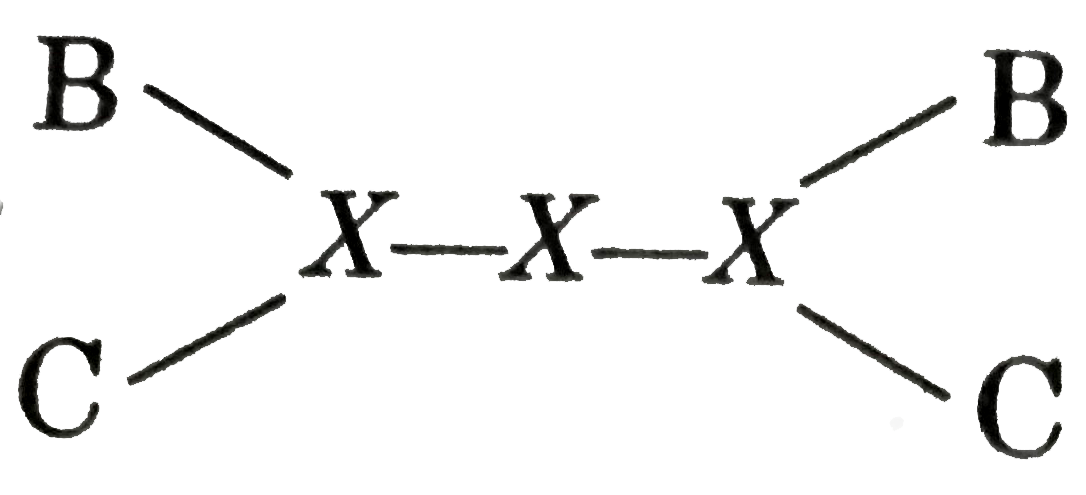
$Δ H_{f} of X (g) = + 300 k J / mole$

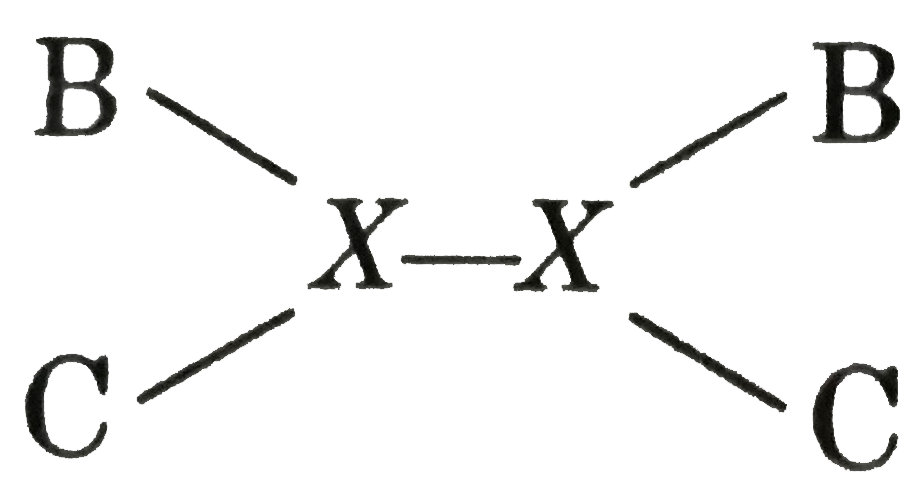A400 kJ
B200 kJ
C100 kJ
D150 kJ

BANSAL-THERMOCHEMISTRY-SOLVED EXAMPLE

Home
Ask Doubt
Profile