One mole monoatomic ideal gas was taken through process ABCD as show in . Calculate
(i) WAB,WBC,WCD,WDA
(ii) qAB,qBC,qCD,qDA
(iii) ΔHAB,ΔHBC,ΔHCD,ΔHDA
[Use:ln(3/2)=0.40:In(4/3)=0.29]
![One mole monoatomic ideal gas was taken through process ABCD as show in . Calculate (i) W(AB), W(BC), W(CD),W(DA) (ii) q(AB), q(BC), q(CD),q(DA) (iii) DeltaH(AB), DeltaH(BC), DeltaH(CD),DeltaH(DA) [Use : ln (3//2) = 0.40 : In (4//3) = 0. 29]](https://d10lpgp6xz60nq.cloudfront.net/physics_images/BSL_CHM_THRM_S01_033_Q01.png)
Text Solution
(i) WAB : Along 'AB' pressure is variable
from graph : V−10T−300=15−10600−300⇒60V−600=T−300⇒60V−300=T
sub value of T in ideal gas equation
PV=R(60V−300) ⇒ P=60R−300RV
WAB=−15∫10PdV=−15∫10[60R−300RV]dV=−60R(V2−V1)+300Rln(V2V1)
=−300R+300R(0.4) (taken ln(3/2)=0.10data)
WAB=−180R=−1496.52J
WBC=−RTln(V3V2)=−RT(4/3) (taken ln (4/3)=0.29data)
=−8.314×600×0.29=−1446.63Joule
WCD=0
WDA=−nRTln(V1V2)=−1×8.314×300ln(1020)=+1728.84Joule
(ii) qAB=ΔEAB−W=32R×300+1496.52=450R+180R=630R=5237.82Joule
qBC=−WBC=+1446.63Joule
qCD=ΔU=nCVΔT=1×32R×(300−600)=−3741.3Joule
qDA=−WDA=1728.84Joule
(iii) ΔHAB=CP(T2−T1)=53R×300=750R=6235.5Joule
ΔHBC=0
ΔHCD=ΔUCD+nRΔT=nCpΔT=1×52R×(−300)=−6235.5Joule
ΔHDA=ΔUDA+nRΔT=0Joule
|
Topper's Solved these Questions
- THERMODYNAMICSBOOK - BANSALCHAPTER - THERMODYNAMICSEXERCISE - Exercise
14 Videos
- THERMODYNAMICSBOOK - BANSALCHAPTER - THERMODYNAMICSEXERCISE - Exercise 1
35 Videos
- THERMODYNAMICSBOOK - BANSALCHAPTER - THERMODYNAMICSEXERCISE - Exercise 3
43 Videos
- THERMOCHEMISTRYBOOK - BANSALCHAPTER - THERMOCHEMISTRYEXERCISE - Exercise 4
15 Videos
Similar Questions
Knowledge Check
q.w, ΔE and ΔH for the following process ABCD on a monoatomic gas are:
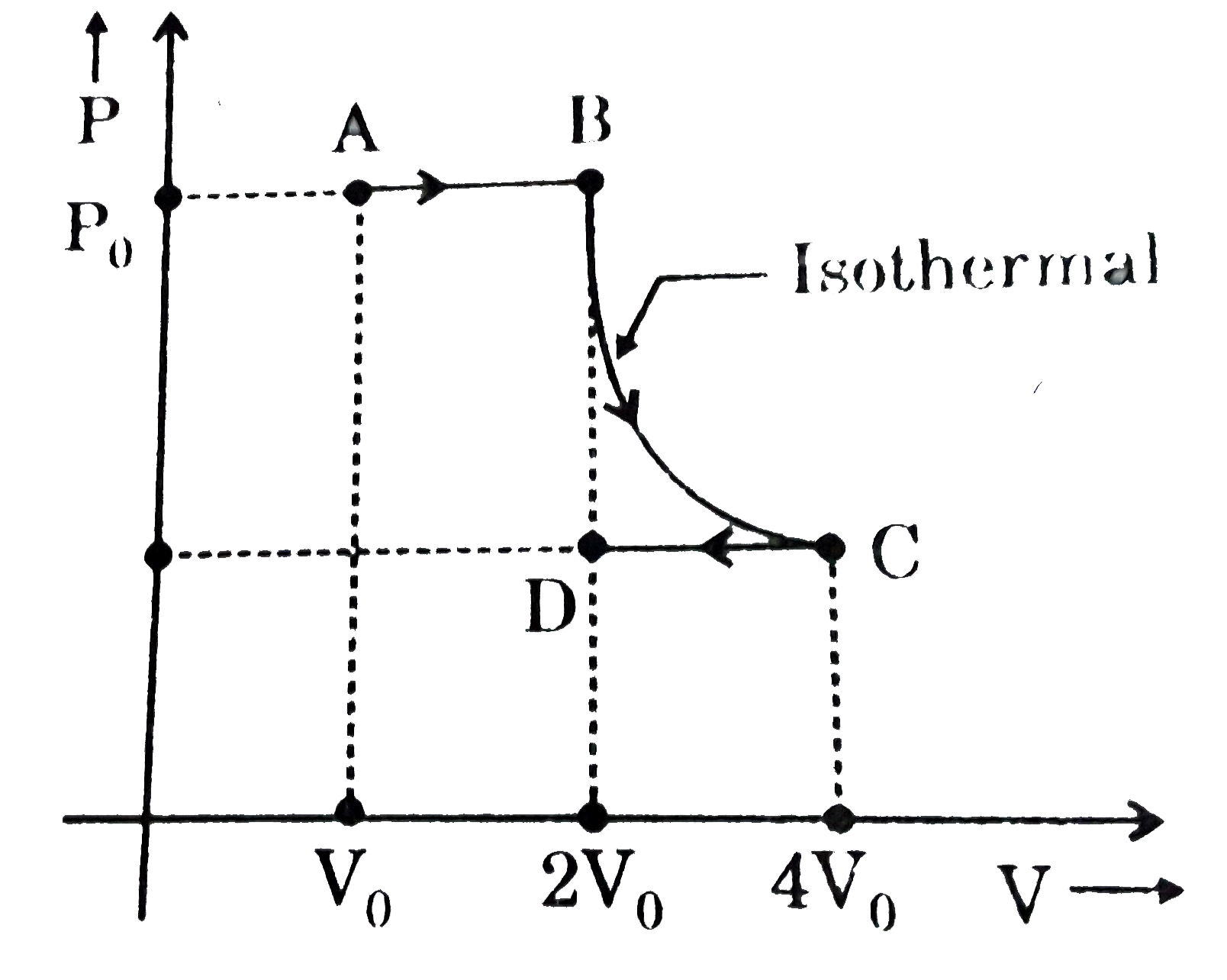 Aw=−2P0V0ln2q=2P0V0ln2ΔE=0ΔH=0Bw=−2P0V0ln2q=2P0V0lnΔE=0ΔH=2P0V0ln2Cw=−P0V0(1+ln2)q=P0V0(1+ln2)ΔE=0ΔH=0Dw=−P0V0ln2q=P0V0ln2ΔE=0ΔH=0
Aw=−2P0V0ln2q=2P0V0ln2ΔE=0ΔH=0Bw=−2P0V0ln2q=2P0V0lnΔE=0ΔH=2P0V0ln2Cw=−P0V0(1+ln2)q=P0V0(1+ln2)ΔE=0ΔH=0Dw=−P0V0ln2q=P0V0ln2ΔE=0ΔH=0A cyclic process for an ideal gas is shown in figure . Given WAB=+700J,WBC=+400J,QCA=−100J.
_E01_231_Q01.png)
Find WCAA−500JB500JC400JD−400JA cyclic process for an ideal gas is shown in figure . Given WAB=+700J,WBC=+400J,QCA=−100J.
_E01_230_Q01.png)
Find ΔUBCA−700JB−400JC−100JD400J
A cyclic process for an ideal gas is shown in figure . Given WAB=+700J,WBC=+400J,QCA=−100J.
_E01_230_Q01.png)
Find ΔUBC
View SolutionIn a quadrilateral ABCD, prove that :
(i) AB+BC+CD>DA
(ii) AB+BC+CD+DA>2AC
(iii) AB+BC+CD+DA>2BD
View SolutionABCD is quadrilateral. Is AB+BC+CD+DA<2(AC+BD)?
View SolutionIf ABCD is a square then,
2¯¯¯¯¯¯AB+4¯¯¯¯¯¯BC+5¯¯¯¯¯¯CD+7¯¯¯¯¯¯DA=
View SolutionIf ABCD is a square, then
¯¯¯¯¯¯AB+2¯¯¯¯¯¯BC+3¯¯¯¯¯¯CD+4¯¯¯¯¯¯DA =
View Solution

