L=√l(l+1)h2π
On the other hand, m determines Z-component of orbital angular momentum as Lz=m(h2π)
Hund's rule states that in degenerate orbitals electron s do not pair up unless and until each such orbital has got an electron with parallel spins. Besides orbital motion, an electron also possess spin-motion. spin may be clockwise and anti-clockwise. Both thes spin motions are called two spin states of electron characterised by spin. s=+12 and s=−12, respectively.
The orbital angular momentum of electron (l=1) makes an angle of 45∘ from Z-axis. The Lz of electron will be
2(h2π)
0(h2π)
h2π
3(h2π)
The correct Answer is:C
Step by step video, text & image solution for L = sqrt(l(l+1)) (h)/(2pi) On the other hand, m determines Z-component of orbital angular momentum as L_(z) = m ((h)/(2pi)) Hund's rule states that in degenerate orbitals electron s do not pair up unless and until each such orbital has got an electron with parallel spins. Besides orbital motion, an electron also possess spin-motion. spin may be clockwise and anti-clockwise. Both thes spin motions are called two spin states of electron characterised by spin. s = +(1)/(2) and s =- (1)/(2), respectively. The orbital angular momentum of electron (l =1) makes an angle of 45^(@) from Z-axis. The L_(z) of electron will be by Chemistry experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Complete the following reaction
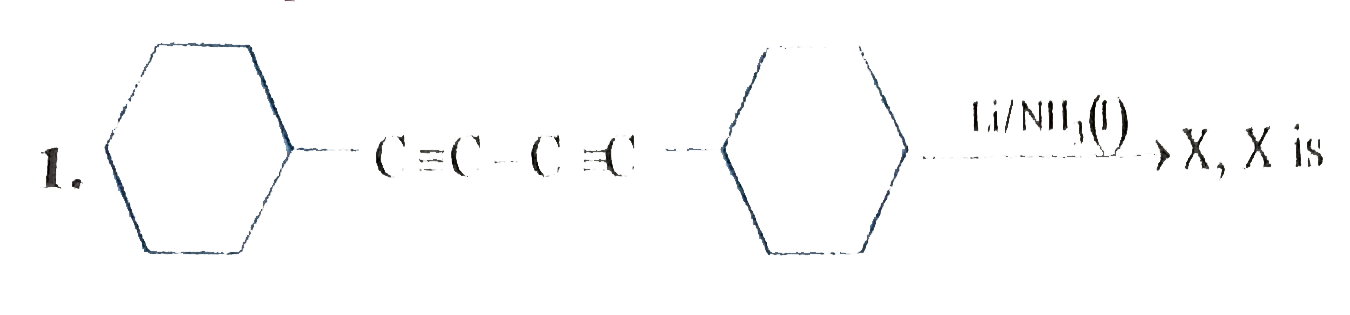
View Solution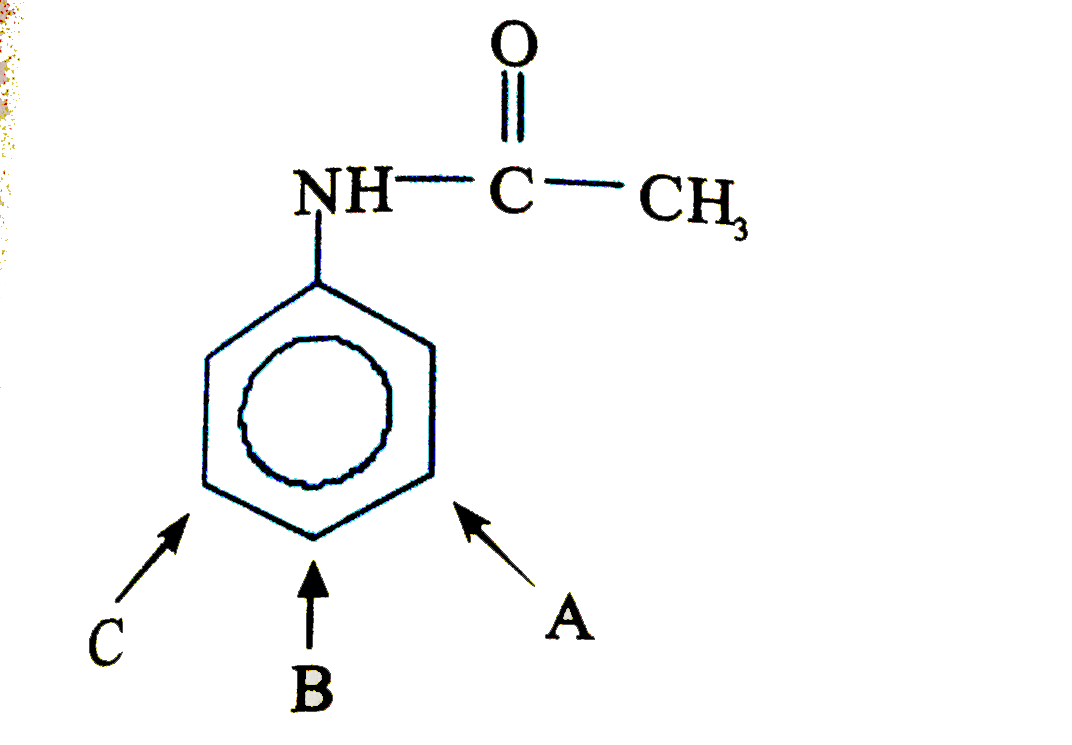
Identify the position where electrophilic aromatic substitution EAS) is most favourable
View SolutionSpin angular momentum of an electron has no analogue in classical mechanics. Howerver, it turns out that the treatment of spin angular momentum is closely analogous to the treatment of orbital angular momentum Spin angular momentum =sqrt(s(s+1))h Orbital angular momentum =sqrt(l(l+1))h Total spin of ana tom or ion is a multiple of (1)/(2) . Spin multiplicity is a factor to confirm the electronic configuration of an atom or ion. Spin multiplicity =(2sums+1) Q. Total spin of Mn^(2+)(Z=25) ion will be:
View SolutionSpin angular momentum of an electron has no analogue in classical mechanics. Howerver, it turns out that the treatment of spin angular momentum is closely analogous to the treatment of orbital angular momentum
Spin angular momentum =√s(s+1)h
Orbital angular momentum=√l(l+1)h
Total spin of ana tom or ion is a multiple of 12. Spin multiplicity is a factor to confirm the electronic configuration of an atom or ion.
Spin multiplicity =(2∑s+1)
Q. Which of the following electronic configurations have four spin multiplicity?
View SolutionSpin angular momentum of an electron has no analogue in classical mechanics. Howerver, it turns out that the treatment of spin angular momentum is closely analogous to the treatment of orbital angular momentum Spin angular momentum =sqrt(s(s+1))h Orbital angular momentum =sqrt(l(l+1))h Total spin of ana tom or ion is a multiple of (1)/(2) . Spin multiplicity is a factor to confirm the electronic configuration of an atom or ion. Spin multiplicity =(2sums+1) Q. In any subshell, the maximum number of electrons having same value of spin quantum numbers is:
View SolutionSpin angular momentum of an electron has no analogue in classical mechanics. Howerver, it turns out that the treatment of spin angular momentum is closely analogous to the treatment of orbital angular momentum Spin angular momentum =sqrt(s(s+1))h Orbital angular momentum =sqrt(l(l+1))h Total spin of ana tom or ion is a multiple of (1)/(2) . Spin multiplicity is a factor to confirm the electronic configuration of an atom or ion. Spin multiplicity =(2sums+1) Q. The orbital angular momentum of a 2p-electron is:
View SolutionThe ratio of orbital angular momentum and spin angular momentum of an electron in 'p' orbital is
View SolutionIt is tempting to think that all possible transition are permissible and that an atomic spectrum arises from the transition of an electron from any initial orbital to any other orbital .However this is not so because a photon a photon has as intrinsic spin angular momentum of √2h/2π corresponding to S=1 although it has no charge and no rest mass
On the other hand , an electron has got two type of angular momentum: orbital angular momentum
L=[√l(l+1)]h/2π,and spin angular momentum L1=√s(s+1)h/2π arising from orbital motion and spin motion of the electron during any electronic transition must compensate for the angular momentum carried away by the photon .To satisfy this condition the different between the azimuthal quantum number of the orbital within which the transition take place must differ by 1.thus, an electron in a d-orbital (l=2) cannot make a transition into as s-orbital (l=0)because the photon cannot carry away enough angular momentum
The maximum orbital angular momentum of an electron with n=5 is
View SolutionThe sum of spins of all the electron is the total spins(S) and (2S+1) is called spin multiplicity of the electronic configuration. Hund's rule defines the ground state configuration of electrons in degenerate orbitals i.e., orbitals within the same sub-shell which have the same values of n and l, states thta in degenerate orbitals pairing of electrons does not occur unless and until all such orbitals are filled singly with their parallel spin. A spinning electron behaves as though it were a tiny bar magnet with poles lying on the axis of spin. The magnetic moment of any atom, ion or molecule due to spin called spin-only magnetic moment (m_(s)) is given by the formula. mu_(s) = sqrt(n(n+2))B.M where n= number of unpaired electron(s) The spin-only magnetic moment of Cr^(3+) ?
View SolutionThe sum of spins of all the electron is the total spins(S) and (2S+1) is called spin multiplicity of the electronic configuration. Hund's rule defines the ground state configuration of electrons in degenerate orbitals i.e., orbitals within the same sub-shell which have the same values of n and l, states thta in degenerate orbitals pairing of electrons does not occur unless and until all such orbitals are filled singly with their parallel spin. A spinning electron behaves as though it were a tiny bar magnet with poles lying on the axis of spin. The magnetic moment of any atom, ion or molecule due to spin called spin-only magnetic moment (ms) is given by the formula.
μs=√n(n+2)B.M
where n= number of unpaired electron(s)
The spin-multiplicity of Fe3+[Ec=[Ar]3d5) in its ground state
View SolutionIn the degenerate orbitals, unpaired electrons have ____________ spin
View Solutionएक कक्षा में चक्कर लगा रहे इलेक्ट्रॉन का कक्षकीय कोणीय संवेग ( orbital angular momentum ) h2π√l(l+1) है । s-इलेक्ट्रॉन के लिए इसका संवेग है :
View Solutionগৌণ কোয়ান্টাম সংখ্যা l-এর জন্য কোনো অর্বিট্যালে ইলেকট্রনের কৌণিক ভরবেগ √l(l+1)h2π হলে s -অর্বিট্যালে ইলেকট্রনের কৌণিক ভরবেগের মান -
View Solutionएक कक्षक में एक घूमते हुए इलेक्ट्रॉन का कक्षक कोणीय संवेग √l(l+1).h2π है। s-इलेक्ट्रॉन के लिए संवेग का मान होगा
View SolutionSpin angular momentum of an electron has no analog in classical mechanics. However, it turns out that the treatment of spin angular momentum is closely analogous to the treatment of orbital angular momentum.
Spin angular momentum =√s(s+1)h
Orbital angular momentum =√l(l+1)h
Total spin of an atom or ion is a multiple of 12. Spin multiplicity is a factor to confirm the electronic configuration of an atom or ion.
Spin multiplicity (2∑s+1)
Total spin of Mn2+ = (Z = 25) ion will be :
View Solution
