Let us consider a binary solution of two volatile liquids 'A' and B, when taken in a closed container.Both the components would evaporate and an equilibrium would be established between vapour phase and liquid phase .Let the total pressure at this stage be ptotal and pA and pB are partial pressures of A and B. Mole fractions of these components in liquid solution are xA and xB, that of vapour phase and yA and yB respectively p∘A and p∘B are vapour pressure of pure A & pure B .
Ideal liquid solution follow Raoult's law and non ideal liquid - liquid solution does not follow Raoult's law, deviation is measured in term of activity coefficient γ
γ=prealpideal
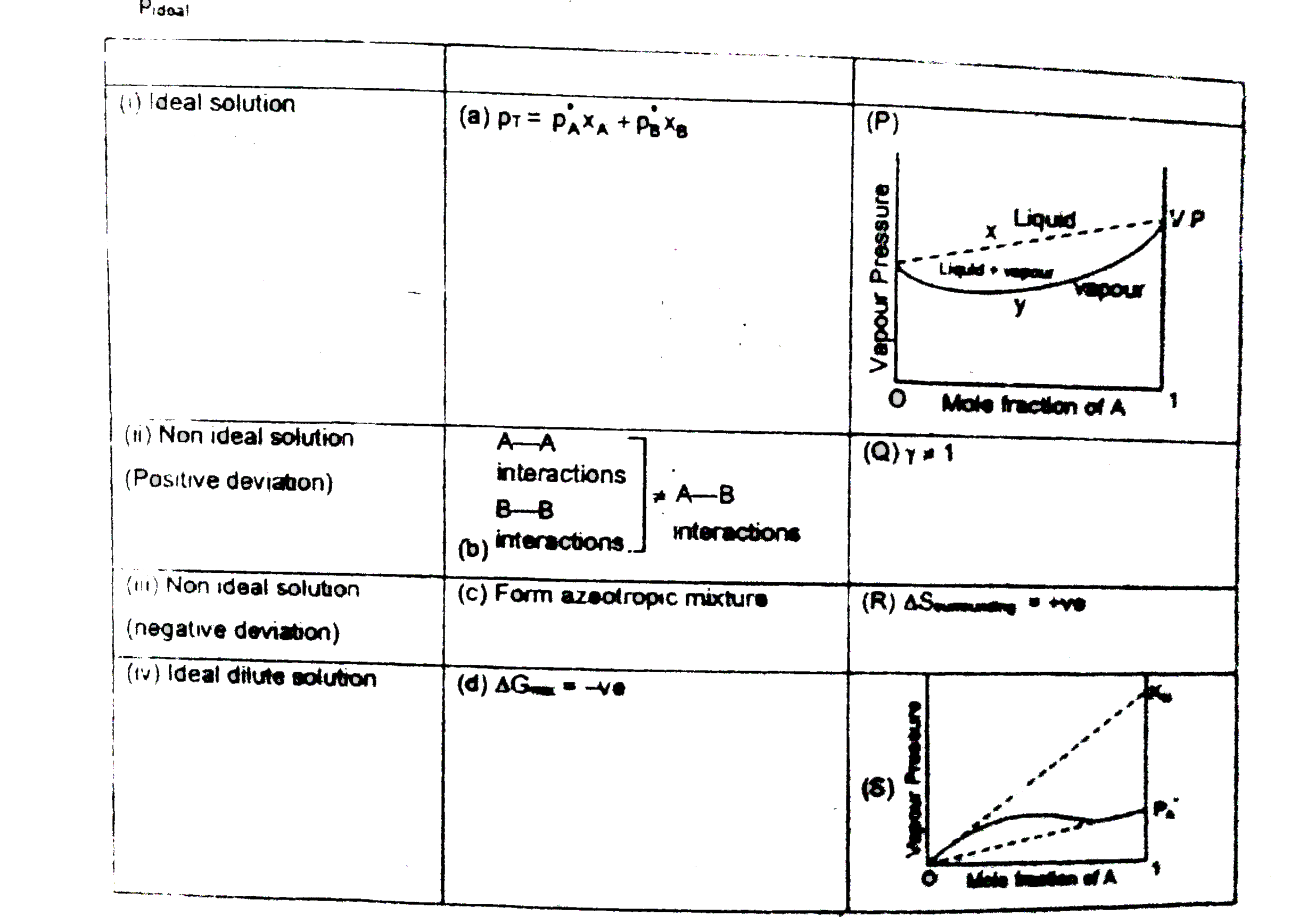
For water + alcohol solution correct set is :
(ii)(b)(Q)
(ii)(c )(R)
(iv)(d)(S)
(iv)(c )(R)
The correct Answer is:B
water+alcohol→ non ideal solution with +ve derivation correct code for this solution are (ii),(iv),b,c,d,Q,S
Topper's Solved these Questions
Similar Questions
If PA is the vapour pressure of a pure liquid A and the mole fraction of A in the mixture of two liquids A and B is x, the parial vapour pressure of A is:
View SolutionThe mole fraction of component A in vapour phase is χ1 and mole fraction of component A in liquid mixture is χ2(P∘A=vapour pressure of pure A,P∘B=vapour pressure of pure B).Then total vapour pressure of the liquid mixture is
View Solution
Knowledge Check
Let us consider a binary solution of two volatile liquids 'A' and B, when taken in a closed container.Both the components would evaporate and an equilibrium would be established between vapour phase and liquid phase .Let the total pressure at this stage be ptotal and pA and pB are partial pressures of A and B. Mole fractions of these components in liquid solution are xA and xB, that of vapour phase and yA and yB respectively p∘A and p∘B are vapour pressure of pure A & pure B .
Ideal liquid solution follow Raoult's law and non ideal liquid - liquid solution does not follow Raoult's law, deviation is measured in term of activity coefficient γ
γ=prealpideal
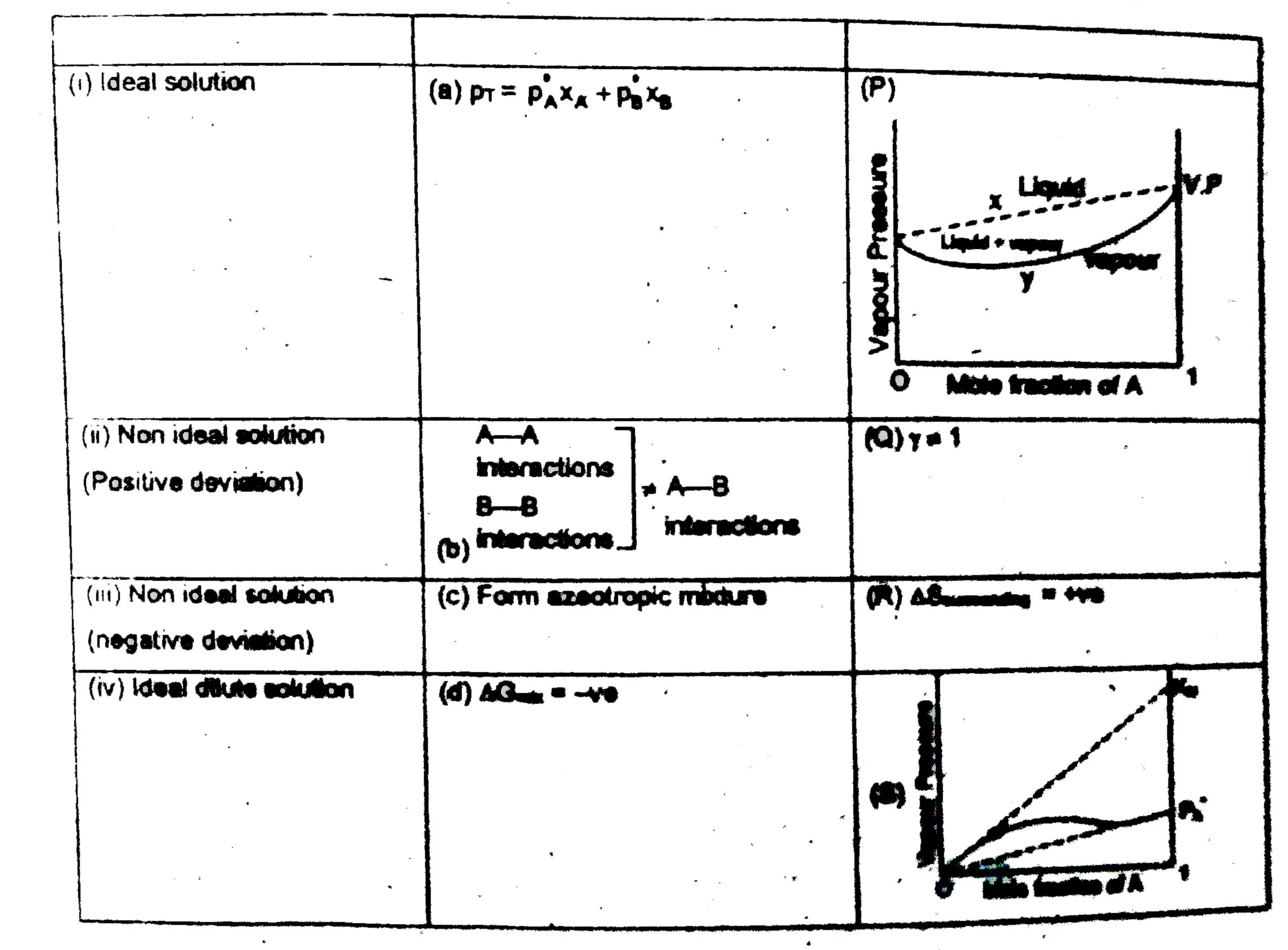
For water + H2SO4 solution correct set isA(i)(a)(P)B(ii)(b)(Q)C(iii)(d)(R)D(iv)(c )(P)Let us consider a binary solution of two volatile liquids 'A' and B, when taken in a closed container.Both the components would evaporate and an equilibrium would be established between vapour phase and liquid phase .Let the total pressure at this stage be ptotal and pA and pB are partial pressures of A and B. Mole fractions of these components in liquid solution are xA and xB, that of vapour phase and yA and yB respectively p∘A and p∘B are vapour pressure of pure A & pure B .
Ideal liquid solution follow Raoult's law and non ideal liquid - liquid solution does not follow Raoult's law, deviation is measured in term of activity coefficient γ
γ=prealpideal
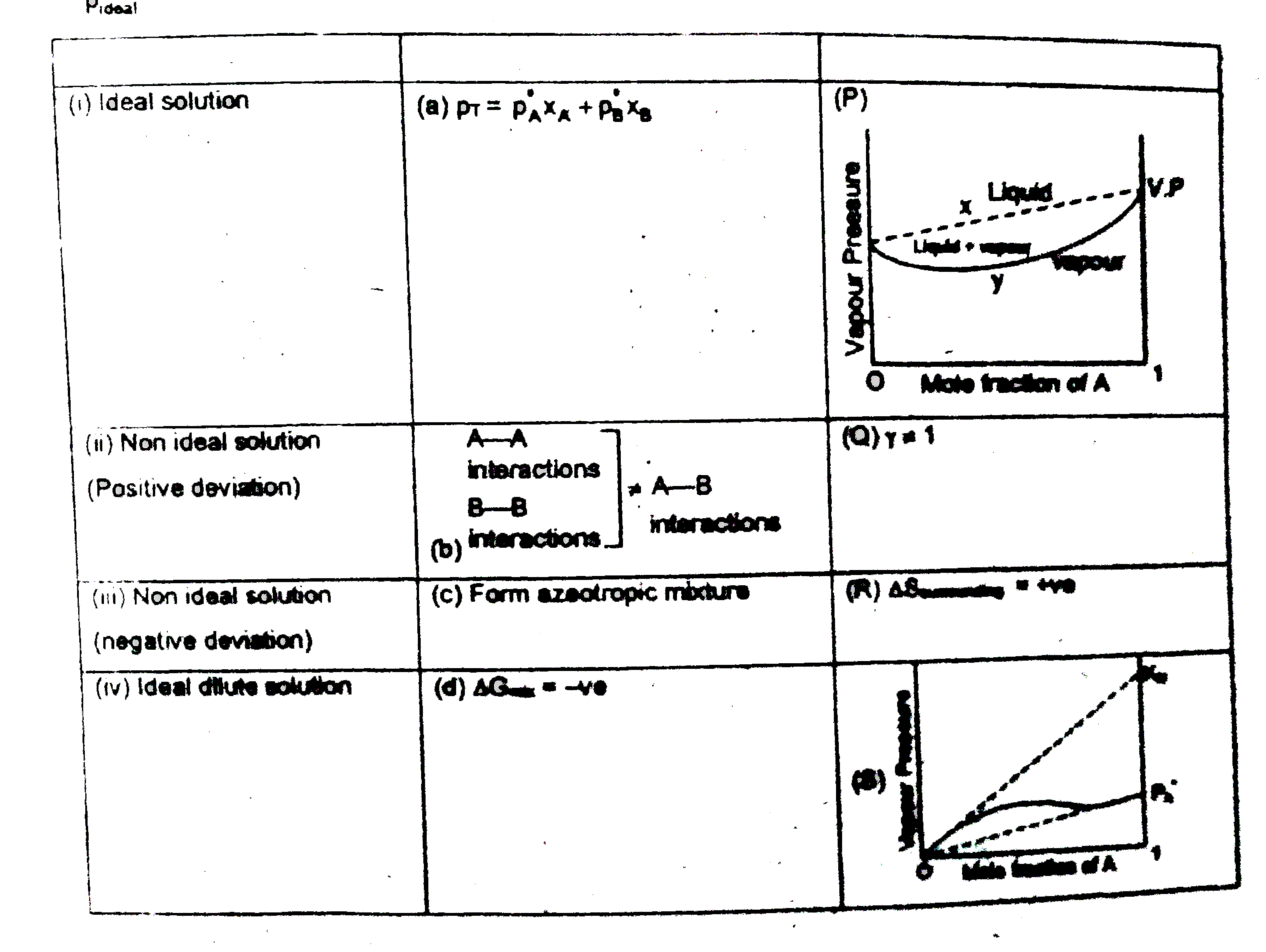
For Hexane + neptane solution correct set is :A(i)(a)(Q)B(ii)(b)(P)C(ii)(d)(R)D(i)(d)(P)PAandPB are the vapour pressure of pure liquid components ,Aand B respectively of an ideal binary solution,If xA represents the mole fraction of component A, the total pressure of the solution will be
Ap∘A−x1x2Bp∘A−x2x1Cp∘Bx1x2Dp∘Bx2x1
Mole fraction of component A in vapour phase is χ1 and that of component A in liquid mixture is χ2, then (p∘A)= vapour pressure of pure A, p∘B = vapour pressure of pure B), the total vapour pressure of liquid mixture is
View SolutionAn ideal solution has two components A is more volatile than B, i.e.P∘A>P∘B and alsoP∘A>Ptotal. If XAandYA are mole fraction of components A in liquid and vapour phases, than :
View SolutionIf PA is the vapour pressure of a pure liquid A and the mole fraction of A in the mixture of two liquids A and B is x, the parial vapour pressure of A is:
View SolutionMole fraction of component A in vapour phase is χ1 and that of component A in liquid mixture is χ2, then (p∘A)= vapour pressure of pure A, p∘B = vapour pressure of pure B), the total vapour pressure of liquid mixture is
View SolutionAn ideal solution has two components A is more volatile than B, i.e.P∘A>P∘B and alsop∘A>Ptotal. If XAandYA are mole fraction of components A in liquid and vapour phases, than :
View Solution
