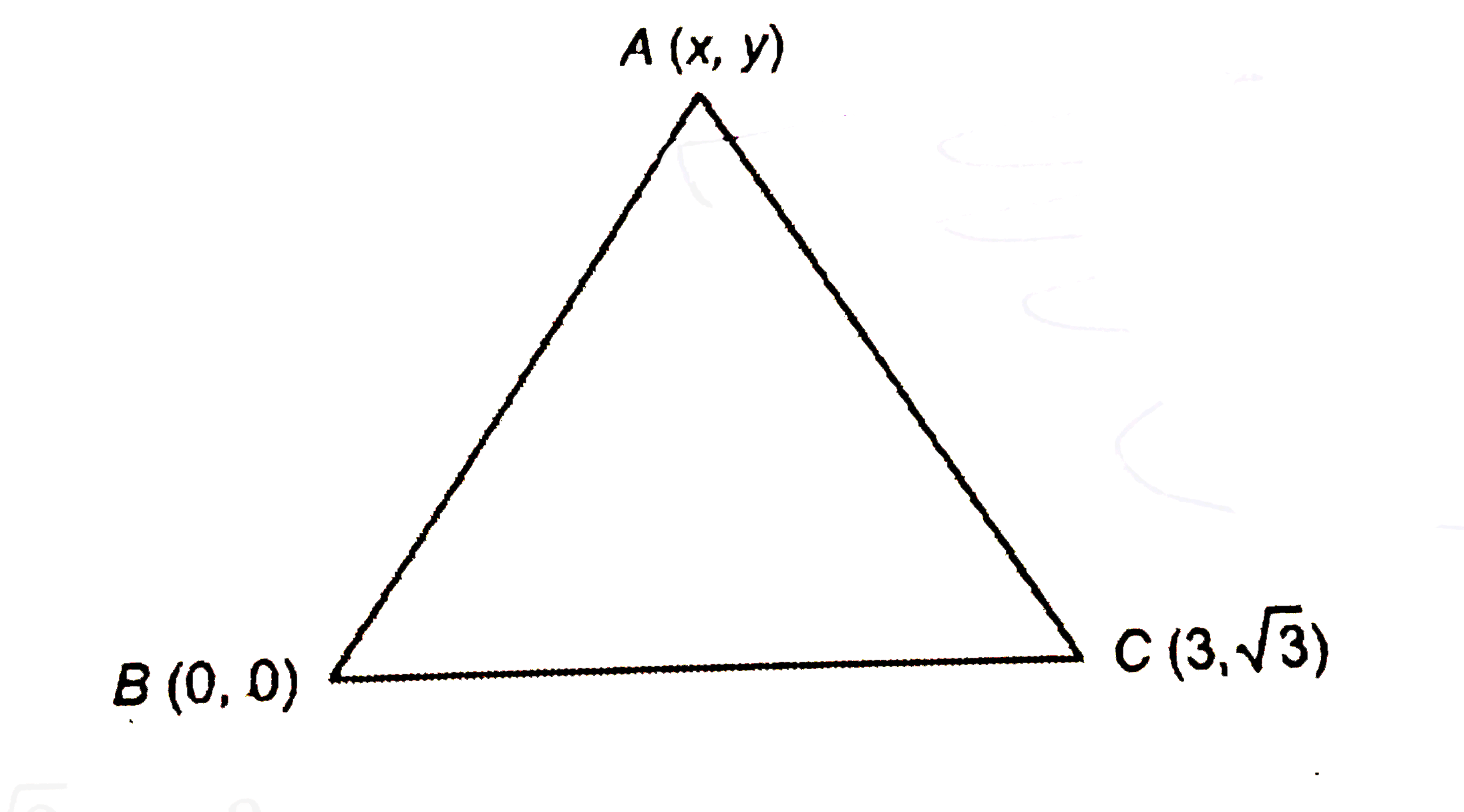
The co-ordinates of two vertices of an equilateral triangle are (0, 0) and (3, $\sqrt{3}$ ) . Find the co-ordinates of the third vertex of the triangle.

Text Solution

Verified by Experts

Let the $Δ A B C$ is an equilateral triangle in which the co-ordinates of points B and C are (0, 0) and (3, $\sqrt{3}$ ) respectively.
Let the co-ordinates of third vertex A be (x, y).
In equilateral $Δ A B C$
$A B = A C = B C$
$\Rightarrow A B^{2} = A C^{2} = B C^{2}$
$∴ A B^{2} = A C^{2}$
$\Rightarrow {(x - 0)}^{2} + {(y - 0)}^{2} = {(x - 3)}^{2} + {(y - \sqrt{3})}^{2}$
$\Rightarrow x^{2} + y^{2} = x^{2} - 6 x + 9 + y^{2} - 2 \sqrt{3} y + 3$
$\Rightarrow 6 x + 2 \sqrt{3} y = 12 \Rightarrow 3 x + \sqrt{3} y = 6$
$\Rightarrow \sqrt{3} x + y = 2 \sqrt{3} y = 2 \sqrt{3} - \sqrt{3} x ... (1)$
and $A B^{2} = B C^{2}$
$\Rightarrow {(x - 0)}^{2} + {(y - 0)}^{2} = {(3 - 0)}^{2} + {(\sqrt{3} - 0)}^{2}$
$\Rightarrow x^{2} + y^{2} = 9 + 3$
$\Rightarrow x^{2} + {(2 \sqrt{3} - \sqrt{3} x)}^{2} = 12$ [from eq. (1)]
$\Rightarrow x^{2} + 12 + 3 x^{2} - 12 x = 12$
$\Rightarrow 4 x^{2} - 12 x = 0$
$\Rightarrow 4 x (x - 3) = 0$
$\Rightarrow x = 0 or x - 3 = 0$
$\Rightarrow x = 0 or x = 3$
Put these value in eq. (1)
$x = 0,$ then $y = 2 \sqrt{3} - 0 = 2 \sqrt{3}$
$x = 3,$ then $y = 2 \sqrt{3} - 3 \sqrt{3} = - \sqrt{3}$
$∴$ Co-ordinates of third vertex = (0, $2 \sqrt{3}$ ) or (3, $- \sqrt{3}$ )

Updated on:21/07/2023

|

Class 10 MATHS COORDINATE GEOMETRY

Topper's Solved these Questions

Similar Questions

Knowledge Check

An equilateral triangle has one vertex at (0, 0) and another at $(3, \sqrt{3})$ . What are the coordinates of the third vertex ?
A $(0, 2 \sqrt{3})$ only
B $(3, \sqrt{3})$ only
C $(0, 2 \sqrt{3}) or (3, - \sqrt{3})$
DNeither $(0, 2 \sqrt{3}) n or (3, - \sqrt{3})$

NAGEEN PRAKASHAN-CO-ORDINATE GEOMETRY-Revision Exercise Short Answer Questions

Home
Ask Doubt
Profile