Solve the following system of equations by cross multiplication method
3x - 5y = 20 and 7x + 2y = 17.
Video Solution
Text Solution
The given equations can be written as
3x - 5y - 20 = 0
and 7x + 2y - 17 = 0
By cross multiplication method, we have
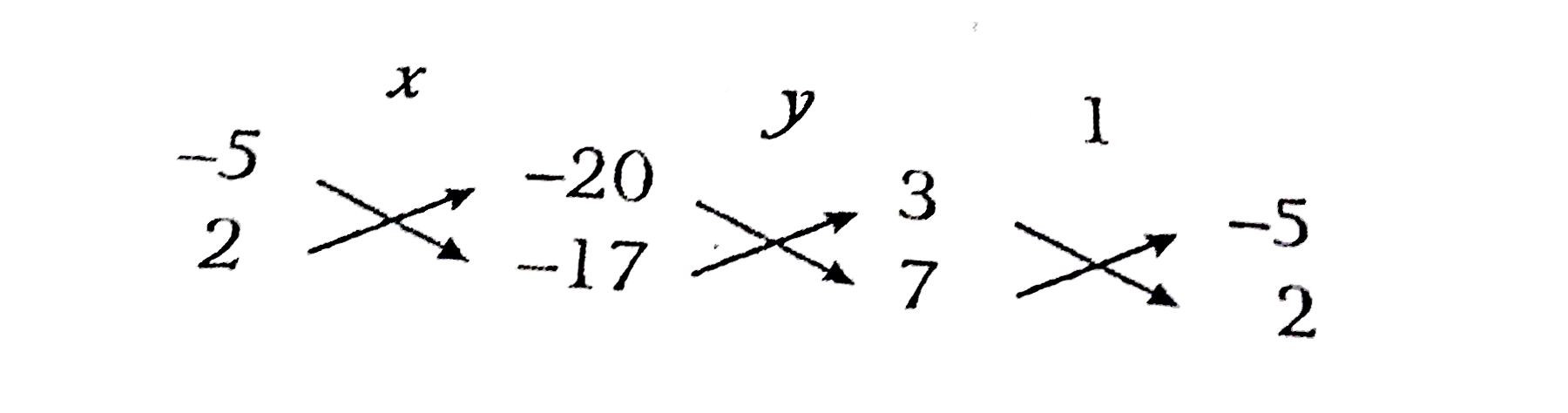
x(−5)×(−17)−2×(−20)=y(−20)×7−(−17)×3=13×2−7×(−5)
implies x85+40=y−140+51=16+35
implies x125=y−89=141
when x125=141⇒x=12541
and y−89=141⇒y=−8941
Hence, x=12541y=−8941⎫⎬⎭ is the required solution.
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHANCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - Exercise 3a18videos
LINEAR EQUATIONS IN TWO VARIABLES
- BOOK - NAGEEN PRAKASHANCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - Exercise 3b30videos
LINEAR EQUATIONS IN TWO VARIABLES
- BOOK - NAGEEN PRAKASHANCHAPTER - INTRODUCTION TO TRIGONOMETRYEXERCISE - Revision Exercise Long Answer Questions5videos
INTRODUCTION TO TRIGONOMETRY
- BOOK - NAGEEN PRAKASHANCHAPTER - POLYNOMIALSEXERCISE - Revision Exercise Long Answer Questions4videos
POLYNOMIALS
Similar Questions
Knowledge Check
- Question 1 - Select One
Solve the following system of linear equations by cross-miltiplication method.
3x+4y=7and2x+5y=6A117,47B−117,47C11,57DNone of these - Question 2 - Select One
Solve the following system of linear equations by cross-multiplication method. 3x+4y=7and2x+5y=6
A117,47B−117,47C11,57DNone of these
Solve the following system of equations by matrix method: 5x+2y=3, 3x+2y=5
View SolutionSolve the following system of equations by matrix method: 3x+7y=4, x+2y=−1
View SolutionSolve the following system of equations by matrix method :
3x−4y=5 and 4x+2y=3View SolutionSolve the following system of equations by matrix method: 3x+y=19, 3x−y=23
View SolutionSolve the following pair of linear equations by cross multiplication method. :
x+2y=2,x−3y=7View SolutionSolve the following system of equations by method of cross-multiplication: 2x−y=6, x−y=2
View Solution

