Show that the following system of equations have unique solution :
(i)7x−2y=3(ii)3x+y=17(iii)2x+5y=1722x−3y=168x+11y=375x+3y=14
and also solve the system of equations in each case.
(i) Given equations are 7x - 2y = 3 ….(1)
22x - 3y = 16 ….(2)
Here, a1=7,b1=−2 and c1=3 [from (1)]
a22=22,b2=−3 and c2=16 [from (2)]
Now, a1a2=722 and b1b2=−2−3=23
Since a1a2≠b1b2. Hence, the given system has a unique solution.
By cross multiplication method, we have
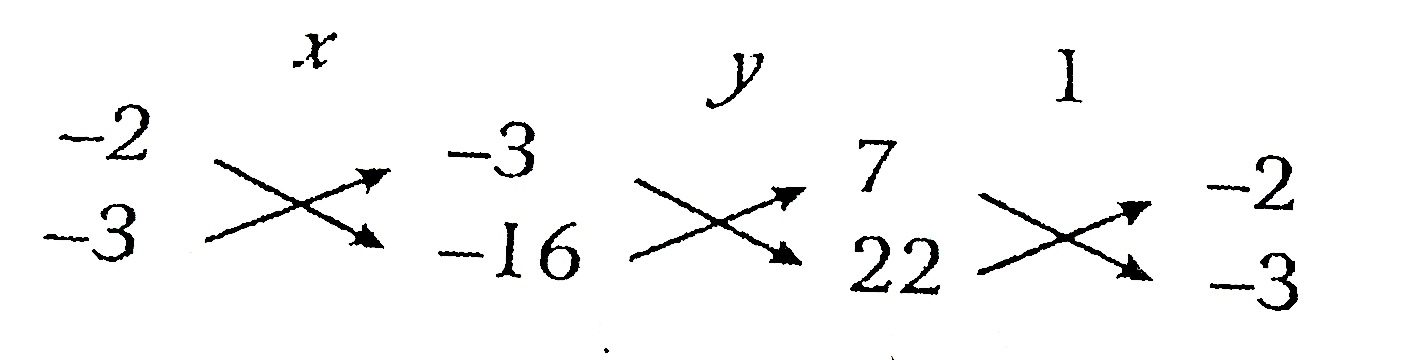
x(−2)×(−16)−(−3)×(−3)=y(−3)×22−(−16)×7=17×(−3)−22×(−2)
⇒x32−9=y−66+112=1−21+44
implies x23=y46=123
When x23=123⇒x=1
and y46=123⇒y=2
Hence, x=1y=2} is the required solution.
(ii) The given system of equations is 3x + y = 17 ....(1)
8x + 11y = 37 ....(2)
Here a1=3,b1=1 and c1=17 [from (1)]
a2=8,b2=11 and c2=37 [from (2)]
Now a1a2=38,b1b2=111
Since a1a2≠b1b2. Hence, the given system has a unique solution.
We can write the equations as
3x+y−17=0and8x+11y−37=0
By cross multiplication method, we have
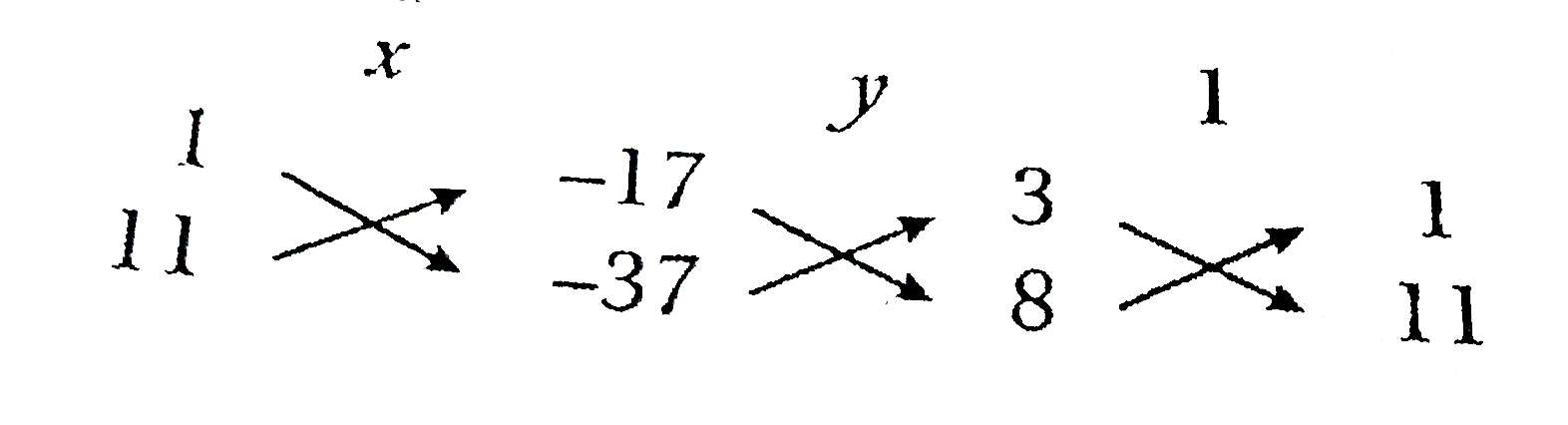
x1×(−37)−11×(−17)=y(−17)×8−(−37)×3=13×11−8×1
implies x150=y−25=125 When x150=125⇒x=6
and y−25=125⇒y=−1
Hence, x=6y=−1} is the required solution.
(iii) The given system of equations is 2x + 5y - 17 = 0 ....(1)
5x + 3y - 14 = 0 ....(2)
Here, a1=2,b1=5 and c1=−17 [from (1)]
a2=5,b2=3 and c2=−14 [from (2)]
Now, a1a2=25,b1b2=53
Since a1a2≠b1b2. Hence, the given system of equations has a unique solution.
By cross multiplication method, we have

x5×(−14)−3×(−17)=y(−17)×5−(−14)×2=12×3−5×5
implies x−70+51=y−85+28=16−25
implies x−19=y−57=1−19
implies When x−19=1−19⇒x=1
and y−57=1−19⇒y=3
Hence, x=1y=3} is the required solution.
Topper's Solved these Questions
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - NAGEEN PRAKASHANCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - Exercise 3a
18 Videos
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - NAGEEN PRAKASHANCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - Exercise 3b
30 Videos
- INTRODUCTION TO TRIGONOMETRYBOOK - NAGEEN PRAKASHANCHAPTER - INTRODUCTION TO TRIGONOMETRYEXERCISE - Revision Exercise Long Answer Questions
5 Videos
- POLYNOMIALSBOOK - NAGEEN PRAKASHANCHAPTER - POLYNOMIALSEXERCISE - Revision Exercise Long Answer Questions
4 Videos
Similar Questions
Solve the system of equations 2x+5y=1 and 3x+2y=7.
View SolutionSolve the system of equations: 2x+3y=13, 5x-y=7
View SolutionSolve the following system of equations by Cramers rule 2x−y=17 , 3x+5y=6
View SolutionSolve the following system of equations by Cramers rule 2x−y=17 , 3x+5y=6
View SolutionSolve the following system of equations by matrix method: 5x+2y=3, 3x+2y=5
View SolutionSolve the following system of equations by matrix method: 5x+2y=3, 3x+2y=5
View SolutionSolve the following system of equations by matrix method: 3x+y=7, 5x+3y=12
View SolutionSolve the following system of equations by matrix method: 3x+y=7, 5x+3y=12
View SolutionSolve the following system of linear equations by Cramers rule: x−2y=4, −3x+5y=−7
View SolutionSolve the following system of linear equations by Cramers rule: x−2y=4, −3x+5y=−7
View Solution
