The students of a class are made to stand in rows. If 3 students are extra in a row, there would be 1 row less. If 3 students are less in a row there would be 2 rows more. Find the number of students in the class.
Let the number of rows = x
and the number of students in each row = y
∴ Total number of students in the class = xy
According to the I condition,
(y + 3) (x - 1) = xy (no. of students in each row × no. of rows = total students)
implies xy - y + 3x - 3 = xy
implies 3x - y = 3 ...(1)
According to the II condition,
(y - 3) (x + 2) = xy
implies xy + 2y - 3x - 6 = xy
implies 3x - 2y = - 6
Subtracting equation (2) from equation (1), we have
y = 9
Put y = 9 in equation (1), we get
3x - 9 = 3 implies 3x = 12 implies x = 4
∴ Total number of students in the class = xy = 4 × 9 = 36.
Topper's Solved these Questions
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - NAGEEN PRAKASHANCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - Exercise 3a
18 Videos
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - NAGEEN PRAKASHANCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - Exercise 3b
30 Videos
- INTRODUCTION TO TRIGONOMETRYBOOK - NAGEEN PRAKASHANCHAPTER - INTRODUCTION TO TRIGONOMETRYEXERCISE - Revision Exercise Long Answer Questions
5 Videos
- POLYNOMIALSBOOK - NAGEEN PRAKASHANCHAPTER - POLYNOMIALSEXERCISE - Revision Exercise Long Answer Questions
4 Videos
Similar Questions
Students of a class are made to stand in row. If one student is extra in each row, there would be 2 rows less. If one student is less in each row, there would be 3 rows more. Find the number of students in the calss.
View SolutionStudents are made to stand in rows . If one student is extra in a row there would be 2 rows less . If one student is less in a row there would be 3 rows more . Find the number of students in the class .
View Solution
Knowledge Check
In a row of students, Sohan is 9th from either end of the row. Find the number of students in a row.
A10B18C17D196, 8, 12 or 16 rows of the ninth class students are prepared, then no students is left. Find the possible number of students in the class. (a) 60 (b) 72 (c) 80 (d) 96
A60B72C80D96In a row of students Ganesh is 7th from one extreme and 11th from the other. Find the total numbers of students in the row.
A17B18C19D20
Students of a class are made to stand in rows. If one student is extra in a row, there would be two rows less. If one student is less in a row, there would be three rows more. What is the number of students in the class ?
View SolutionStudents of a class are made to stand in rows. If 4 students are extra in each row, then there would be 2 rows less. If four students are less in each row, then there would be 4 more rows. What is the number of students in the class?
View SolutionStudents of a class are preparing for a drill and are made to stand in rows. If 4 students are extra in a row, then there would be 2 rows less. But there would be 4 more rows if 4 students are less in a row. The number of students in the class is (a) 56 (b) 65 (c) 69 (d) 96
View SolutionWhat would be the next row of the numbers ?
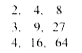
View SolutionIn a row of students Ganesh is 7th from one extreme and 11th from the other. Find the total numbers of students in the row.
View Solution
