The angle of elevation of the top of a tower from a point on the same level as the foot of tower is $ϕ$ . On moving 'a' mctrcs towards the foot of tower, the angle of elevation becomes $45^{\circ}$ and again on moving b metres in the same dorection, the angle of elevation becomes $(90^{\circ} - ϕ)$ . Find the height of the tower.

Text Solution

Verified by Experts

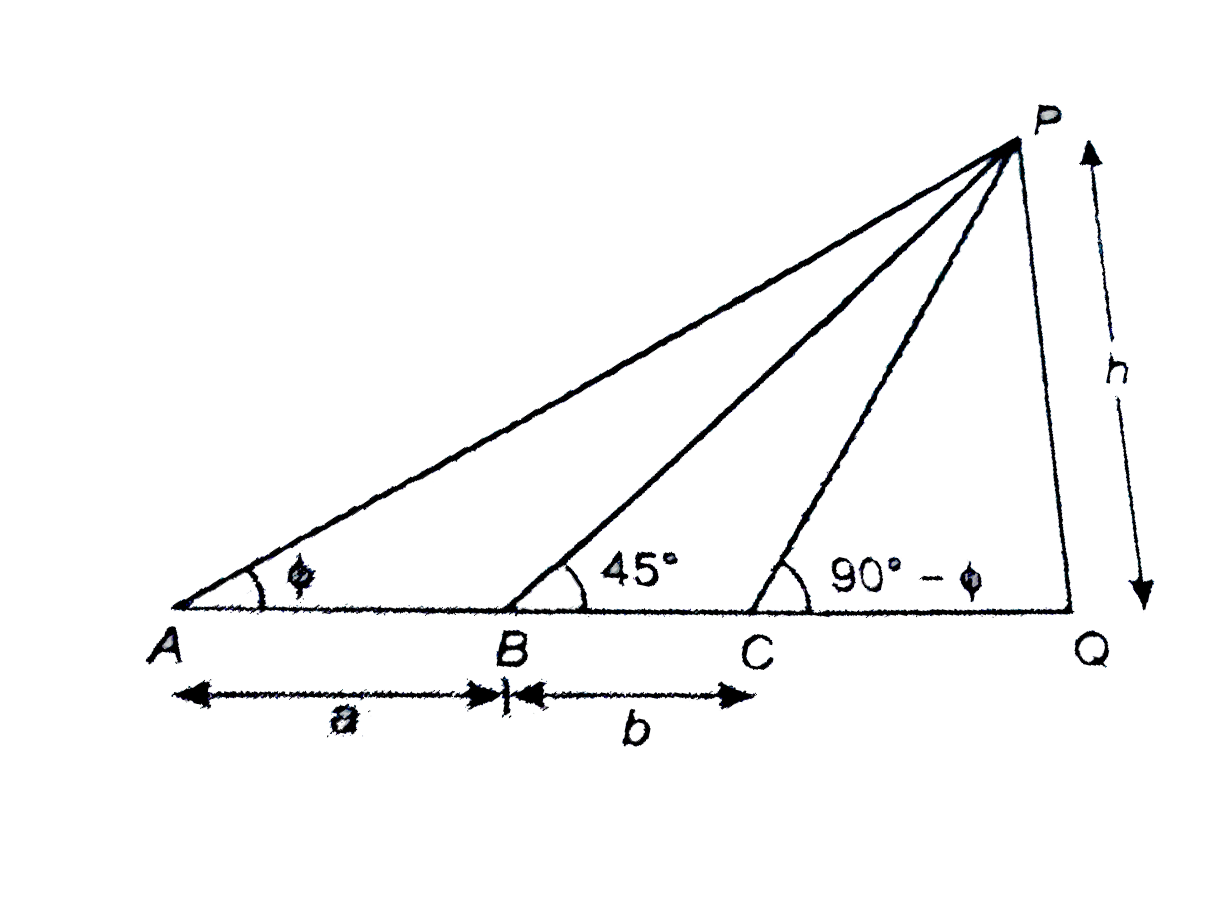
In the figure, PQ is a tower.
Let PQ = h
A, B and C are three points on the line passing through its base Q and $∠ P A Q = ϕ, ∠ P B Q = 90^{\circ} - ϕ$
AB=a and BC = b
In $Δ P B Q$ ,
${tan 45}^{\circ} = \frac{P Q}{B Q}$
$\Rightarrow 1 = \frac{h}{B Q} \Rightarrow B Q = h$
$\Rightarrow b + C Q = h \Rightarrow C Q = h - b$
Now, $A Q = A B + B Q = a + h$
In $Δ P A Q$ ,
$tan ϕ = \frac{P Q}{A Q} = \frac{h}{a + h} ... (1)$
and in $Δ P C Q$
$tan (90^{\circ} - ϕ) = \frac{P Q}{C Q}$
$\Rightarrow cot ϕ = \frac{h}{h - b} \Rightarrow tan ϕ = \frac{h - b}{h} ... (2)$
From equations (1) and (2)
$\frac{h}{a + h} = \frac{h - b}{h} \Rightarrow h^{2} = h a - a b + h^{2} - b h$
$\Rightarrow h (a - b) = a b \Rightarrow h = \frac{a b}{a - b}$
$∴ Height of tower = \frac{a b}{a - b}$

Updated on:21/07/2023