The angle of elevation of the top of a tower from a point 40 m away from its foot is 60∘. Find the height of the tower.
The correct Answer is:40√3m
To find the height of the tower using the given information, we can follow these steps:
Step 1: Understand the problem
We have a tower and a point from which the angle of elevation to the top of the tower is given. We need to find the height of the tower.
Step 2: Draw a diagram
Let's denote:
- The height of the tower as h.
- The distance from the point to the foot of the tower as d=40 m.
- The angle of elevation as θ=60∘.
Step 3: Identify the right triangle
From the point at a distance of 40 m from the foot of the tower, we can form a right triangle:
- The height of the tower h is the opposite side.
- The distance from the point to the foot of the tower (40 m) is the adjacent side.
- The angle of elevation θ is at the point.
Step 4: Use the tangent function
In a right triangle, the tangent of an angle is the ratio of the opposite side to the adjacent side. Therefore, we can write:
tan(θ)=OppositeAdjacent=hd
Substituting the known values:
tan(60∘)=h40
Step 5: Calculate tan(60∘)
We know that:
tan(60∘)=√3
So we can substitute this into our equation:
√3=h40
Step 6: Solve for h
To find the height h, we can rearrange the equation:
h=40⋅√3
Step 7: Calculate the numerical value
Using the approximate value of √3≈1.732:
h≈40⋅1.732≈69.28 m
Final Answer
The height of the tower is approximately 69.28 meters.
---
We have a tower and a point from which the angle of elevation to the top of the tower is given. We need to find the height of the tower.
Let's denote:
- The height of the tower as h.
- The distance from the point to the foot of the tower as d=40 m.
- The angle of elevation as θ=60∘.
From the point at a distance of 40 m from the foot of the tower, we can form a right triangle:
- The height of the tower h is the opposite side.
- The distance from the point to the foot of the tower (40 m) is the adjacent side.
- The angle of elevation θ is at the point.
In a right triangle, the tangent of an angle is the ratio of the opposite side to the adjacent side. Therefore, we can write:
tan(θ)=OppositeAdjacent=hd
Substituting the known values:
tan(60∘)=h40
We know that:
tan(60∘)=√3
So we can substitute this into our equation:
√3=h40
To find the height h, we can rearrange the equation:
h=40⋅√3
Using the approximate value of √3≈1.732:
h≈40⋅1.732≈69.28 m
The height of the tower is approximately 69.28 meters.
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHANCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Revision Exercise (long Answer Questions)1videos
SOME APPLICATIONS OF TRIGONOMETRY
- BOOK - NAGEEN PRAKASHANCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Long Answer Questions5videos
SOME APPLICATIONS OF TRIGONOMETRY
- BOOK - NAGEEN PRAKASHANCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Exercise32videos
SOME APPLICATIONS OF TRIGONOMETRY
- BOOK - NAGEEN PRAKASHANCHAPTER - REAL NUMBERSEXERCISE - Revision Exercise Long Answer Questions5videos
REAL NUMBERS
- BOOK - NAGEEN PRAKASHANCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions5videos
STATISTICS
Similar Questions
If the angle of elevation of the top of a tower from a point distant 100 m from its base is 45∘, then find the height of the tower.
View SolutionIn figure , the angle of elevation of the top of a tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m, Find the distance of the point from the foot of the power .
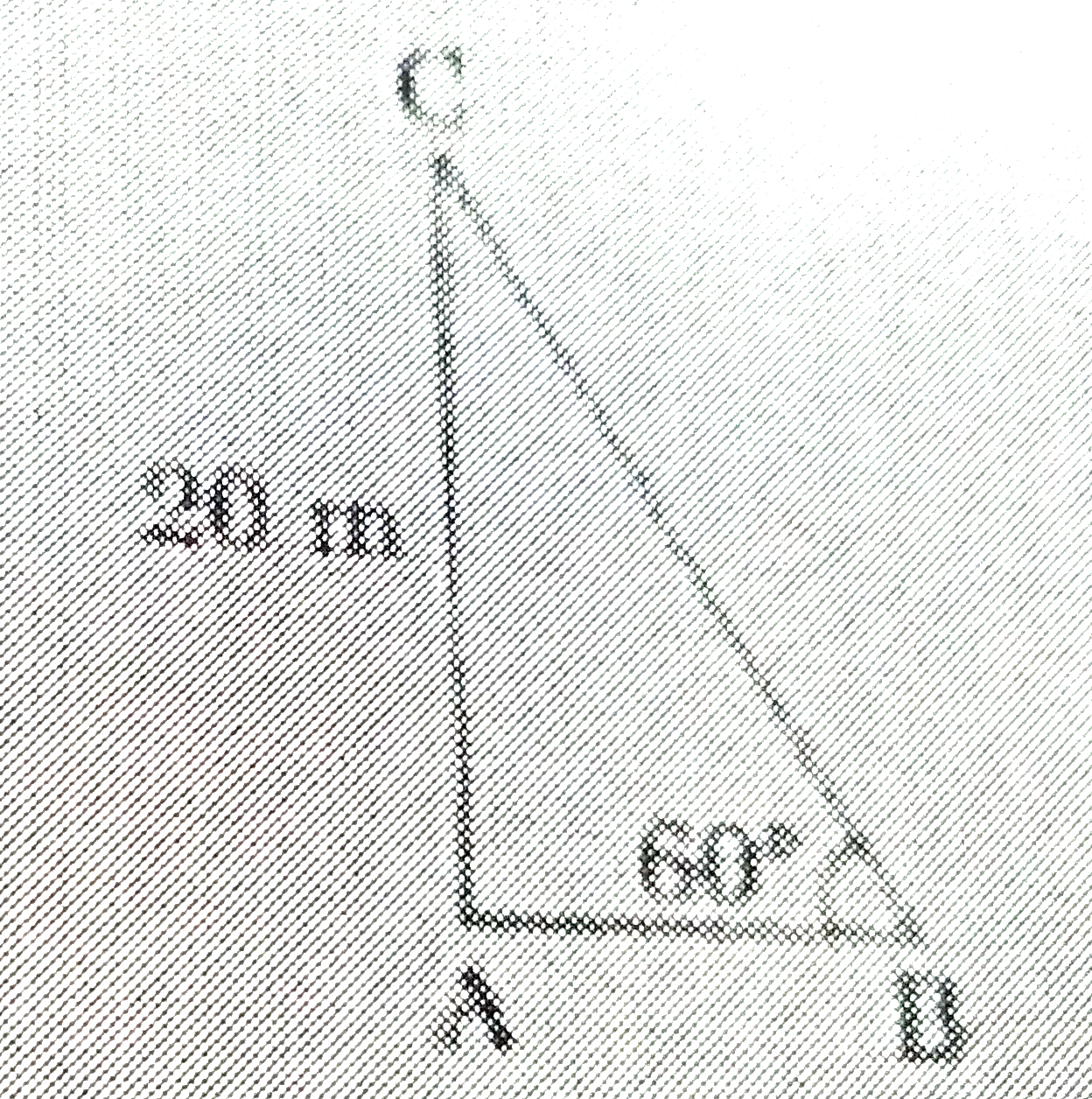 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
The angle of elevation of the top of a tower from a point 20 m away from its base is 45∘.What is the height of the tower?
A10 mB20mC30mD40m - Question 2 - Select One
The angle of elevation of the top of a tower form a point 20 m away from its base is 45∘. What is the height of the tower?
A10 mB20mC30mD40m - Question 3 - Select One
The angle of elevation of the top of a tower from a point 20 metres away from its base is 45∘. The height of the tower is
A10 mB20mC30mD40m
In the figure-2, the angle of elevation of the top of tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m. find the distance of the point from the foot of the tower.
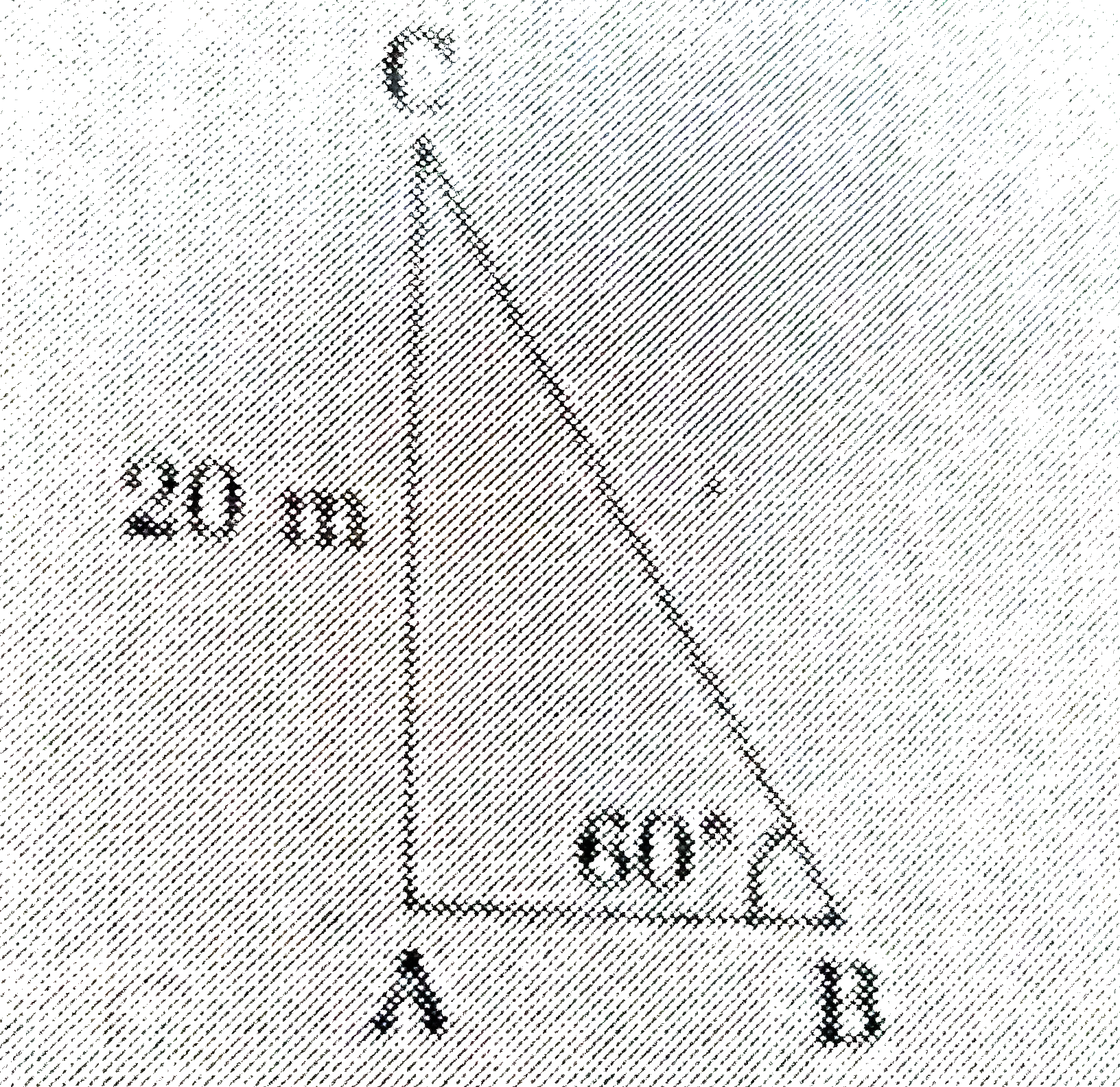 View Solution
View SolutionIn figure, the angle of elevation of the top of a tower AC from a point B on the ground is 60∘. If the height of the tower is 20m , find the distance of the point from the foot of the tower.
View SolutionThe angle of elevation of the top of an incomplete tower, at a point 40m away from its foot, is 45∘. How much more high the tower must be constructed so that the angle of elevation of its top at the same point be 45∘?
View SolutionThe angle of elevation of the top of a lower at a distance of 25 m from its foot is 60∘ The approximate height of the tower is -
View SolutionThe angle of elevation of the top of a 15 meters high tower from a point 15 metres aways from its foot is:
View Solution

