What must be added to $x^{4} + 2 x^{3} - 2 x^{2} - 2 x - 1$ to obtain a polynomial which is exactly divisible by $(x^{2} + 2 x - 3)$ ?

Video Solution

Text Solution

Verified by Experts

Let $p (x) = x^{4} + 2 x^{3} - 2 x^{2} - 2 x - 1$
and $g (x) = x^{2} + 3 x - 3 = (x - 1) (x + 3)$
$x^{2} + 1$

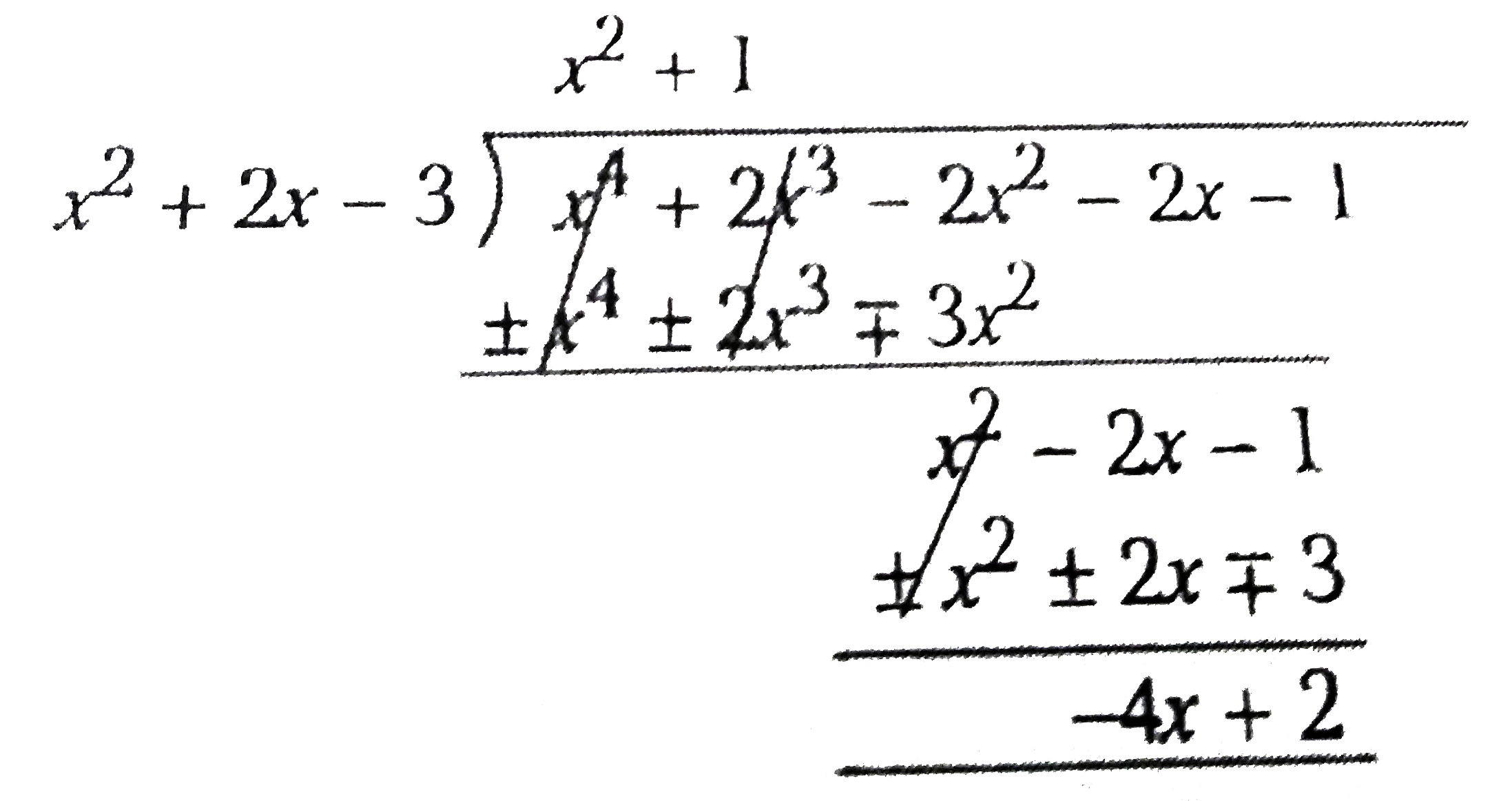
On actual division, we see that remainder (extra) is $- 4 x + 2$ . So, if we subtract $- 4 x + 2$ or add $- (- 4 x + 2)$ i.e., 4x-2 to p(x), then remainder vanishes, therefore p(x) will be exactly divisible by $x^{2} + 2 x - 3$ .
Hence, (4x-2) is added to the given polynomial p(x).
Alternative Method : Since p(x) is divided by a polynomial g(x) of degree 2, therefore we will get a remainder of degree 1.
Let (ax+b) be added to p(x) to get
$h (x) = (x^{4} + 2 x^{3} - 2 x^{2} - 2 x - a) + (a x + b)$
If equation (1) is divisible by $x^{2} + 2 x - 3 or (x - 1) (x + 3)$
$∴ h (- 3) = 0$ and h(1)=0
$\Rightarrow {(- 3)}^{4} + 2 {(- 3)}^{3} - 2 {(- 3)}^{2} - 2 (- 3) - 1 + a (- 3) + b = 0$
$\Rightarrow 81 - 54 - 18 + 6 - 1 - 3 a + b = 0 \Rightarrow - 3 a + b = - 14$
Also h(1)=0
$\Rightarrow {(1)}^{4} + 2 {(1)}^{3} - 2 {(1)}^{2} - 2 (1) - 1 + a (1) + b = 0$
$\Rightarrow 1 + 2 - 2 - 2 - 1 + a + b = 0 \Rightarrow a + b = 2$
Subtracting (3) from (2), we get
$- 4 a = - 16 \Rightarrow a = 4$
Put this value of a in (3), we get
b=-2
So, the required polynomial to be added =ax+b=4x-2.

Updated on:21/07/2023

What must be added to $(x^{3} - 3 x^{2} + 4 x - 15)$ to obtain a polynomial which is exactly divisible by $(x - 3)$ ?
A $2$
B $3$
C $- 3$
D $- 2$
What must be sutracted from $(x^{3} + 4 x^{2} - 6 x - 14)$ to obtain a polynomial exactly divisible by $(x^{2} - x - 2)$ ?
A $x + 8$
B $x - 4$
C $x + 4$
D $2 x + 4$
What must be added to $2 x^{4} - 5 x^{3} + 2 x^{2} - x - 3$ so that the result is exactly divisible by ( x-2 ) ?
A-5
B4
C5
D-4