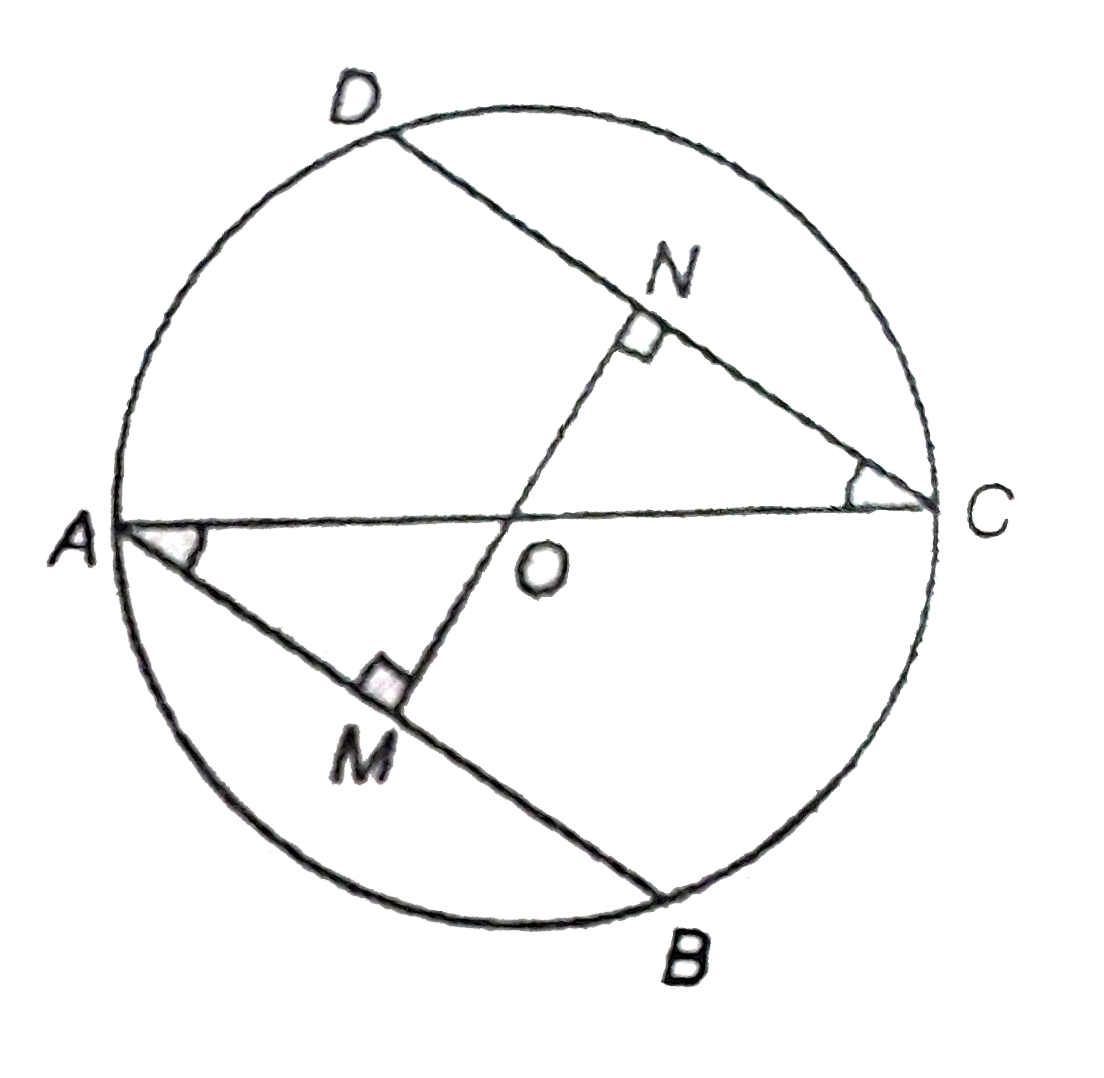
AB and CD are two parallel chords of a circle whose diameter is AC . Prove that AB=CD
In the figure O is centre of the circle.
Draw OM⊥AB and ON⊥CD.
In ΔOAM and ΔOCN, we have
∵ ⎧⎪⎨⎪⎩∠OAM=∠OCN(alternate angles)∠OAM=∠ONC(given=90∘)OA=OC(radii of same circle)
∴ΔOAM≅ΔOCN (AAS congurency
AM=CN (c.p.c.t)
2AM=2CN
⇒AB=CD (because Perpendiculars from centre bisect the chords)
Similar Questions
AB and CD are two parallel chords of a circle with centre O such that AB=6 cm and CD=12 cm. The chords are on the same side of the centre and the distance between them is 3 cm. The radius of the circle is 6 cm
View SolutionLet AB and CD are two parallel chords of circle whose radius is 5 units. If P and Q are mid points of AB and CD respectively such that PA.PB=9,QC.QD=16, then distance betweẹn AB and CD is
View SolutionAB and CD are two chords of a circle such that AB=6 cm, CD=12 cm and ABCD. If the distance between AB and CD is 3 cm, find the radius of the circle.
View SolutionIf AB, BC and CD are equal chords of a circle with O as centre and AD diameter, then ∠AOB= 600 (b) 900 (c) 1200 (d) none of these
View SolutionThe sides AB and CD of a parallelogram ABCD are bisected at E and F. Prove that EBFD is a parallelogram.
View SolutionIn Figure ABCDandAEFD are two parallelograms. Prove that: PE=FQ

View SolutionAB and CD are two chords of a circle such that AB = 6 cm, CD = 12 cm and AB || CD. If the distance between AB and CD is 3 cm, find the radius of the circle
View SolutionIn Figure, BM and DN are both perpendiculars to the segments AC and BM=DN . Prove that AC bisects BD
View SolutionIn Fig. 15.69, AB and CD are two diameters of a circle perpendicular to each other and OD is the diameter of the smaller circle. If OA=7 cm , find the area of the shaded region. (FIGURE)
View SolutionTwo circle with centres A and B intersect at C and D. Prove that ∠ACB=∠ADB.
View Solution
