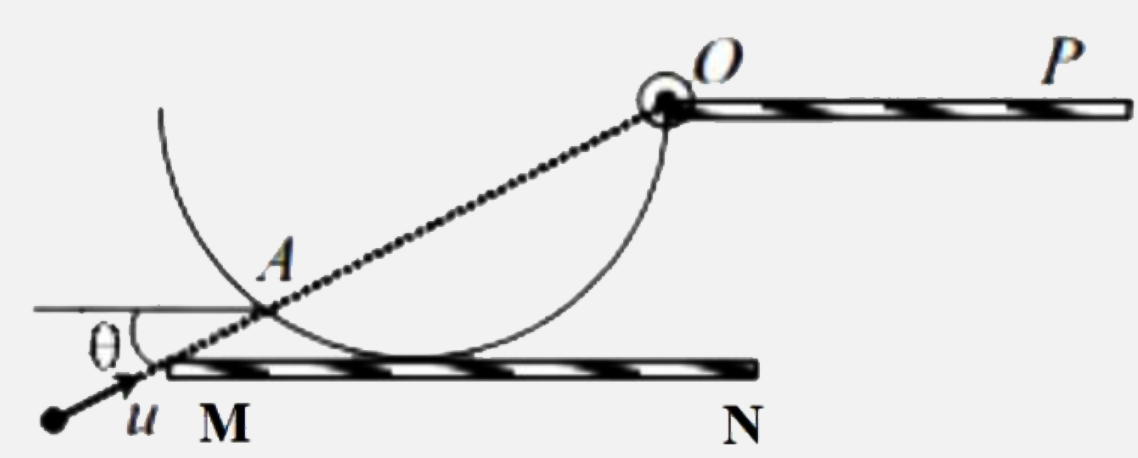
The diagram shows a hemispherical shell of mass m and radius R is hinged at point of placed on a horizontal surface. A ball of mass strikes the shell at point A ( as shown in the figure ) moving with velocity u inclined at an angle θ=tan−1(12) and then it stops . For the given shell to reach horizontal surface OP what minimum speed u is required ?
2√gR3
√2gR3
gR√5
Not possible
The correct Answer is:D
Step by step video & image solution for The diagram shows a hemispherical shell of mass m and radius R is hinged at point of placed on a horizontal surface. A ball of mass strikes the shell at point A ( as shown in the figure ) moving with velocity u inclined at an angle theta = tan^(-1) (1/2) and then it stops . For the given shell to reach horizontal surface OP what minimum speed u is required ? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
A force F acts at the centre of a thin spherical shell of mass m and radius R.
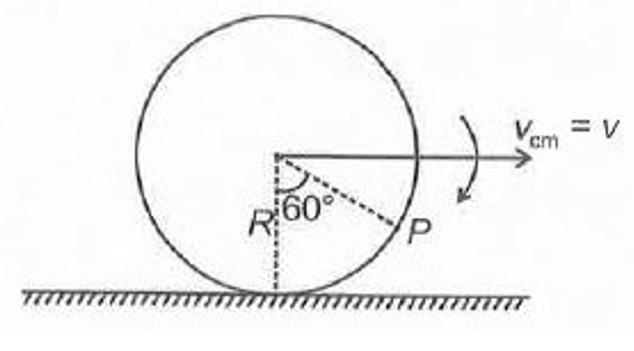
Find the acceleration of the shell if the surface is smooth.View SolutionA cycle tyre of mass M and radius R is in pure rolling over a horizontal surface. If the velocity of the centre of mass of the tyre is v, then the velocity of point P on the tyre, shown in figure is
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
The distance of the centre of mass of a hemispherical shell of radius R from its centre is
AR2BR3C2R2D2R3 - Question 2 - Select One
A particle of mass m is placed at the centre of a unifrom spherical shell of mass 3 m and radius R The gravitational potential on the surface of the shell is .
A−GmRB−3GmRC−4GmRD−2GmR - Question 3 - Select One
A shell of mass M and radius R has point mass m placed at a distance r from its centre. The gravitational potential energy U(r) vs r will be
A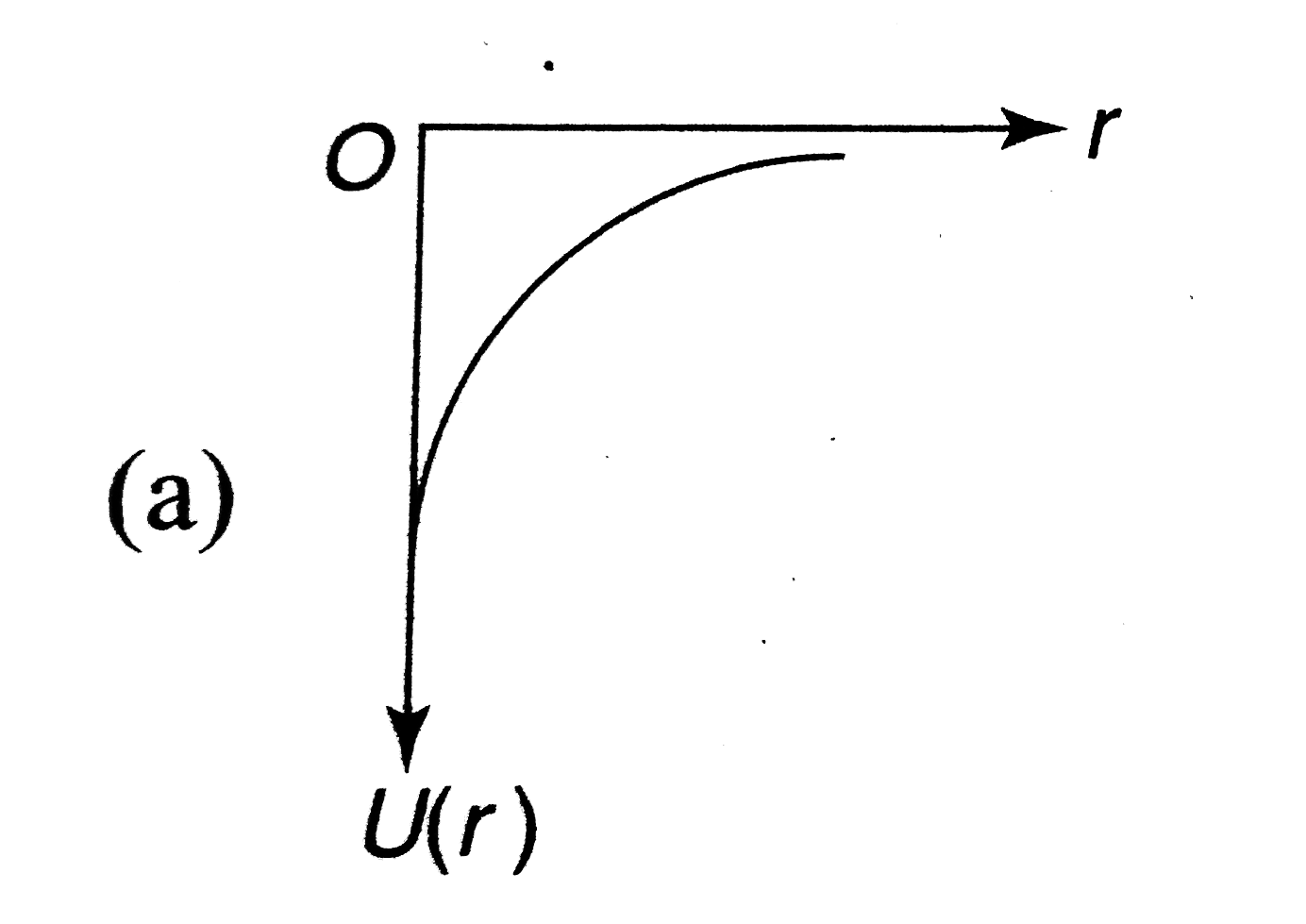 B
B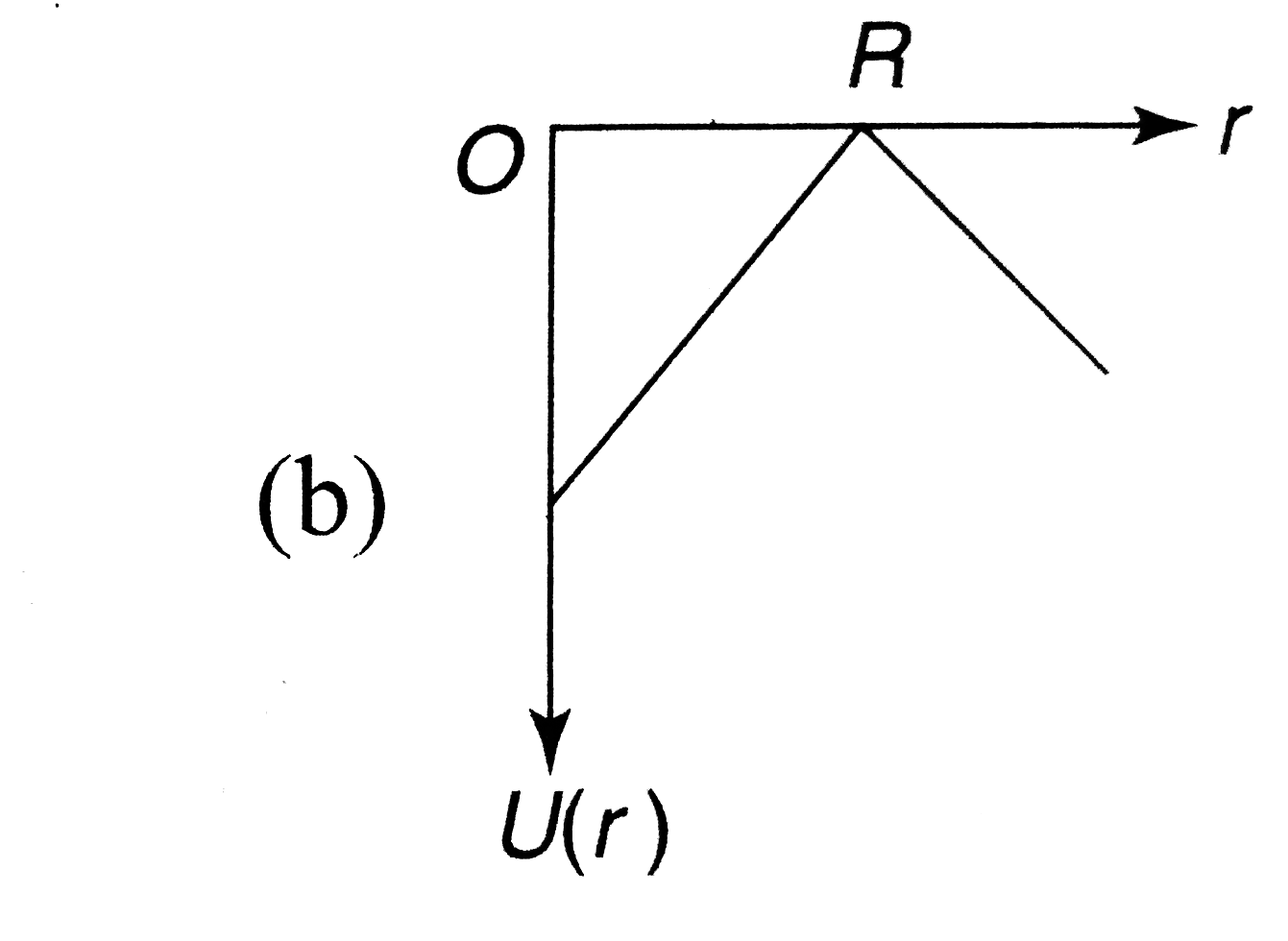 C
C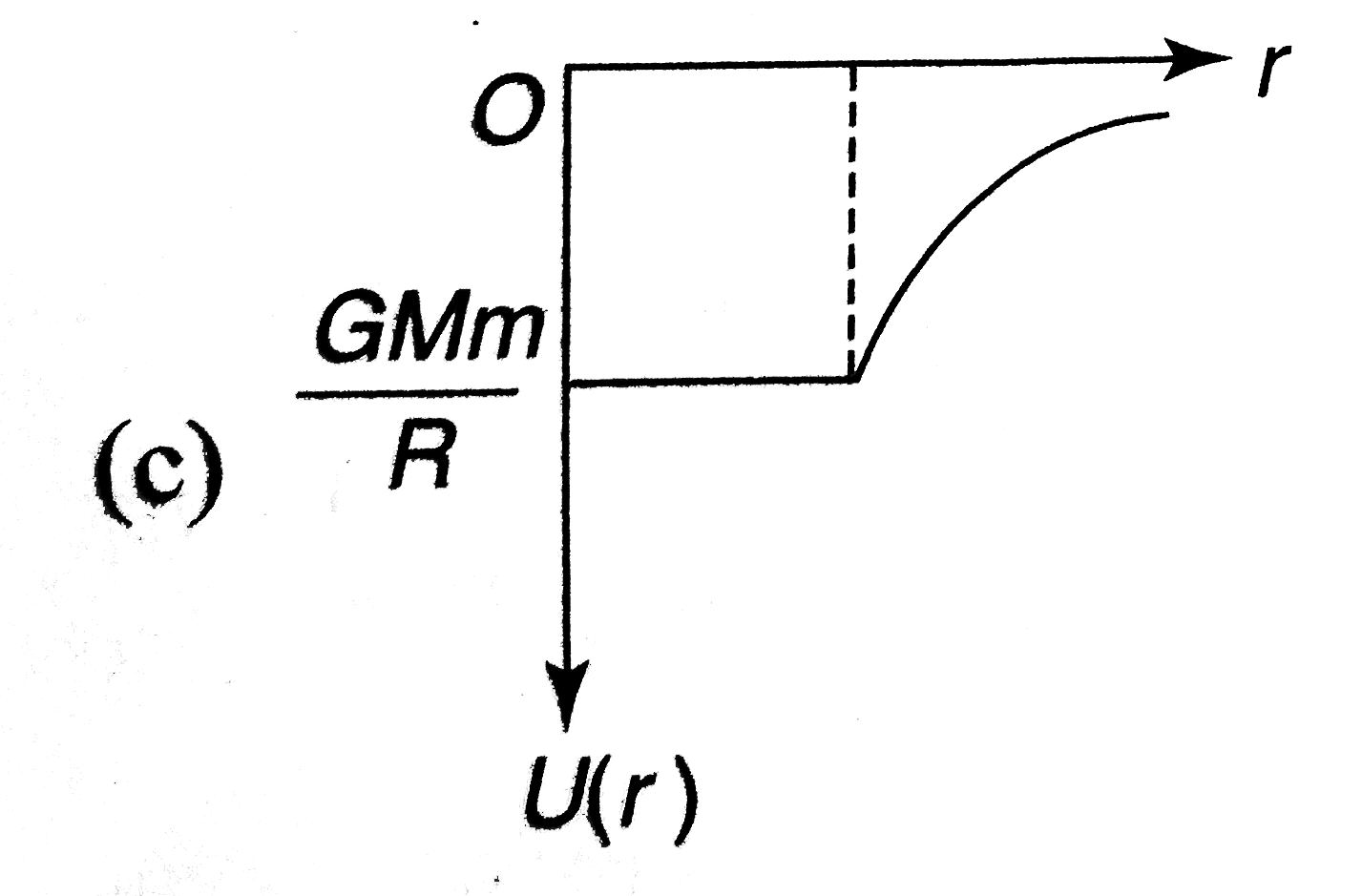 D
D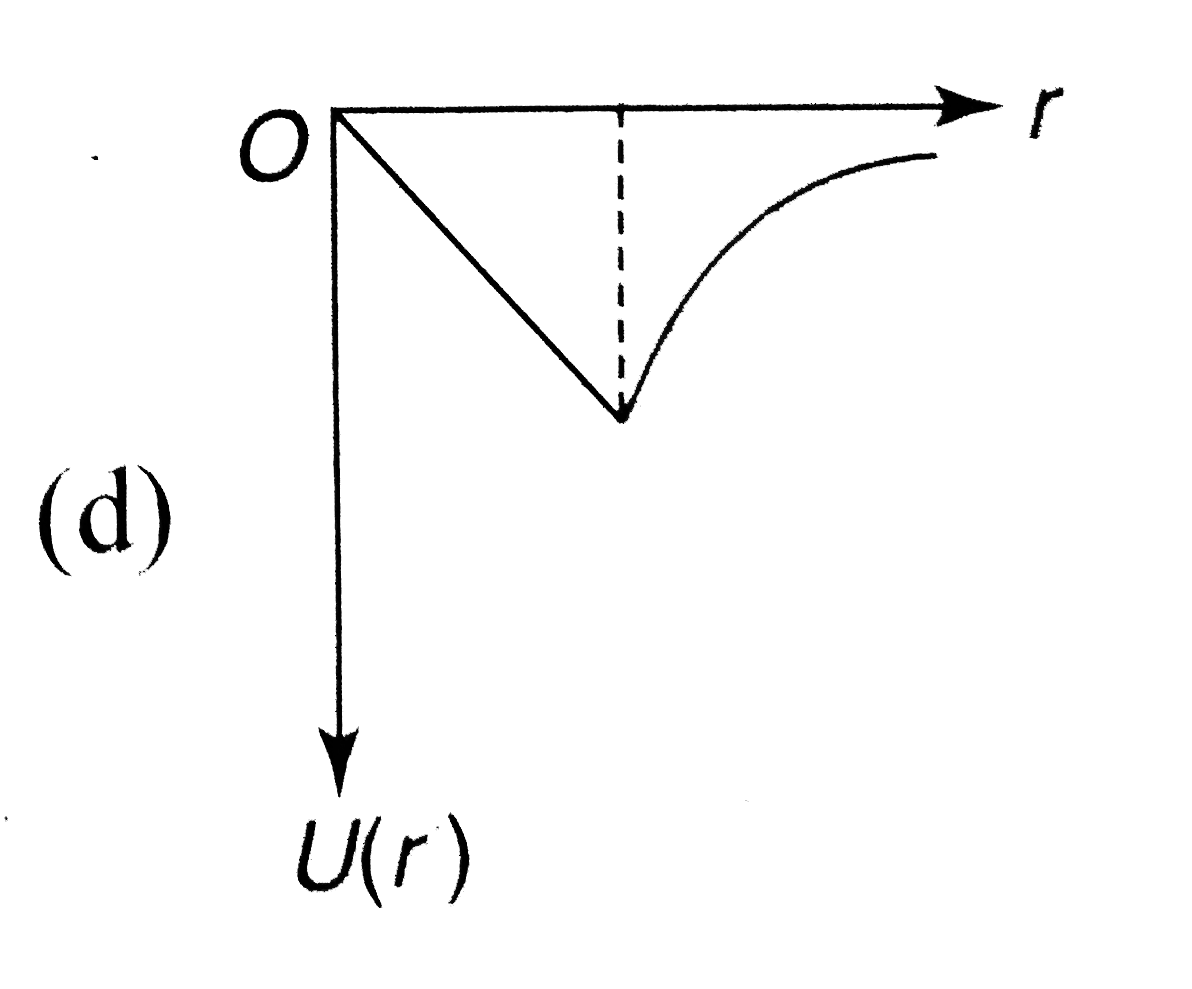
A thin spherical shell of radius R lying on a rough horizontal surface is hit sharply and horizontally by a cue. Where should it be hit so that the shell does not slip on the surface?
View SolutionThe figure shows a spherical shell of mass M. The point a is not at the centre but away from the centre of the shell. If a particle of mass m is placed at A, then
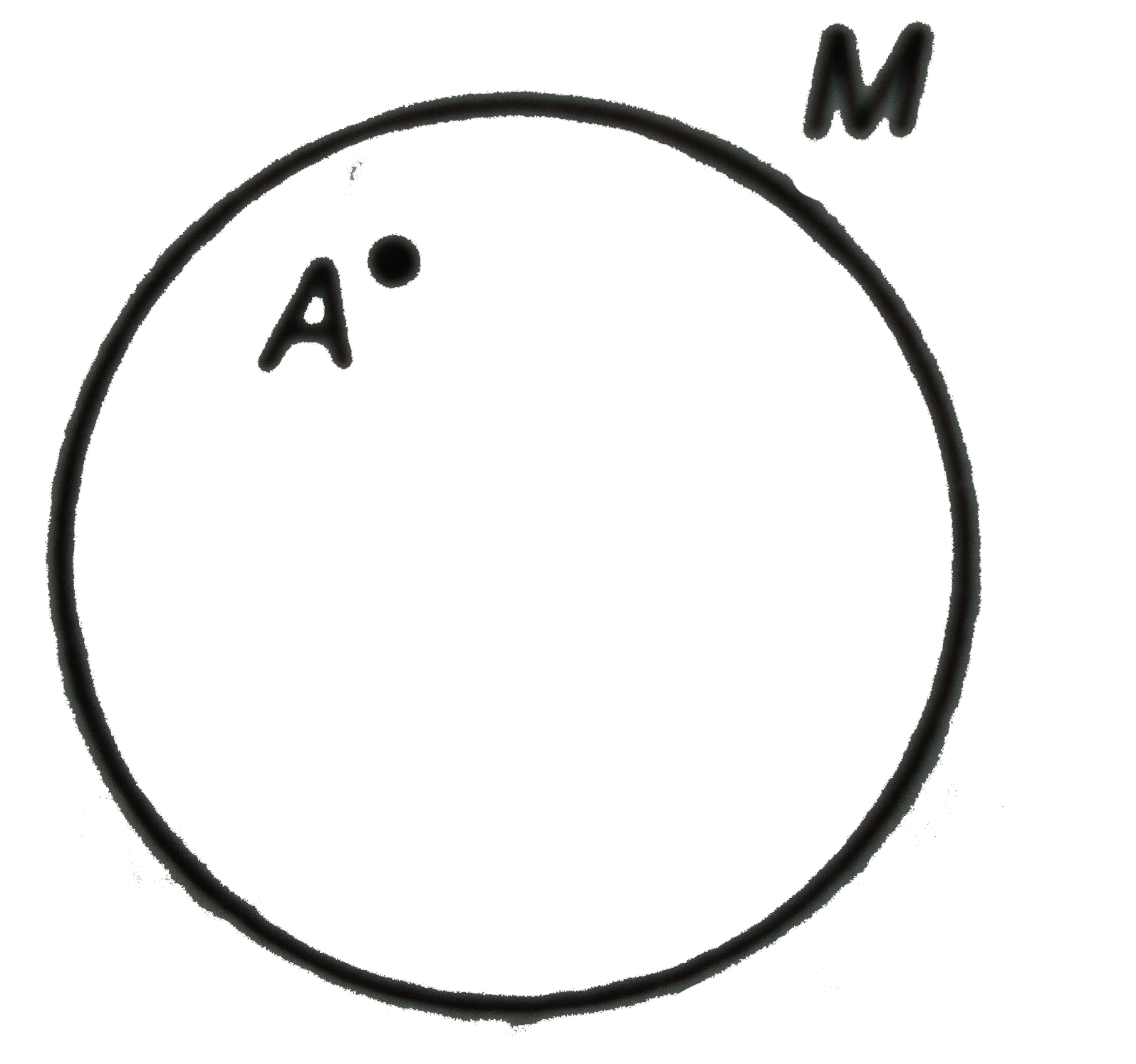 View Solution
View SolutionA particel of mass M is placed at the centre of a inform spherical shell of mass 2M and radius R. The gravitational potential on the surface of the shell is
View SolutionA shell of mass M and radius R has another point mass m placed at a distance r from its centre (r>R). The force of attraction between the shell and point mass is :
View SolutionFigure show a hemispherical shell having uniform mass density The direction of gravitational field at point P will be along
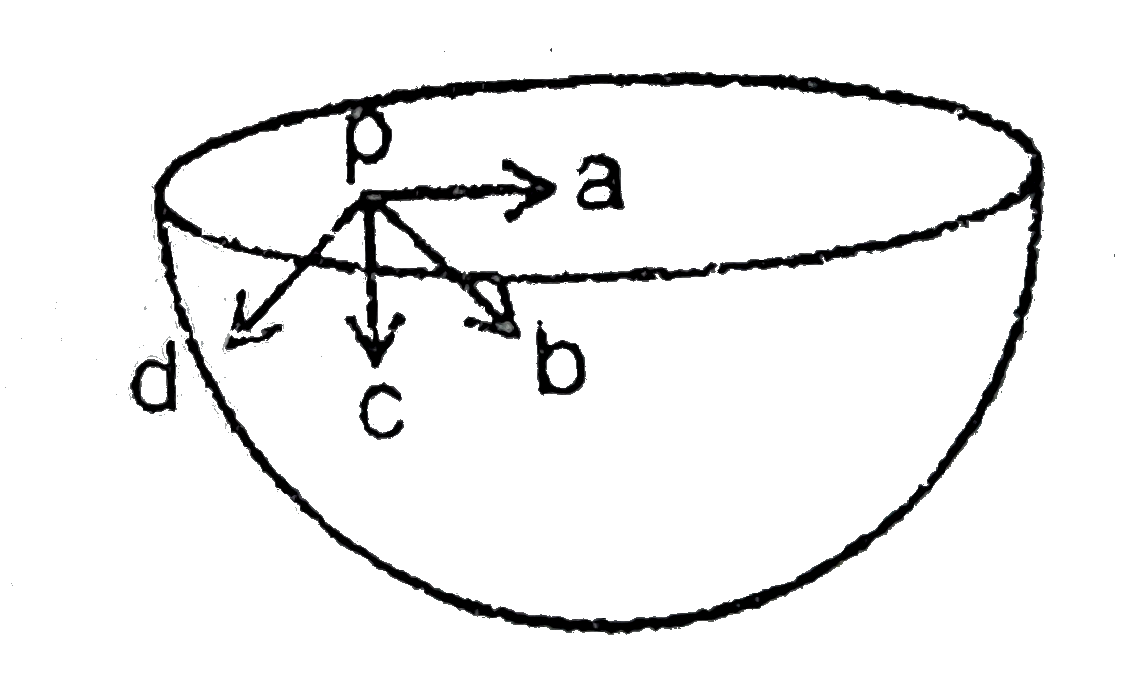 .View Solution
.View Solution

