A chance Q placed at thee center of a metallic spherical shell with inner and outer radii $R_{1} and R_{2}$ respectively . The normal component of the electric field at any point on the Gaussian surface with radius between $R_{1} and R_{2}$ will be

Zero

$\frac{Q}{4 π R_{1}^{2}}$

$\frac{Q}{4 π R_{2}^{2}}$

$\frac{Q}{4 π {(R_{1} - R_{2})}_{1}^{2}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Updated on:21/07/2023

Answer

Step by step video, text & image solution for A chance Q placed at thee center of a metallic spherical shell with inner and outer radii R _1 and R_2 respectively . The normal component of the electric field at any point on the Gaussian surface with radius between R_1and R_2 will be by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Knowledge Check

A positive charge $Q$ is placed at the centre $O$ of a thin metalic spherical shell. Select the correct statements from the following:
(i) The electric field at any point outside the shell is zero
(ii) The electrostatic potential at any point outside the shell is $\frac{Q}{4 π ε_{0} r}$ , where $r$ is the distance of the point from $O$
(iii) The outer surface of the spherical shell is an equipotential surface
(iv) The electric field at any point inside the shell , other than $O$ , is zero
A $(i), (i i)$
B $(i i), (i i i)$
C $(i), (i v)$
Dall
There are three concentric metallic spherical shells of radii R, 2R and 3q, respectively. The middle spherical shell is connected to earth. Let $q_{1}$ be the charge appearing on the inner surface of the middle sphere and $q_{2}$ be the charge on outer surface of the middle sphere. Similarly $q_{3}$ is the charge appearing on inner surface of outermost sphere and $q_{4}$ is the charge appearing on outer surface of outermost sphere.

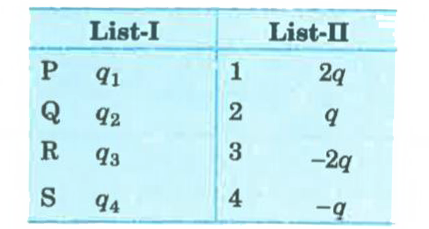A $\begin{matrix} P & Q & R & S 4 & 3 & 1 & 2 \end{matrix}$
B $\begin{matrix} P & Q & R & S 4 & 3 & 2 & 1 \end{matrix}$
C $\begin{matrix} P & Q & R & S 3 & 4 & 1 & 2 \end{matrix}$
D $\begin{matrix} P & Q & R & S 3 & 4 & 2 & 1 \end{matrix}$
A point charge $Q$ is located at the centre of a hollow spherical conductor having inner radius as $R_{1}$ and outer radius $R_{2}$ . The conductor being uncharged initially. The potential at the inner surface will be
A $K Q [\frac{1}{R_{1}} + \frac{1}{R_{2}}]$
B $K Q [\frac{1}{R_{1}} - \frac{1}{R_{2}}]$
C $K Q [\frac{1}{R_{2}} - \frac{1}{R_{1}}]$
Dnone of these

NTA MOCK TESTS-NTA NEET SET 83-PHYSICS

Home
Ask Doubt
Profile

A chance Q placed at thee center of a metallic spherical shell with inner and outer radii R1andR2 respectively . The normal component of the electric field at any point on the Gaussian surface with radius between R1andR2 will be

The correct Answer is:A

Topper's Solved these Questions

Similar Questions

Knowledge Check

A point charge Q is located at the centre of a hollow spherical conductor having inner radius as R1 and outer radius R2. The conductor being uncharged initially. The potential at the inner surface will be

NTA MOCK TESTS-NTA NEET SET 83-PHYSICS

A chance Q placed at thee center of a metallic spherical shell with inner and outer radii $R_{1} and R_{2}$ respectively . The normal component of the electric field at any point on the Gaussian surface with radius between $R_{1} and R_{2}$ will be

A point charge $Q$ is located at the centre of a hollow spherical conductor having inner radius as $R_{1}$ and outer radius $R_{2}$ . The conductor being uncharged initially. The potential at the inner surface will be