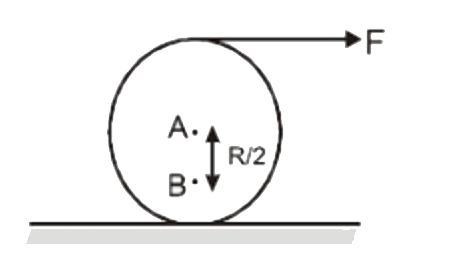
A disc of mass m and radius R, is placed on a smooth text service fixed surface . Point A is the geometrical centre of the disc while point B is the centre of mass of the disc . The moment of inertia of the dics about an axis through its centre of mass and perpendicular to the plane of the figure is I .A constant force F is applied to the top of the disc . The acceleration of the centre A of the disc at the instant B is below A (on the same vertical line ) will be :

$\frac{F}{m}$

$\frac{F}{m} + \frac{3 F R^{2}}{4 l}$

$\frac{F}{m} + \frac{F R^{2}}{4 l}$

$\frac{F}{m} + \frac{3 F R^{2}}{l}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Updated on:21/07/2023

Answer

Step by step video, text & image solution for A disc of mass m and radius R, is placed on a smooth text service fixed surface . Point A is the geometrical centre of the disc while point B is the centre of mass of the disc . The moment of inertia of the dics about an axis through its centre of mass and perpendicular to the plane of the figure is I .A constant force F is applied to the top of the disc . The acceleration of the centre A of the disc at the instant B is below A (on the same vertical line ) will be : by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Knowledge Check

The moment of inertia of a copper disc, rotating about an axis passing through its centre and perpendicular to its plane
Aincreases if its temperature is increased
Bchanges if its axis of rotation is changed
Cincreases if its angular velocity is increased
Dboth (a) and (b) are correct
Moment of inertia of a uniform quarter disc of radius $R$ and mass $M$ about an axis through its centre of mass and perpendicular to its plane is $:$
A $\frac{M R^{2}}{2} - M {(\frac{4 R}{3 π})}^{2}$
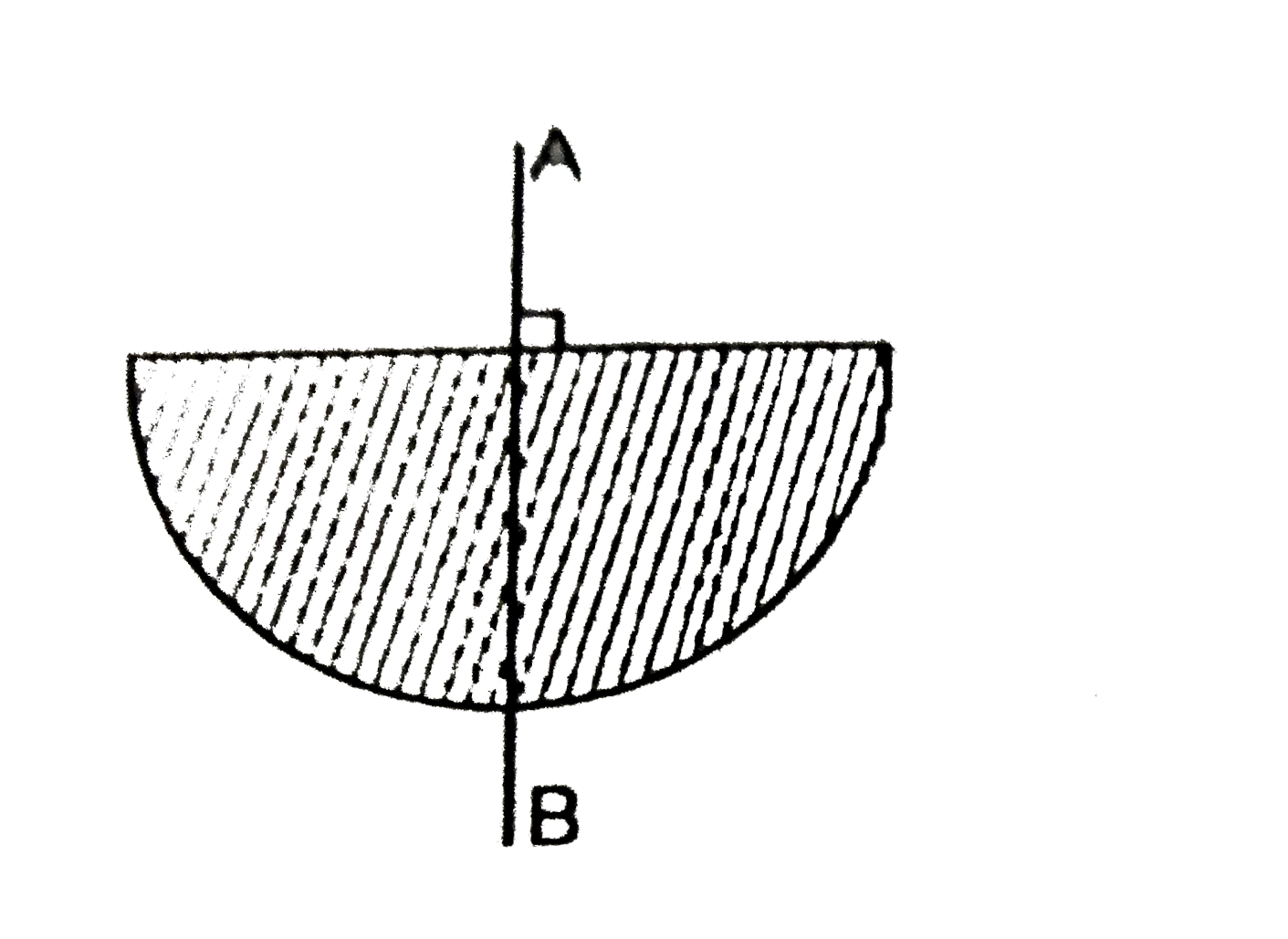
B $\frac{M R^{2}}{2} - M {(\sqrt{2} \frac{4 R}{3 π})}^{2}$
C $\frac{M R^{2}}{2} + M {(\frac{4 R}{3 π})}^{2}$
D $\frac{M R^{2}}{2} + M {(\sqrt{2} \frac{4 R}{3 π})}^{2}$
A uniform semicircular disc of mass $' m'$ and radius $' R'$ is shown in the figure. Find out its moment of inertia about.
$(a)$ axis $' A B' ($ shown in the figure $0$ which passes through geometrical centre and lies in the plane of the disc
$(b)$ axis $' C D'$ which passes through its centre of mass and it is perpendicular to the plane of the disc.

A $\frac{M R^{2}}{2} - M {(\frac{2 R}{π})}^{2}$
B $\frac{M R^{2}}{2} - M {(\frac{4 R}{π})}^{2}$
C $\frac{M R^{2}}{2} + M {(\frac{4 R}{3 π})}^{2}$
D $\frac{M R^{2}}{2} + M {(\frac{2 R}{π})}^{2}$