Question

A ray of light suffers a minimum deviation when incident on equilateral prism of refractive index $\sqrt{2}$ The angle of deviation is

Options

A. `30^@`
B. `45^@`
C. `60^@`
D. `50^@`

Answer

A

Explanation

To solve the problem of finding the angle of minimum deviation when a ray of light passes through an equilateral prism with a refractive index of $$\sqrt{2}$$, we can follow these steps:

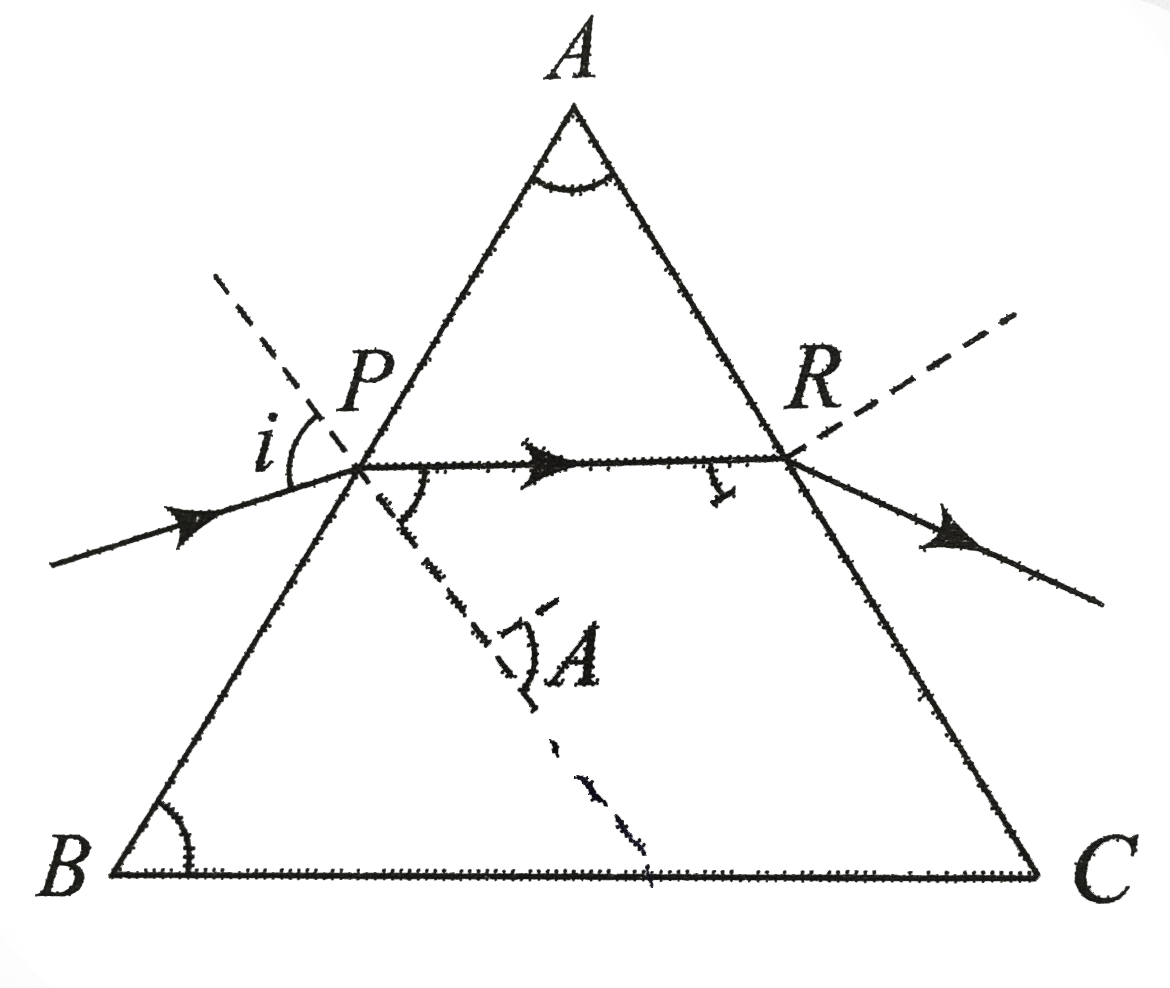
Step 1: Understand the properties of an equilateral prism
An equilateral prism has three equal angles, each measuring \(60^\circ\). The angle of the prism is denoted as \(A\), which is \(60^\circ\) in this case.

Step 2: Recall the condition for minimum deviation
In the condition of minimum deviation, the angle of incidence \(i\) is equal to the angle of emergence \(e\). This means that the light ray enters and exits the prism symmetrically.

Step 3: Use the formula for refractive index
The refractive index \(n\) of the prism can be related to the angle of the prism \(A\) and the minimum deviation \(\delta_m\) using the formula:
\( n = \frac{\sin\left(\frac{A + \delta_m}{2}\right)}{\sin\left(\frac{A}{2}\right)} \)
Here, \(A = 60^\circ\).

Step 4: Substitute the known values into the formula
Given that \(n = \sqrt{2}\) and \(A = 60^\circ\), we can substitute these values into the formula:
\( \sqrt{2} = \frac{\sin\left(\frac{60^\circ + \delta_m}{2}\right)}{\sin\left(\frac{60^\circ}{2}\right)} \)
Calculating \(\sin\left(\frac{60^\circ}{2}\right)\):
\( \sin\left(30^\circ\right) = \frac{1}{2} \)
Thus, the equation becomes:
\( \sqrt{2} = \frac{\sin\left(30^\circ + \frac{\delta_m}{2}\right)}{\frac{1}{2}} \)
This simplifies to:
\( \sqrt{2} = 2 \sin\left(30^\circ + \frac{\delta_m}{2}\right) \)
\( \sin\left(30^\circ + \frac{\delta_m}{2}\right) = \frac{\sqrt{2}}{2} \)

Step 5: Solve for \(\delta_m\)
The value of \(\sin\left(45^\circ\right) = \frac{\sqrt{2}}{2}\), so we set:
\( 30^\circ + \frac{\delta_m}{2} = 45^\circ \)
Subtracting \(30^\circ\) from both sides gives:
\( \frac{\delta_m}{2} = 15^\circ \)
Multiplying both sides by 2 results in:
\( \delta_m = 30^\circ \)

Conclusion
The angle of minimum deviation \(\delta_m\) when a ray of light passes through an equilateral prism of refractive index \(\sqrt{2}\) is \(30^\circ\).