The mass of a pendulum bob is m = 2kg and the length of the string is l. It is displaced through 90∘ from the vertical and released. What minimum tension (in N) should the string should be able to withstand so that it doesn't break during motion?
The correct Answer is:60
To solve the problem of finding the minimum tension that the pendulum string should withstand, we can follow these steps:
1. Identify the Parameters:
- Mass of the pendulum bob, m=2kg
- Length of the string, l (not specified, but it will cancel out later)
- Angle of displacement from the vertical, θ=90∘
2. Determine the Forces Acting on the Bob:
- When the bob is at the lowest point of its swing, the forces acting on it are:
- The gravitational force acting downward, Fg=mg
- The tension in the string, T, acting upward.
3. Centripetal Force Requirement:
- At the lowest point, the bob is moving in a circular path, so it requires a centripetal force, Fc, given by:
Fc=mv2l
- Here, v is the speed of the bob at the lowest point.
4. Apply Newton's Second Law:
- At the lowest point, the net force acting on the bob is the difference between the tension and the gravitational force:
T−mg=Fc
- Rearranging gives:
T=mg+mv2l
5. Use Conservation of Energy:
- The potential energy lost by the bob when it swings down from the height h (which is equal to the length of the string l when displaced by 90∘) is converted into kinetic energy:
mgh=12mv2
- Since h=l, we can write:
mgl=12mv2
- Solving for v2:
v2=2gl
6. Substitute v2 Back into the Tension Equation:
- Substitute v2 into the tension equation:
T=mg+m(2gl)l
- This simplifies to:
T=mg+2mg=3mg
7. Calculate the Tension:
- Now substituting m=2kg and g=10m/s2:
T=3×2×10=60N
Final Answer:
The minimum tension that the string should be able to withstand is 60 N.
- Mass of the pendulum bob, m=2kg
- Length of the string, l (not specified, but it will cancel out later)
- Angle of displacement from the vertical, θ=90∘
- When the bob is at the lowest point of its swing, the forces acting on it are:
- The gravitational force acting downward, Fg=mg
- The tension in the string, T, acting upward.
- At the lowest point, the bob is moving in a circular path, so it requires a centripetal force, Fc, given by:
Fc=mv2l
- Here, v is the speed of the bob at the lowest point.
- At the lowest point, the net force acting on the bob is the difference between the tension and the gravitational force:
T−mg=Fc
- Rearranging gives:
T=mg+mv2l
- The potential energy lost by the bob when it swings down from the height h (which is equal to the length of the string l when displaced by 90∘) is converted into kinetic energy:
mgh=12mv2
- Since h=l, we can write:
mgl=12mv2
- Solving for v2:
v2=2gl
- Substitute v2 into the tension equation:
T=mg+m(2gl)l
- This simplifies to:
T=mg+2mg=3mg
- Now substituting m=2kg and g=10m/s2:
T=3×2×10=60N
The minimum tension that the string should be able to withstand is 60 N.
Topper's Solved these Questions
Similar Questions
A particle of mass m is suspended from a fixed point O by a string length l.At t=0, it is displaced from its equilibrium position and released.If T is the tension in the string with time t, then the correct graph is
View SolutionA pendulum bob of mass m and length L is released from angle θ with the vertical. Find
(a) the speed of the bob at the bottom of the swing and
(b) tension in the string at that time
View Solution
Knowledge Check
The string of pendulum of length l is displaced through 90∘ from the vertical and released. Then the minimum strength of the string in order to withstand the tension, as the pendulum passes through the mean position is
AmgB3mgC5mgD6mgThe bob of a simple pendulum of length L, is displaced through 90∘, from its mean position and then released. What will be the tension in the string when the bob of mass m will be at its lowest position?
AmgB3 mgC2 mgD6 mgA pendulum bob on a 2 m string is displaced 60∘ from the vertical and then released. What is the speed of the bob as it passes through the lowest point in its path
A√2m/sB√9.8m/sC4.43m/sD1/√2m/s
A pendulum bob on a 2 m string is displaced 60∘ from the verticle and then released . What is the speed of the bob as it passes through the lowest point in its path?
View SolutionThe bob of a simple pendulum of length L, is displaced through 90∘, from its mean position and then released. What will be the tension in the string when the bob of mass m will be its lowest position ?
View SolutionA bob hangs from a rigid support by an inextensible string of length l. If it is displaced through a distance l (from the lowest position) keeping the string straight and then released. The speed of the bob at the lowest position is
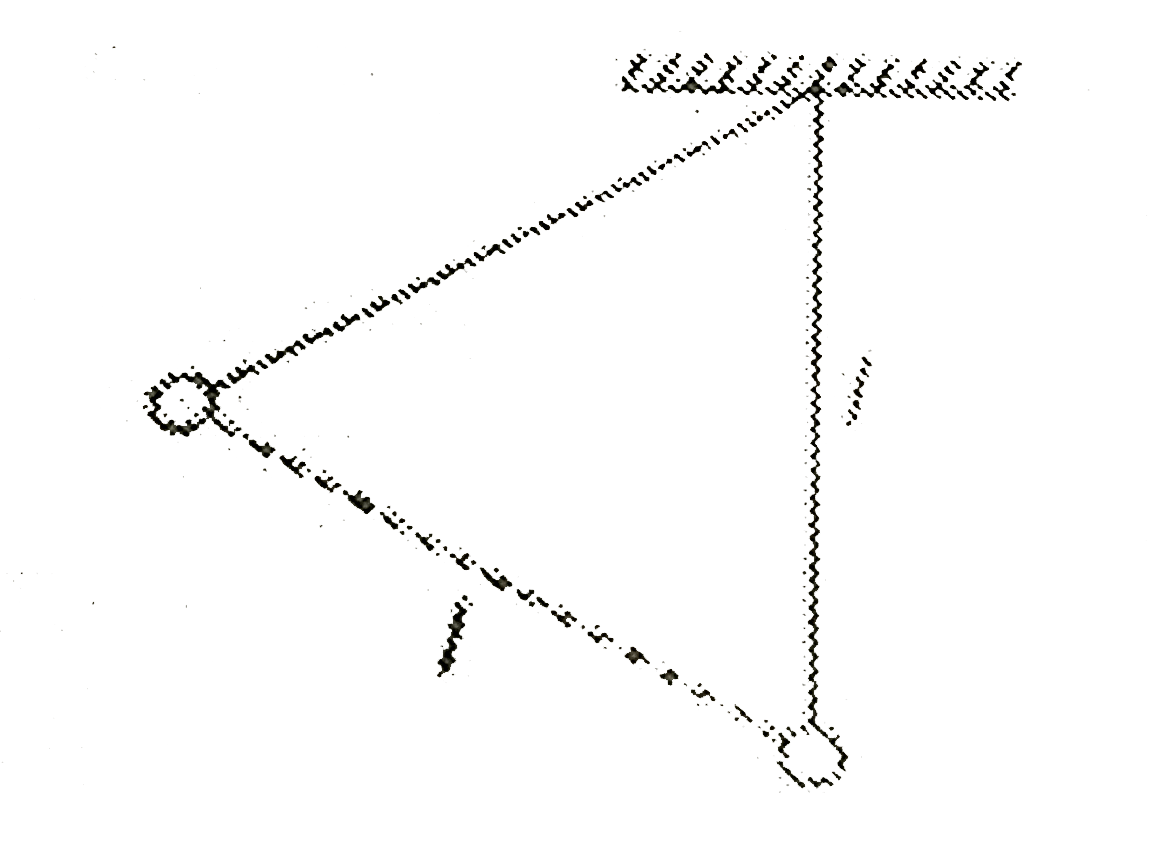
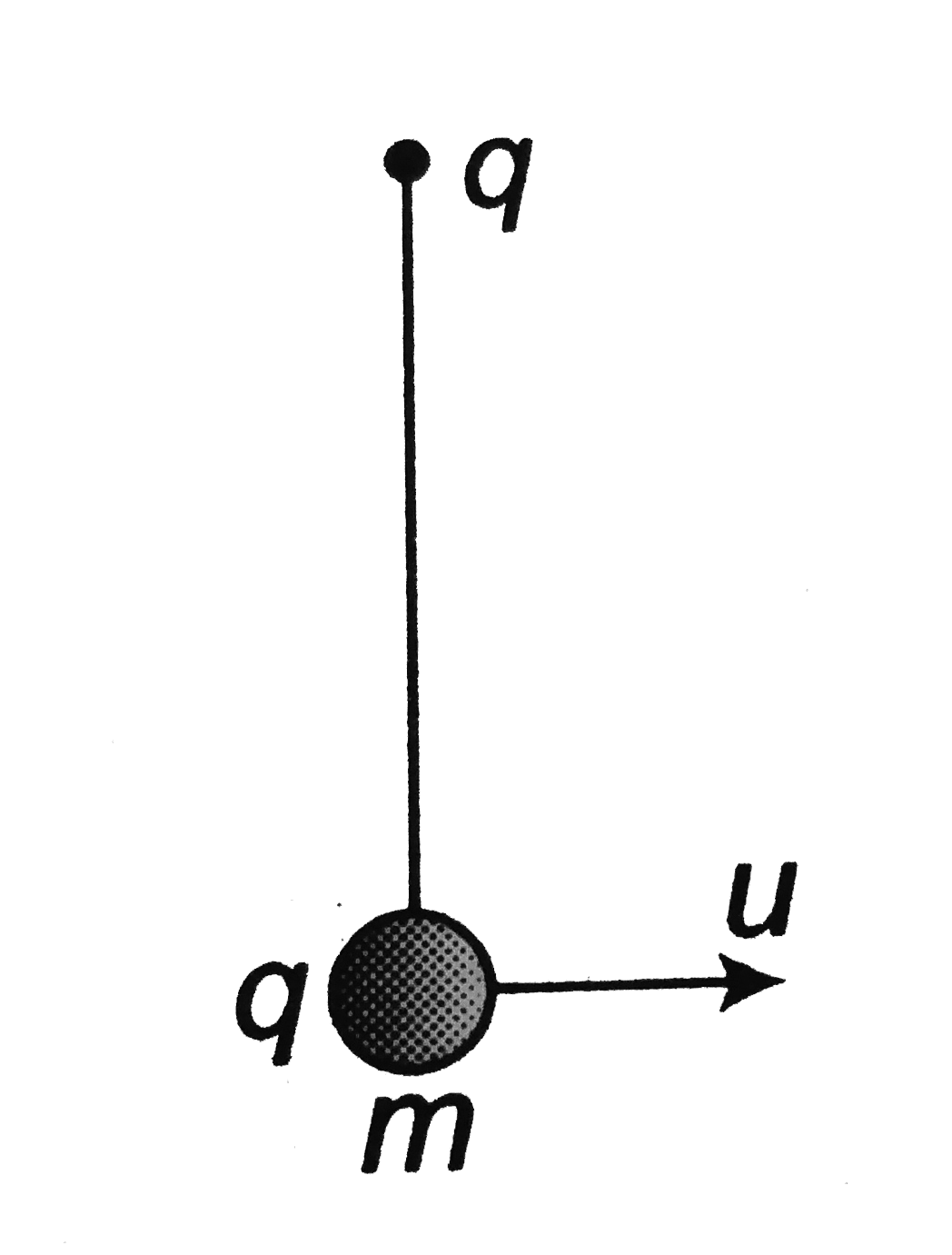
View SolutionThe bob of a pendulum has mass m=1kg and charge q=40μC. Length of the pendulum is l=0.9m. The point of suspension also has the same charge 40μC. What the minimum speed u should be imparted to the bob so that it can complete verticel circel ?
View SolutionA particle of mass m is suspended from a fixed O by a string of length L. At t=0, it is displaced from its equilibrium position and released. The graph which shows the variation of the tension T in the string with time t is :
View Solution
