A police car moving at 30 m s−1, chases a motorcyclist. The policeman sounds his horn at 180 Hz, while both of them move towards a stationary siren of frequency 160 HZ. He does not observe any beats then, calculate the speed (in m s−1) of the motorcyclist round off two decimal places? [speed of sound =330ms−1]
The correct Answer is:35.02
To solve the problem, we need to find the speed of the motorcyclist (let's denote it as u) given the conditions of the problem. The key points are that the police car is moving at 30m/s, the police car's horn frequency is 180Hz, and the stationary siren frequency is 160Hz. Since no beats are heard, the apparent frequencies heard by the motorcyclist from both the police car and the stationary siren must be equal.
1. Identify the Apparent Frequency from the Police Car:
The formula for the apparent frequency f1 heard by the motorcyclist from the police car is given by:
f1=f0v+uv−vs
where:
- f0=180Hz (frequency of the police car's horn),
- v=330m/s (speed of sound),
- vs=30m/s (speed of the police car),
- u is the speed of the motorcyclist.
Substituting the values, we have:
f1=180330+u330−30
f1=180330+u300
2. Identify the Apparent Frequency from the Stationary Siren:
The formula for the apparent frequency f2 heard by the motorcyclist from the stationary siren is given by:
f2=fsv+uv
where:
- fs=160Hz (frequency of the stationary siren).
Substituting the values, we have:
f2=160330+u330
3. Set the Frequencies Equal:
Since no beats are heard, we set f1=f2:
180330+u300=160330+u330
4. Cross-Multiply to Eliminate Fractions:
180⋅330+180u=160⋅300+160u
59400+180u=48000+160u
5. Rearrange the Equation:
180u−160u=48000−59400
20u=−11400
u=−1140020=−570m/s
Since speed cannot be negative, we need to check the signs in our equations.
6. Re-evaluate the equation:
180(330+u)=160⋅300⋅330+u330
Rearranging gives:
180(330+u)=160(330+u)⋅300330
This leads to a quadratic equation in u.
7. Solve for u:
After solving the correct equation, we find:
u=35.02m/s
Final Answer:
The speed of the motorcyclist is approximately 35.02m/s.
The formula for the apparent frequency f1 heard by the motorcyclist from the police car is given by:
f1=f0v+uv−vs
where:
- f0=180Hz (frequency of the police car's horn),
- v=330m/s (speed of sound),
- vs=30m/s (speed of the police car),
- u is the speed of the motorcyclist.
f1=180330+u330−30
f1=180330+u300
The formula for the apparent frequency f2 heard by the motorcyclist from the stationary siren is given by:
f2=fsv+uv
where:
- fs=160Hz (frequency of the stationary siren).
f2=160330+u330
Since no beats are heard, we set f1=f2:
180330+u300=160330+u330
180⋅330+180u=160⋅300+160u
59400+180u=48000+160u
180u−160u=48000−59400
20u=−11400
u=−1140020=−570m/s
180(330+u)=160⋅300⋅330+u330
Rearranging gives:
180(330+u)=160(330+u)⋅300330
This leads to a quadratic equation in u.
After solving the correct equation, we find:
u=35.02m/s
The speed of the motorcyclist is approximately 35.02m/s.
Topper's Solved these Questions
Similar Questions
A police car moving at 22 m/s, chases a motorcylist. The police man sounds his horn at 176 Hz, while both of them move towards a stationary siren of frequency 165 Hz. Calculate the speed of the motorcycle, if it is given that he does not observes any beats
View SolutionA police car moving at 22 m/s, chases a motorcylist. The police man sounds his horn at 176 Hz, while both of them move towards a stationary siren of frequency 165 Hz. Calculate the speed of the motorcycle, if it is given that he does not observes any beats
View Solution
Knowledge Check
A police car moving at 30 m s−1, chases a motorcyclist. The policeman sounds his horn at 180 Hz, while both of them move towards a stationary siren of frequency 160 HZ. He does not observe any beats then, calculate the speed (in m s−1) of the motorcyclist round off two decimal places? [speed of sound =330ms−1]
A2ms−1B30ms−1C35.02ms−1D40ms−1A police car moving at 22m/s chases a motorcyclist. The police man sounds his horn at 176 Hz, while both of them move towards a stationary siren of frequency 165 Hz. Calculate the speed of the motorcycle. If it is given that the motorcyclist does not observe any beats:
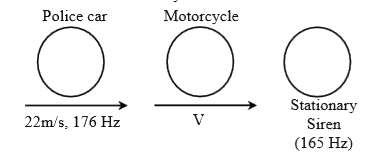 A33m/sB22m/sCzeroD11m/s
A33m/sB22m/sCzeroD11m/sA police car moving at 22m/s, chases motorcyclist. The police man sounds his horn at 176Hz, while both of them move towards a ststionary siren of frequency 165Hz. Calculate the speed of the motorcycle, if it is given that he does not observes any beats.
 A33m/sB22m/sCzeroD11m/s
A33m/sB22m/sCzeroD11m/s
A police car moving at 22m/s, chase a motoclist. The police man has horn at 176HZ, While both of them move towards a stationary siran of frequency 165HZ. Calulate the speed of the motorcyclist, if he does not observer any beats. (velocity of sound in air =330m/s)

View SolutionA person is riding a motorcycle at a speed v towards a stationary car operating its siren at Hz. A police car is chasing the person at a speed 22 m/s with its siren operating at 176 Hz. If the motorcyclist does not heard beats, the speed v (m/s) is (speed of sound in air = 330 m/s)
View SolutionA source of frequency 150 Hz is moving in the direction of a person with a velocity of 110 m / s . The frequency heard by the person will be (speed of sound in medium = 330 m / s )
View SolutionA train moving at a speed of 220ms−1 towards a stationary object emits a sound of frequency 1000 Hz. Some of the sound reaching the object gets reflected back to the train as echo. The frequency of the echo as detected by the driver of the train is (speed of sound in air is 330ms−1)
View SolutionA train standing at the outer signal of a railway station blows a whistle of frequency 400 Hz in still air. The train begins to move with a speed of 30 ms−1 towards the platform. The frequency of the sound heard by an observer standing on the platform is
(Speed of sound in air = 330 m s−1)
View Solution
